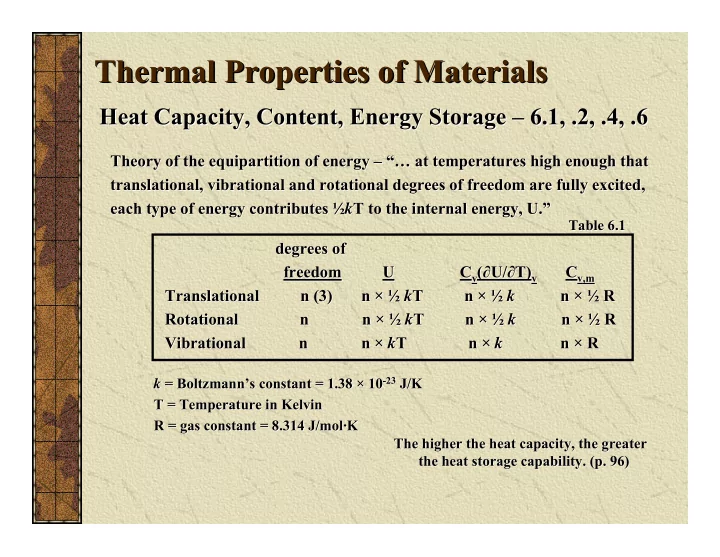

Thermal Properties of Materials Thermal Properties of Materials Heat Capacity, Content, Energy Storage – – 6.1, .2, .4, .6 6.1, .2, .4, .6 Heat Capacity, Content, Energy Storage Theory of the equipartition equipartition of energy of energy – – “… at temperatures high enough that “… at temperatures high enough that Theory of the translational, , vibrational vibrational and rotational degrees of freedom are fully excited, and rotational degrees of freedom are fully excited, translational each type of energy contributes ½ k T to the internal energy, U.” to the internal energy, U.” each type of energy contributes ½ k T Table 6.1 Table 6.1 degrees of degrees of freedom U C v ( ∂ ∂ U/ U/ ∂ ∂ T) T) v C v freedom U C v ( C v v,m ,m Translational n (3) n n (3) n × × ½ ½ k T n n × × ½ ½ k n × × ½ ½ R R Translational k T n k Rotational n n × × ½ ½ k T n n × × ½ ½ k n × × ½ ½ R R Rotational n n k T n k Vibrational n n n n × × k T n n × × k n × × R R Vibrational k T n k 23 J/K k = = Boltzmann’s Boltzmann’s constant = 1.38 constant = 1.38 × × 10 10 - -23 J/K k T = Temperature in Kelvin T = Temperature in Kelvin R = gas constant = 8.314 J/mol· R = gas constant = 8.314 J/mol ·K K The higher the heat capacity, the greater The higher the heat capacity, the greater the heat storage capability. (p. 96) the heat storage capability. (p. 96)
Thermal Properties of Materials Thermal Properties of Materials Degrees of Freedom, Heat Capacity Degrees of Freedom, Heat Capacity An N- -atom atom polyatomic polyatomic molecule has 3N degrees of freedom. These are molecule has 3N degrees of freedom. These are An N divided into translational translational, rotational and , rotational and vibrational vibrational components. components. divided into H 2 O 3N = 9 H 2 O 3N = 9 dof C v dof C Linear Nonlinear Linear Nonlinear v,m ,m Translational = 3 = 3 3/2R Translational 3/2R Translational 3 3 3 Translational 3 Rotational = 3 3/2R Rotational = 3 3/2R Rotational 2 3 Rotational 2 3 Vibrational = 3 = 3 3R Vibrational 3R Vibrational 3N 3N- -5 3N 5 3N- -6 6 Vibrational C v = 6R C ,m = 6R v,m C v (exp) = 3.038R C ,m (exp) = 3.038R v,m Why? Why? Translation and rotational energies are most easily excited (3R), , Translation and rotational energies are most easily excited (3R) while vibrations of gaseous water molecules at room temperature are are while vibrations of gaseous water molecules at room temperature only slightly active (0.038R). only slightly active (0.038R).
Thermal Properties of Materials Thermal Properties of Materials Heat Capacities of Solids Heat Capacities of Solids 1819, Dulong Dulong and Petit found for nonmetallic solids at room and Petit found for nonmetallic solids at room 1819, temperature, C C v ~ 3R = 25 J/K· ·mol. ( mol. (Dulong Dulong- -Petit Law) Petit Law) temperature, ,m ~ 3R = 25 J/K v,m Each atom in a solid has 3 degrees of freedom, and these Each atom in a solid has 3 degrees of freedom, and these are vibrational vibrational (simple (simple monoatomic monoatomic solids). U = 3kT; solids). U = 3kT; C C v = 3R are ,m = 3R v,m Not explained is why (experimentally) C C v � 0 as T 0 as T � � 0K ? 0K ? Not explained is why (experimentally) ,m � v,m
Thermal Properties of Materials Thermal Properties of Materials Heat Capacities of Solids Heat Capacities of Solids Einstein model of the heat capacity of a solid – – The thermal depopulation The thermal depopulation Einstein model of the heat capacity of a solid of vibrational vibrational energy levels is used to explain why energy levels is used to explain why C C v � 0 as T 0 as T � � 0K. 0K. of ,m � v,m (but predicts values too low at low temperatures) (but predicts values too low at low temperatures) Debye model of the heat capacity of a solid model of the heat capacity of a solid – – Considers the atoms in a Considers the atoms in a Debye solid to be vibrating with a distribution of frequencies, up to ν ν D . solid to be vibrating with a distribution of frequencies, up to D . θ D = h ν D / k k - - approximates strength of approximates strength of interatomic interatomic interactions interactions θ D = h ν D / - materials with higher materials with higher θ θ D are harder to deform - D are harder to deform θ D (K) θ D (K) Diamond 2230 Diamond 2230 Gold 225 Gold 225 Neon 75 Neon 75 Mercury (solid) 72 Mercury (solid) 72
Thermal Properties of Materials Thermal Properties of Materials Heat Capacities of Metals Heat Capacities of Metals For metals, there is the additional contribution of ‘free’ For metals, there is the additional contribution of ‘free’ conducting electrons (above Ef Ef). ). conducting electrons (above If each atom had one free valence electron, the total internal energy nergy If each atom had one free valence electron, the total internal e would be: U = 3 kT kT + 3/2 + 3/2 kT kT = 4.5 = 4.5 kT kT and and C C v = 4.5R would be: U = 3 ,m = 4.5R v,m (The first term is from vibrations alone, the second term is the (The first term is from vibrations alone, the second term is the translation of the free electron) translation of the free electron) The experimentally observed molar heat capacity of a monoatomic monoatomic The experimentally observed molar heat capacity of a metal is slightly over 3R. metal is slightly over 3R. Only a small fraction of the valence Only a small fraction of the valence electrons must be free to electrons must be free to translate. (one of the first translate. (one of the first supporting facts for Fermi Fermi supporting facts for statistics) statistics)
In- -Class Problem Set Class Problem Set In Ch. 6, #11) A new product claims to protect seedlings from freezing by ing by Ch. 6, #11) A new product claims to protect seedlings from freez surrounding them with vertical cylinders of water. a) How does this this surrounding them with vertical cylinders of water. a) How does device work? (explain in thermodynamic terms), b) Would this device work? (explain in thermodynamic terms), b) Would this device be as effective filled with another liquid, like oil? Explain. lain. device be as effective filled with another liquid, like oil? Exp Ch. 6, #22) The sound velocity varies considerably with depth in the the Ch. 6, #22) The sound velocity varies considerably with depth in earth’s crust, 8km/s in the upper mantle, and 12km/s in the lower r earth’s crust, 8km/s in the upper mantle, and 12km/s in the lowe mantle. a) How does θ θ D vary with depth? b) What does the mantle. a) How does D vary with depth? b) What does the variation of the sound velocity indicate about the interactions within within variation of the sound velocity indicate about the interactions the crustal crustal materials as a function of depth? c) Suggest an materials as a function of depth? c) Suggest an the application of the variation of sound velocity with depth in the application of the variation of sound velocity with depth in the earth’ ’s crust. s crust. earth For next time: Read Ch. 7 (pp. 128- -140) 140) For next time: Read Ch. 7 (pp. 128
Thermal Properties of Materials Thermal Properties of Materials Thermal Expansion of Solids – – 7.1, .3 (133 7.1, .3 (133- -140) 140) Thermal Expansion of Solids “Almost all materials expand when heated, regardless of the phase of matter” e of matter” “Almost all materials expand when heated, regardless of the phas � Related directly to the forces between atoms Related directly to the forces between atoms � V(x) V(x) Harmonic Harmonic potential potential x x st approximation, atoms in a solid To a 1 st approximation, atoms in a solid To a 1 V(x) = cx 2 2 ; c = spring stiffness, ; c = spring stiffness, V(x) = cx can be considered connected to each can be considered connected to each x = displaced distance x = displaced distance other by ‘springs’ other by ‘springs’ *The average value of x is *The average value of x is independent of temperature independent of temperature
Recommend
More recommend