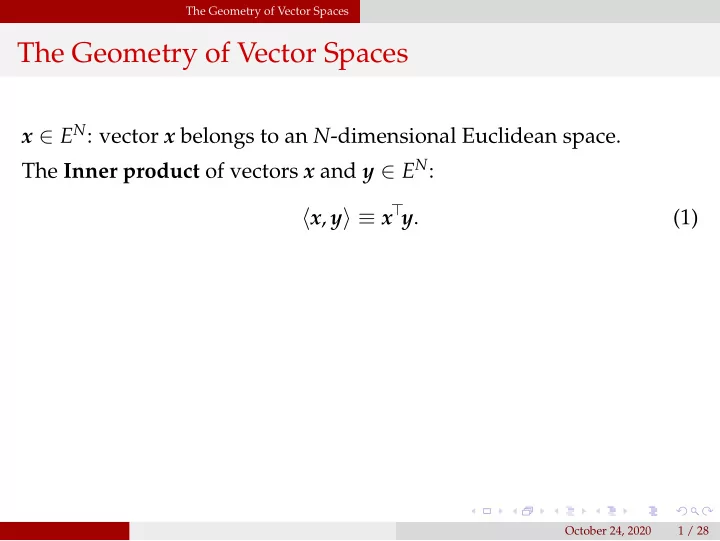
The Geometry of Vector Spaces x E N : vector x belongs to an N - PowerPoint PPT Presentation

The Geometry of Vector Spaces The Geometry of Vector Spaces x ∈ E N : vector x belongs to an N -dimensional Euclidean space. The Inner product of vectors x and y ∈ E N : � x , y � ≡ x ⊤ y . (1) October 24, 2020 1 / 28

The Geometry of Vector Spaces The Geometry of Vector Spaces x ∈ E N : vector x belongs to an N -dimensional Euclidean space. The Inner product of vectors x and y ∈ E N : � x , y � ≡ x ⊤ y . (1) The length or norm of a vector x in E N is � N 1/2 � � x � ≡ ( x ⊤ x ) 1/2 = ∑ x 2 . (2) i i = 1 October 24, 2020 1 / 28

The Geometry of Vector Spaces The Geometry of Vector Spaces x ∈ E N : vector x belongs to an N -dimensional Euclidean space. The Inner product of vectors x and y ∈ E N : � x , y � ≡ x ⊤ y . (1) The length or norm of a vector x in E N is � N 1/2 � � x � ≡ ( x ⊤ x ) 1/2 = ∑ x 2 . (2) i i = 1 A vector x in E 2 has coordinates x 1 and x 2 . Pythagoras’ Theorem tells us that the length of the vector x , the hypotenuse of a right-angled triangle, is ( x 2 1 + x 2 2 ) 1/2 . October 24, 2020 1 / 28