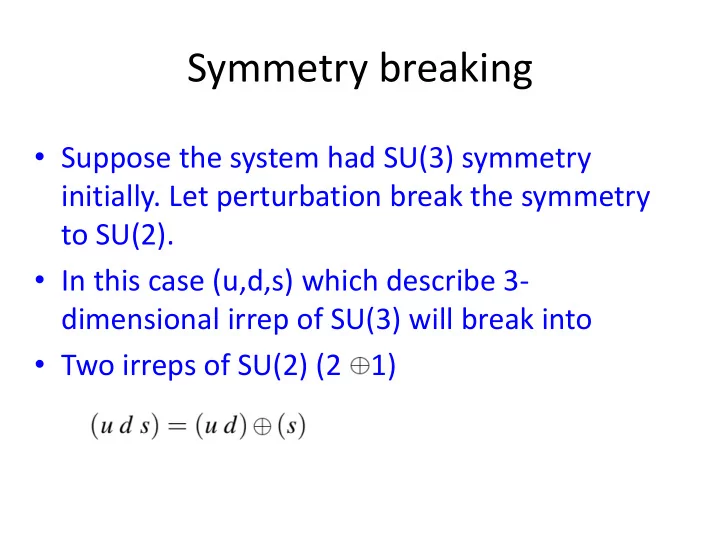
Symmetry breaking Suppose the system had SU(3) symmetry initially. - PowerPoint PPT Presentation

Symmetry breaking • Suppose the system had SU(3) symmetry initially. Let perturbation break the symmetry to SU(2). • In this case (u,d,s) which describe 3- dimensional irrep of SU(3) will break into • Two irreps of SU(2) (2 1)

Dynamical Symmetry • Suppose symmetry of the system has no geometrical interpretation , we say that the symmetry is dynamical symmetry • Recall Kepler laws of planetary motion/charged particle in a Coulombic potential • Hamiltonian commutes with angular momentum. This system has rotational and time translation symmetry- L and E are conserved • Runge-Lenz vector M is another conserved quantity

Dynamical Symmetry (contd) • Classically M satisfies the following constraints: • Quantum mechanical operator M is • Classical relations modifies to

Hydrogen atom- Lie algebra • The conserved quantities which commutes with Hamiltonian are L and M. Lie algebra involving them: • Rescale so that we obtain so(4) algebra- determine `a’

Hydrogen atom- Lie algebra • The rescale factor `a’ in will be • The last equation acting on a state with energy E will resemble so(4) if

Hydrogen atom- so(4) algebra • The generators are • Hence we add a fourth fictious coordinate ω so that the rotation in 4-dimensional space is given by • Recall the energy levels are E n = -13.6/n 2 eV. • Can we exploit SO(4) symmetry to obtain this energy?

SO(4)= SU(2) xSU(2) • We can rewrite so(4) generators such that the algebra resembles product of two su(2) algebras • That is., • Clearly, • Hence we can write states which are simultaneous eigenstates of H, I z , K z

SO(4)= SU(2) xSU(2) • The simultaneous eigenstate is denoted as • We can construct two Casimir operators which commutes with all so(4) generatos and H: • Using the form of M and L, you can check that C 2 is a null operator • This implies that i and k are constrained to be same • Hence where

SO(4)= SU(2) xSU(2) • From the two equations: • E is determined by equating • The exact expression is

SO(4)= SU(2) xSU(2) • Replace (2i+1) = n in the energy eigenvalues • The eigenvalues are the familiar hydrogen atom energy levels: • We know that the energy levels are n 2 fold degenerate ignoring spin quantum number • We can see this from the su(2) X su(2) algebra

Physical orbital angular momentum L • Note that the orbital angular momentum L is given by addition of the two su(2) generators : • The eigenstates of L z • Where the range of will be from • With the condition i=k , • in terms of n=2i+1, we get = 0,1,2 ,…n -1 giving degeneracy