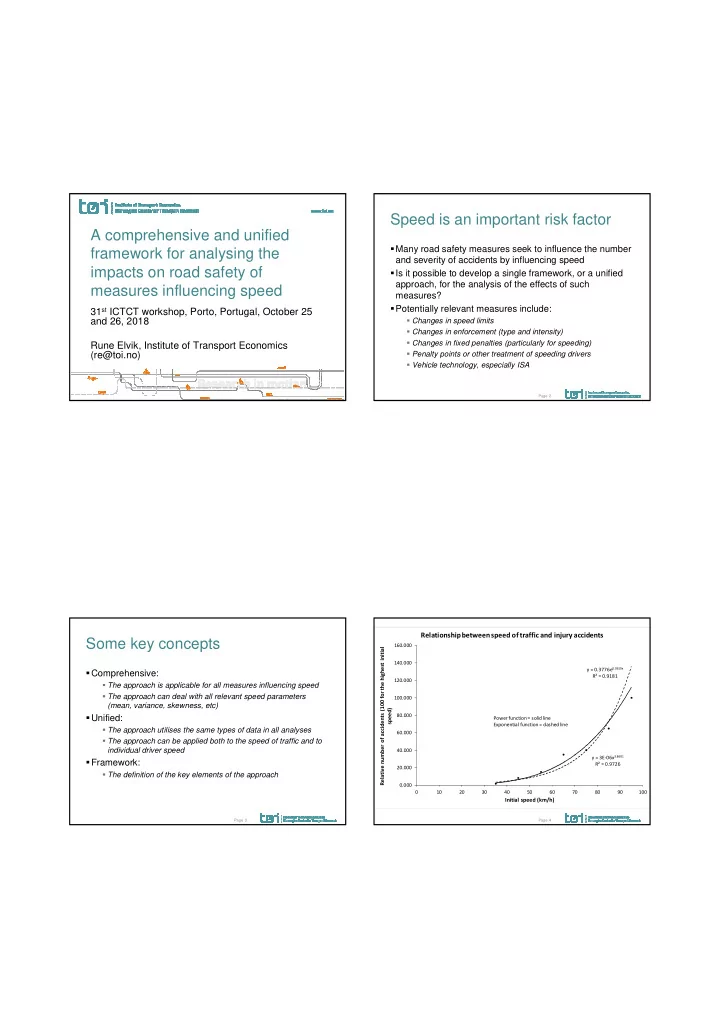
Speed is an important risk factor A comprehensive and unified Many - PDF document

Speed is an important risk factor A comprehensive and unified � Many road safety measures seek to influence the number framework for analysing the and severity of accidents by influencing speed impacts on road safety of � Is it possible to develop a single framework, or a unified approach, for the analysis of the effects of such measures influencing speed measures? � Potentially relevant measures include: 31 st ICTCT workshop, Porto, Portugal, October 25 � Changes in speed limits and 26, 2018 � Changes in enforcement (type and intensity) � Changes in fixed penalties (particularly for speeding) Rune Elvik, Institute of Transport Economics � Penalty points or other treatment of speeding drivers (re@toi.no) � Vehicle technology, especially ISA Page 2 Relationship between speed of traffic and injury accidents Some key concepts 160.000 Relative number of accidents (100 for the highest initial 140.000 � Comprehensive: y = 0.3776e 0.0619x R² = 0.9181 120.000 � The approach is applicable for all measures influencing speed � The approach can deal with all relevant speed parameters 100.000 (mean, variance, skewness, etc) speed) � Unified: 80.000 Power function = solid line Exponential function = dashed line � The approach utilises the same types of data in all analyses 60.000 � The approach can be applied both to the speed of traffic and to individual driver speed 40.000 y = 3E-06x 3.8601 � Framework: R² = 0.9726 20.000 � The definition of the key elements of the approach 0.000 0 10 20 30 40 50 60 70 80 90 100 Initial speed (km/h) Page 3 Page 4

Relationship between a driver's speed and probability of accident Actual speed distribution of control drivers (Kloeden et al. 1997) involvement (Kloeden et al. 1997) compared to normal distribution 1.00 250 y = 0.0032e 0.0678x R² = 0.776 0.90 205 200 0.80 168 0.70 Number of drivers Probability of accident 148 150 0.60 133 127 115 0.50 y = 3E-08x 3.8814 100 R² = 0.783 79 0.40 57 0.30 48 50 34 30 25 0.20 12 6 4 5 5 2 2 1 1 0 0.10 0 35 40 45 50 55 60 65 70 75 80 85 0.00 Speed (km/h) 0 10 20 30 40 50 60 70 80 90 Speed (kilometres per hour) Actual Normal Page 5 Page 6 The framework (case 80 km/h) Data needed � The mean speed of traffic Share of Mean Relative Relative Relative Interval traffic speed fatality rate serious injury rate slight injury rate � Either standard deviation or some statistic from which 3 to 2.5 below 0.6 56.3 0.21 0.30 0.45 standard deviation can be estimated, like 85th fractile of 2.5 to 2 below 1.7 59.9 0.27 0.38 0.52 speed distribution 2 to 1.5 below 4.4 63.5 0.36 0.47 0.60 � Estimates of the relationship between speed and risk of 1.5 to 1 below 9.2 67.1 0.49 0.58 0.70 1 to 0.5 below 15.0 70.7 0.65 0.72 0.81 injury 0.5 to 0 below 19.1 74.3 0.87 0.90 0.93 � In the example, the exponential model was applied: 0 to 0.5 above 19.1 77.9 1.15 1.11 1.07 � Coefficient 0.08 for fatal injury 0.5 to 1 above 15.0 81.5 1.54 1.38 1.24 � Coefficient 0.06 for serious injury 1 to 1.5 above 9.2 85.1 2.05 1.72 1.43 � Coefficient 0.04 for slight injury 1.5 to 2 above 4.4 88.7 2.74 2.13 1.66 2 to 2.5 above 1.7 92.3 3.65 2.64 1.91 2.5 to 3 above 0.6 95.9 4.87 3.28 2.21 Page 7 Page 8

Divide and conquer A very flexible framework � Assume that speed has a normal distribution � The framework can handle the following types of changes: � Divide the distribution into twelve intervals, each spanning � A reduction in speed across the whole distribution one half standard deviation � A larger reduction of the highest speeds than the lowest � Estimate the share of traffic in each interval � A reduction of speed variance � A truncation of the speed distribution at the speed limit (effect of � Estimate mean speed in each interval ISA) � Set relative risk equal to 1 at the mean speed � A dose-response curve for police enforcement � Compute relative risk in each interval, relying on the � The deterrent effect of increased fixed penalties exponential model � Examples of how to use the framework follow � The risk in each interval is the risk to drivers driving at the speeds comprised by that interval Page 9 Page 10 Speed distributions for speed limits 80 and 70 km/h in Norway An example 1 0.9 Share of Old New Change in Old relative New relative Interval traffic speed speed fatality rate fatality rate fatality rate 0.8 3 to 2.5 below 0.6 56.3 53.5 0.80 0.21 0.16 0.7 Cumulative distribution 2.5 to 2 below 1.7 59.9 56.2 0.74 0.27 0.20 2 to 1.5 below 4.4 63.5 58.9 0.69 0.36 0.25 0.6 1.5 to 1 below 9.2 67.1 61.6 0.64 0.49 0.31 0.5 68.3 76.1 1 to 0.5 below 15.0 70.7 64.3 0.60 0.65 0.39 0.4 0.5 to 0 below 19.1 74.3 67.0 0.56 0.87 0.48 0 to 0.5 above 19.1 77.9 69.7 0.52 1.15 0.60 0.3 0.5 to 1 above 15.0 81.5 72.4 0.48 1.54 0.74 0.2 1 to 1.5 above 9.2 85.1 75.1 0.45 2.05 0.92 1.5 to 2 above 4.4 88.7 77.8 0.42 2.74 1.14 0.1 2 to 2.5 above 1.7 92.3 80.5 0.39 3.65 1.41 0 2.5 to 3 above 0.6 95.9 83.2 0.36 4.87 1.76 0 20 40 60 80 100 120 Weighted sum 1.18 0.59 Speed (km/h) Page 11 Page 12

Driver adaptation to changes in police enforcement (model Application to speed model estimated) 1.200 Change in rate of speeding (1.00 = no change; 0.80 = 20 % Share of Contribution Compliance Revised 1.106 1.037 Interval traffic Speed to fatalities modification contribution 1.000 0.967 1.000 3 to 2.5 below 0.6 56.3 0.001 1.000 0.001 0.925 0.868 reduction; 1.20 = 20 % increase) 2.5 to 2 below 1.7 59.9 0.005 1.000 0.005 Current level of speeding 2 to 1.5 below 4.4 63.5 0.016 1.000 0.016 0.800 1.5 to 1 below 9.2 67.1 0.045 1.000 0.045 1 to 0.5 below 15.0 70.7 0.097 1.000 0.097 0.600 Current risk of apprehension 0.5 to 0 below 19.1 74.3 0.165 1.000 0.165 0 to 0.5 above 19.1 77.9 0.221 1.000 0.221 0.400 0.5 to 1 above 15.0 81.5 0.231 1.000 0.231 1 to 1.5 above 9.2 85.1 0.189 0.777 0.147 0.200 1.5 to 2 above 4.4 88.7 0.121 0.777 0.094 2 to 2.5 above 1.7 92.3 0.062 0.768 0.048 2.5 to 3 above 0.6 95.9 0.029 0.768 0.022 0.000 0.60 0.70 0.80 0.90 1.00 1.10 1.20 1.30 1.40 1.50 1.60 Weighted sum 1.182 1.092 Change in risk of apprehension (1.00 = no change; 0.80 = 20 % reduction; 1.20 = 20 % increase) Sum 1-3 above 0.401 0.311 Page 13 Page 14 Conclusions � To accurately estimate the effects on road safety of changes in speed, it is useful to model speed distributions � A default assumption is that speed follows a normal distribution, but the model can accommodate other distributions � The risk assumed at each level of the speed distribution should reflect the mean individual risk of drivers driving at that speed � Effects on individual driver risk and on the total number of accidents or injuries can then be integrated and made consistent Page 15