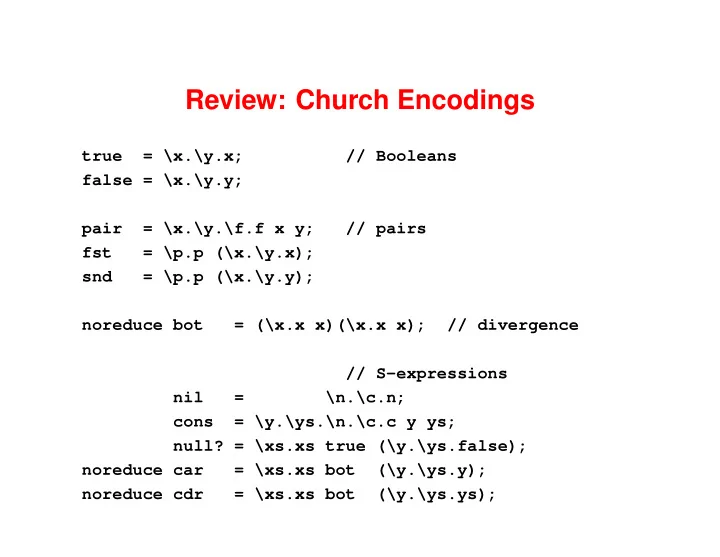
Review: Church Encodings true = \x.\y.x; // Booleans false = - PowerPoint PPT Presentation

Review: Church Encodings true = \x.\y.x; // Booleans false = \x.\y.y; pair = \x.\y.\f.f x y; // pairs fst = \p.p (\x.\y.x); snd = \p.p (\x.\y.y); noreduce bot = (\x.x x)(\x.x x); // divergence // S-expressions nil = \n.\c.n; cons = \y.\ys.\n.\c.c y ys; null? = \xs.xs true (\y.\ys.false); noreduce car = \xs.xs bot (\y.\ys.y); noreduce cdr = \xs.xs bot (\y.\ys.ys);

Review: Church Numerals zero = \f.\x.x; succ = \n.\f.\x.f (n f x); plus = \n.\m.n succ m; times = \n.\m.n (plus m) zero; ... -> four; \f.\x.f (f (f (f x))) -> three; \f.\x.f (f (f x)) -> times four three; \f.\x.f (f (f (f (f (f (f (f (f (f (f (f x)))))))))))

Reduction rules Central rule based on substitution (B ETA ) ( � x : M ) N ! M [ x 7! N � ℄ Structural rules: Beta-reduce anywhere, any time ! N ′ ! M ′ ! M ′ N M M � � � ! MN ′ ! M ′ N � x : M ′ MN MN � x : M � � � !

Free variables x is free in M _ x is free in N x is free in x x is free in MN 6 = x ′ x is free in M x � x ′ x is free in : M

Your turn! Free Variables What are the free variables in each expression? \x.\y. y z \x.x (\y.x) \x.\y.\x.x y \x.\y.x (\z.y w) y (\x.z) (\x.\y.x y) y

Your turn! Free Variables What are the free variables in each expression? \x.\y. y z - z \x.x (\y.x) - nothing \x.\y.\x.x y - nothing \x.\y.x (\z.y w) - w y (\x.z) - y z (\x.\y.x y) y - y

Capture-avoiding substitution x [ x 7! M M ℄ = y [ x 7! M y ℄ = ( YZ )[ x 7! M ( Y [ x 7! M ℄)( Z [ x 7! M ℄ = ℄) ( � x : Y )[ x 7! M � x : Y ℄ = ( � y : Z )[ x 7! M � y : Z [ x 7! M ℄ = ℄ if x not free in Z or y not free in M ( � y : Z )[ x 7! M � w : ( Z [ y 7! w ℄)[ x 7! M ℄ = ℄ where w not free in Z or M Last transformation is renaming of bound variables

Renaming of bound variables So important it has its own Greek letter: w not free in Z (A LPHA ) � y : Z � w : ( Z [ y 7! w ℄) � ! Also has structural rules

Conversion and reduction Alpha-conversion (rename bound variable) y not free in Z � x : Z � y : Z [ x 7! y � ! ℄ Beta-reduction (the serious evaluation rule) ( � x : M ) N ! M [ x 7! N � ℄ Eta-reduction: x not free in M � x : Mx ! M � All structural: Convert/reduce whole term or subterm

Church-Rosser Theorem Equivalence of convertible terms: if A ! B and A ! C ! ∗ D and C ! ∗ D there exists D s.t. B

Idea: normal form A term is a normal form if It cannot be reduced What do you suppose it means to say • A term has no normal form? • A term has a normal form?

Idea: normal form A term is a normal form if It cannot be reduced A term has a normal form if There exists a sequence of reductions that terminates (in a normal form) A term has no normal form if It always reduces forever (This term diverges)

Normal forms code for values Corollary of Church-Rosser: ! ∗ B , B in normal form, and if A ! ∗ C , C in normal form A then B and C are identical (up to renaming of bound variables)

Normal-order reduction (If a normal form exists, find it!) Application offers up to three choices: #1 (B ETA ) ( � x : M ) N ! M � [ x 7! N ℄ ! N ′ ! M ′ ! M ′ N M M � � � #3 #2 ! MN ′ ! M ′ N � x : M ′ MN MN � x : M � � � ! Slogan: “leftmost, outermost redex”

Normal-order illustration Not every term has a normal form: ( � x : xx )( � x : xx ) ( � x : xx )( � x : xx ) � ! But ( � x :� y : y )(( � x : xx )( � x : xx )) � y : y � ! Think “bodies before arguments” Applicative order does not terminate!

What solves this equation? Equation: � n : if n = 0 then 1 else n � 1 ) ? ( n fact � fact = The factorial function!

Review What solves this Equation? � n : if n = 0 then 1 else n � 1 ) ? ( n fact � fact = The factorial function!

Your turn: Recursion equations Is there a solution? Is it unique? If so, what is it? f1 = \n.\m.(eq? n m) n (plus n (f1 (succ n) m)); f2 = \n.f2 (isZero? n 100 (pred n)); f3 = \xs.xs nil (\z.\zs.cons 0 (f3 zs)); f4 = \xs.\ys.f4 ys xs;

Wait for it...

Your turn: Recursion equations f1 = \n.\m.(eq? n m) n (plus n (f1 (succ n) m)); ; sigma (sum from n to m) f2 = \n.f2 (isZero? n 100 (pred n)); ; no unique solution (any constant f2) f3 = \xs.xs nil (\z.\zs.cons 0 (f3 zs)); ; map (const 0) f4 = \xs.\ys. f4 xs ys; ; not unique: constant functions, commutative

Recursion = Fixed point Equation: � n : if n = 0 then 1 else n � 1 ) ? ( n fact � fact = Definition such that fact = g fact : :� n : if n = 0 then 1 else n g � f � f ( n � 1 ) =

Conversion to fixed point length = \xs.null? xs 0 (+ 1 (length (cdr xs))) lg = \lf.\xs.null? xs 0 (+ 1 (lf (cdr xs)))

One startling idea You can define a fixed-point operator • More than one!

A simple algebraic law If fix g = g (fix g) then fix g can define recursive functions! The only recursion equation you’ll ever need

Y combinator can implement fix Define Y such that, for any g , Y g ( Y g ) : = g Y � f : ( � x : f ( xx ))( � x : f ( xx )) = Y g ( � x : g ( xx ))( � x : g ( xx )) = and by beta-conversion Y g g (( � x : g ( xx ))( � x : g ( xx ))) = Y g g ( Y g ) = so Y g is a fixed point of g Does Y g have a normal form?