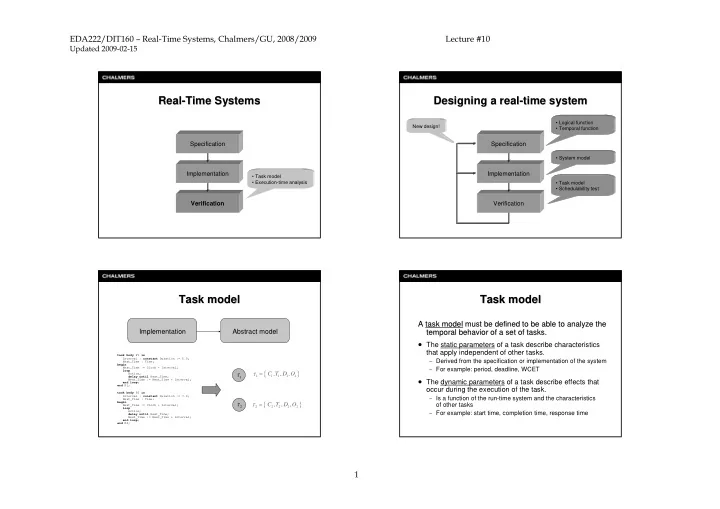

EDA222/DIT160 – Real-Time Systems, Chalmers/GU, 2008/2009 Lecture #10 Updated 2009-02-15 Real Real- -Time Systems Time Systems Designing a real- Designing a real -time system time system • Logical function What should be done & New design! • Temporal function When should it be done? Specification Specification • System model How should it be done? Implementation Implementation • Task model • Execution-time analysis • Task model Can it be done with the • Schedulability test given implementation? Verification Verification Task model Task model Task model Task model A task model A task model must be defined to be able to analyze the must be defined to be able to analyze the Implementation Implementation Abstract model Abstract model temporal behavior of a set of tasks. temporal behavior of a set of tasks. • The static parameters of a task describe characteristics that apply independent of other tasks. task body P1 is Interval : constant Duration := 5.0; – Derived from the specification or implementation of the system Next_Time : Time; begin Next_Time := Clock + Interval; – For example: period, deadline, WCET { } loop τ τ = C T D O , , , Action; delay until Next_Time; 1 1 1 1 1 1 • The dynamic parameters of a task describe effects that Next_Time := Next_Time + Interval; end loop ; end P1; occur during the execution of the task. task body P2 is Interval : constant Duration := 7.0; – Is a function of the run-time system and the characteristics Next_Time : Time; { } τ τ = begin C T D O , , , of other tasks Next_Time := Clock + Interval; 2 2 2 2 2 2 loop Action; – For example: start time, completion time, response time delay until Next_Time; Next_Time := Next_Time + Interval; end loop ; end P2; 1
EDA222/DIT160 – Real-Time Systems, Chalmers/GU, 2008/2009 Lecture #10 Updated 2009-02-15 Task model Task model Task model Task model Static task parameters: Static task parameters: Static task parameters: Static task parameters: (undisturbed) WCET C : i Task’s worst-case execution time (WCET) C T period : i i { } τ τ = – Represents the longest undisturbed execution time for one C T D O , , , D (relative) deadline : i i i i i i i iteration of the task O (absolute) time offset : i – Derived as a function of the task’s program code Task’s relative deadline (responsiveness constraint) D D i i – Represents the maximum allowed time within which the task must complete its execution – Applies relative to the time when the task becomes executable 0 t – Derived as a function of the environment (e.g., laws of nature, C i control theory, ...) O T i i Task model Task model Task model Task model Static task parameters: Different types of tasks: Different types of tasks: Static task parameters: • Periodic tasks T Task’s periodicity i – A periodic task arrives with a time interval T i – Represents how often the task should be repeated • Sporadic tasks – Each iteration of the task has the same WCET – A sporadic task arrives with a time interval ≥ T i O Task’s time offset i • Aperiodic tasks – Represents the first arrival time of the task, e.g., the earliest time instant at which the task becomes executable – An aperiodic task has no guaranteed minimum time between – Applies relative to a given ”origin” of the system two subsequent arrivals ⇒ Hard real ⇒ The arrival time of the n:th iteration of a task then becomes Hard real- -time systems can only contain periodic and time systems can only contain periodic and sporadic tasks. sporadic tasks. = + − ⋅ n A O ( n 1 ) T i i i 2
EDA222/DIT160 – Real-Time Systems, Chalmers/GU, 2008/2009 Lecture #10 Updated 2009-02-15 The importance of models The importance of models (this page deliberately left blank) (this page deliberately left blank) Dad? How do t hey know They dr ive bigger and Then t hey t ake t he Oh, I guess Honey, if you how much weight a br idge bigger t rucks over t he weight of t he last I should have don' t know t he can handle? bridge unt il it collapses! t ruck and r ebuild t he known t hat ! answer, j ust bridge SAY so! Free t ranslat ion f rom Swedish by J . J onsson Execution- -time analysis time analysis Execution- -time analysis time analysis Execution Execution Motivation: Motivation: • Worst-case execution time (WCET) is important since Real-time compiler Code Program – it is a prerequisite for (hard) schedulability analysis Compiler + (no input – resource needs should be estimated early in the design phase WCET data) WCET analysis • The execution time of a task depends on – program structure + input data – initial system state – temporal properties of the system (OS + hardware) for I:=1 to N loop begin – internal and external system events if A > K then A:=K-1; 42 42 Estimation of WCET should consequently be made else A:=K+1; if A < K while the program is compiled! then A:=K; else A:=K-1; end; 3
EDA222/DIT160 – Real-Time Systems, Chalmers/GU, 2008/2009 Lecture #10 Updated 2009-02-15 Execution- Execution -time analysis time analysis Execution- Execution -time analysis time analysis Execution time Requirements: Requirements: • WCET must be pessimistic but tight estimated WCET 0 ≤ ”Estimated WCET” – “Real WCET” < ε ( ε small compared to real WCET) real WCET pessimistic: pessimistic: to make sure assumptions made in the schedulability analysis of hard real-time tasks also apply at run time tight: tight: to avoid unnecessary waste of resources during scheduling of hard real-time tasks • The computational complexity of the analysis method Input data must be tractable A simple (yet challenging) example A simpler (but non- -trivial) example trivial) example A simple (yet challenging) example A simpler (but non Derive WCET for the following program: Derive WCET for the following program: Derive WCET for the following statement: Derive WCET for the following statement: Issues to consider: Issues to consider: Issues to consider: Issues to consider: A := A / B; for I:=1 to N loop • Input data is unknown • Execution time: begin – Iteration bounds must be known if A > K – affected by cache misses, pipeline conflicts, exceptions ... to facilitate analysis then A:=K-1; (T1) – depends on previous and (!) subsequent instructions else A:=K+1; (E1) • Path explosion – also depends on (unknown) input data if A < K – 4^N paths in this example • Observations: then A:=K; (T2) • Exclusion of non-executable (false) else A:=K-1; (E2) – accurate estimation of WCET must be based on a detailed end; paths timing model of the system architecture – T1 + E2 is a false path in the example – uncertainties are handled by making worst-case assumptions 4
EDA222/DIT160 – Real-Time Systems, Chalmers/GU, 2008/2009 Lecture #10 Updated 2009-02-15 Formulation of the WCET problem Formulation of the WCET problem Fundamental issues Fundamental issues • Issues in the analysis of program paths – how to limit WCET (if necessary, pessimistically) – how to eliminate false paths (in order to derive a tight Given a system WCET estimate) (= program structure + system platform) • Issues in the analysis of temporal behavior find the program’s “worst-case” execution time ”everything that takes time must be modeled in a realistic fashion (or at least not optimistically)” for all possible input data, initial system states – accurate and effective timing model of the system platform and (internal and external) system events (influence of, e.g., cache memories, pipelining, …) – consequences of system events at run time (e.g.: exceptions, interrupts, context switches) Path analysis Path analysis Path analysis Path analysis Shaw’ Shaw ’s Timing Schema (1989): s Timing Schema (1989): A control flow graph (CFG) describes The estimated WCET ( The estimated WCET (WCETe WCETe) is the ) is the the structure of the program execution time of the longest structural structural execution time of the longest for I:=1 to N loop path through the program path through the program begin Timing analysis problem: if A > K (I1) Find the longest executable path in then A:=K-1; (T1) WCETe = else A:=K+1; (E1) the program’s CFG N*(WCET(loop) + if A < K (I2) WCET(I1) + then A:=K; (T2) max(WCET(T1), WCET(E1)) + else A:=K-1; (E2) WCET(I2) + • CFG may not contain cycles end; max(WCET(T2), WCET(E2))) • Non-executable paths must be eliminated 5
Recommend
More recommend