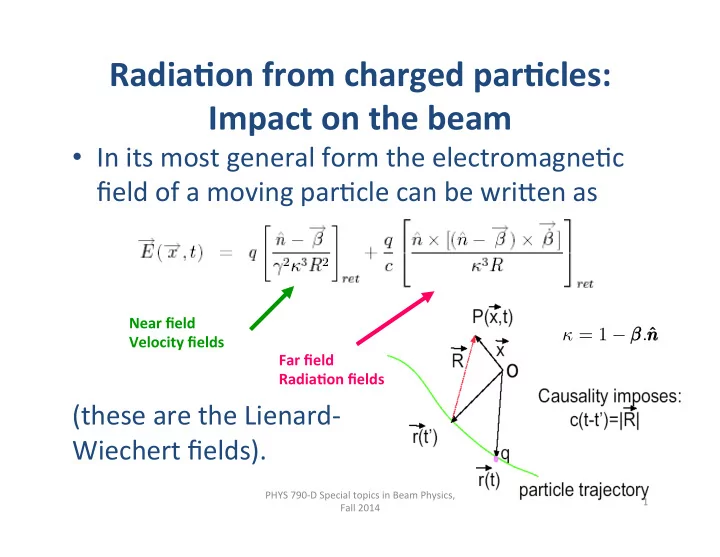
Radia%on from charged par%cles: Impact on the beam In - PowerPoint PPT Presentation

Radia%on ¡from ¡charged ¡par%cles: ¡ ¡ Impact ¡on ¡the ¡beam ¡ • In ¡its ¡most ¡general ¡form ¡the ¡electromagne1c ¡ field ¡of ¡a ¡moving ¡par1cle ¡can ¡be ¡wri8en ¡as ¡ ¡ ¡ ¡ ¡ Near ¡field ¡ ¡ ¡ κ = 1 − β β . ˆ β ˆ ˆ Velocity ¡fields ¡ n n n Far ¡field ¡ ¡ ¡ Radia%on ¡fields ¡ (these ¡are ¡the ¡Lienard-­‑ ¡ Wiechert ¡fields). ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 1 ¡ Fall ¡2014 ¡

Radia%on ¡from ¡a ¡bunch ¡of ¡par%cle ¡ • A ¡bunch ¡of ¡par1cle ¡comprises ¡ many ¡par1cles ¡ ¡ • how ¡does ¡this ¡affects ¡the ¡proper1es ¡of ¡the ¡emi8ed ¡ radia1on? ¡ • It ¡actually ¡depends ¡on ¡the ¡wavelength/frequency ¡of ¡ observa1on ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 2 ¡ Fall ¡2014 ¡

mul%par%cle ¡coherence ¡ P • let’s ¡compute ¡the ¡total ¡field ¡generated ¡by ¡an ¡ ensemble ¡of ¡ N ¡par1cles ¡as ¡detected ¡at ¡ P ¡ ct k N X E k ( P ) e − i ω t k E N ( P ) = O k =1 • assume ¡the ¡field ¡produced ¡by ¡each ¡par1cle ¡is ¡ iden1cal ¡ ¡ 2 d 2 W ∝ | E N ( P ) | 2 = d 2 W � � � � X e − i ω t k � � � � � � � � d ω d Ω d ω d Ω � � � � N 1 1 k • let’s ¡evaluate ¡the ¡mul1plica1ve ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 3 ¡ Fall ¡2014 ¡

mul%par%cle ¡coherence ¡ • we ¡have ¡ ¡ ! ! 2 � � X X X X e + i ω t k X � e � i ω t k � e + i ω t k e � i ω t k e � i ω t l = N + = � � � � k k k k l 6 = k • introducing ¡the ¡line-­‑charge ¡density ¡ ¡ Λ ( t ) Fourier ¡ ¡ � � transform ¡ X 2 � � � � = N + N ( N − 1) | e e − i ω t k Λ ( ω ) | 2 � � bunch ¡form ¡ k � � factor ¡(BFF) ¡ • or ¡ X 2 � � � � ' N + N 2 | e e − i ω t k Λ ( ω ) | 2 � � N � 1 k PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 4 ¡ Fall ¡2014 ¡

bunch ¡form ¡factor ¡ Gaussian ¡bunch ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡Modulated ¡Gaussian ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡bunch ¡ × N PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 5 ¡ Fall ¡2014 ¡

bunch ¡form ¡factor ¡(cnt’d) ¡ 10 13 100000 90000 10 12 80000 . ¡ 10 11 Population 70000 BFF (a.u.) 10 10 60000 10 9 50000 40000 10 8 30000 10 7 20000 10 6 10000 10 5 0 10 -5 10 -4 10 -3 10 -2 10 -1 10 0 10 1 10 2 -5.0 -2.5 0.0 2.5 5.0 10 13 100000 90000 10 12 80000 10 11 Population 70000 BFF (a.u.) 10 10 60000 10 9 50000 40000 10 8 30000 10 7 20000 10 6 10000 10 5 0 10 -5 10 -4 10 -3 10 -2 10 -1 10 0 10 1 10 2 -5.0 -2.5 0.0 2.5 5.0 10 13 100000 90000 10 12 80000 10 11 Population 70000 BFF (a.u.) 10 10 60000 10 9 50000 40000 10 8 30000 10 7 20000 10 6 10000 10 5 0 10 -5 10 -4 10 -3 10 -2 10 -1 10 0 10 1 10 2 -5.0 -2.5 0.0 2.5 5.0 z/ / PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ z z 6 ¡ Fall ¡2014 ¡

case ¡of ¡synchrotron ¡radia%on ¡and ¡ ¡ coherent ¡synchrotron ¡radia%on ¡(CSR) ¡ Beam ¡pipe ¡ • . ¡ induced ¡ ¡ (CSR) ¡enhancement ¡ frequency ¡ ¡ cut-­‑off ¡ SR ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 7 ¡ Fall ¡2014 ¡

Summary ¡of ¡mul%par%cle ¡coherence ¡ • a ¡bunch ¡of ¡par1cle ¡radiates ¡coherently ¡at ¡ wavelength ¡larger ¡than ¡the ¡typical ¡bunch ¡size ¡ • the ¡coherent ¡enhancement ¡goes ¡as ¡the ¡number ¡ of ¡par1cles ¡ N ¡in ¡the ¡bunch ¡(so ¡total ¡energy ¡scales ¡ as ¡ N 2 ) ¡ • therefore ¡to ¡enhance ¡the ¡genera1on ¡of ¡radia1on ¡ at ¡a ¡given ¡wavelength ¡(via ¡an ¡electromagne1c ¡ process) ¡one ¡needs ¡to ¡compress ¡(or ¡modulate) ¡ the ¡temporal ¡bunch ¡density ¡to ¡a ¡scale ¡below ¡the ¡ desired ¡wavelength ¡ ¡ • This ¡is ¡how ¡a ¡free-­‑electron ¡laser ¡(FEL) ¡works. ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 8 ¡ Fall ¡2014 ¡

Collec%ve ¡effects ¡ • electromagne1c ¡radia1on ¡ can ¡produce ¡a ¡“wake” ¡ • Given ¡the ¡field ¡produced ¡ ¡ by ¡a ¡one ¡par1cle ¡the ¡par1cles ¡ behind ¡experience ¡a ¡change ¡in ¡momentum ¡ Z + ∞ p ( x x, s ) = q dt [ E E ( x x, t ) + ˆ B ( x x, t )] ret δ p p x E x z B x z zB −∞ beamline ¡ coordinate ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 9 ¡ Fall ¡2014 ¡

case ¡of ¡energy ¡modula%on ¡ ¡ due ¡to ¡wakefield ¡ • the ¡change ¡in ¡energy ¡can ¡be ¡wri8en ¡as ¡ Z + ∞ � � δ δ E ( x x, s ) ' c δ δ δ p z ( x x, s ) = cq dtE z ( x x, t ) δ x x x � ¡ � ret −∞ • for ¡a ¡bunch ¡we ¡need ¡to ¡carry ¡the ¡convolu1on ¡ with ¡the ¡charge ¡distribu1on ¡ ¡ ¡ Nq Λ ( z = ct ) Z + 1 we ¡ignore ¡ δ E ( z, s = L ) ' Nq 2 L dz 0 Λ ( z 0 ) E ( z 0 � z ) dependence ¡ on ¡transverse ¡ ¡ z coordinate ¡ interac%on ¡ longitudinal ¡posi%on ¡ normalized ¡ length ¡ of ¡the ¡e-­‑ ¡within ¡the ¡ to ¡unity ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ bunch ¡ 10 ¡ Fall ¡2014 ¡

Example ¡of ¡wakefields ¡ • resis1ve ¡wake: ¡due ¡to ¡ ¡ finite ¡conduc1vity ¡of ¡ ¡ [from ¡A. ¡Chao, ¡SLAC] ¡ vacuum ¡pipe ¡ • geometric ¡wakes: ¡due ¡ to ¡change ¡in ¡beam ¡pipe ¡ ¡ [from ¡Phys. ¡Rev. ¡Le8. ¡108, ¡ ¡ geometry ¡ 034801 ¡(2012)] ¡ • dielectric, ¡surface ¡ ¡ roughness ¡wakefield ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 11 ¡ Fall ¡2014 ¡

Collec%ve ¡effects: ¡ ¡ self-­‑interac%on ¡via ¡CSR ¡ • Condi1on ¡for ¡a ¡bunch ¡interact ¡with ¡SR ¡it ¡ emi8ed ¡at ¡a ¡previous ¡1me: ¡ σ s b a a σ s ≥ R φ 3 b R ϕ 24 • This ¡is ¡the ¡regime ¡of ¡ coherent ¡ synchrotron ¡radia1on ¡for ¡most ¡prac1cal ¡cases ¡ • The ¡bending ¡path ¡length ¡should ¡be ¡larger ¡than ¡ the ¡overtaking ¡length ¡ ¡ L 0 = (24 σ s R 2 ) 1 / 3 PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 12 ¡ Fall ¡2014 ¡

self-­‑interac%on ¡via ¡CSR ¡ • The ¡self ¡interac1on ¡due ¡to ¡ ¡ bunch ¡trajectory ¡ CSR ¡is ¡actually ¡a ¡short-­‑ ¡ range ¡interac1on ¡ γ = 6 Green’s ¡func1on ¡ energy ¡loss ¡ energy ¡gain ¡ distance ¡behind ¡the ¡par1cle ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 13 ¡ Fall ¡2014 ¡

CSR ¡(cnt’d) ¡ • The ¡rate ¡of ¡energy ¡loss ¡of ¡an ¡electron ¡located ¡ at ¡z ¡within ¡the ¡bunch ¡is ¡ Z z head ¡ W ( z ) = 2 r e mc 2 1 Λ ( z 0 ) ( z − z 0 ) 4 / 3 dz 0 3 4 / 3 R 2 / 3 �1 tail ¡ • ager ¡integra1on ¡by ¡parts ¡ ¡ Z z W ( z ) = 2 r e mc 2 1 d Λ ( z 0 ) dz 0 3 1 / 3 R 2 / 3 ( z − z 0 ) 1 / 3 dz 0 �1 PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 14 ¡ Fall ¡2014 ¡

CSR ¡(cnt’d) ¡ • realis1c ¡simula1on ¡ trajectory ¡ emiNed ¡ ¡ radia%on ¡ pulse ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 15 ¡ Fall ¡2014 ¡