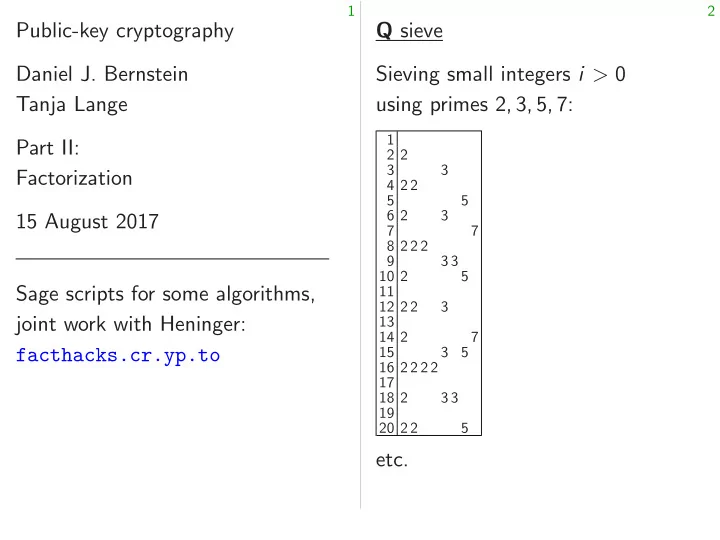

4 5 Why did this find a factor of 611? Why did the first three Was it just blind luck: completely factored congruences gcd { 611 ; random } = 47? have square product? Was it just blind luck? No. Yes. The exponent vectors By construction 611 divides s 2 − t 2 (1 ; 0 ; 4 ; 1) ; (6 ; 3 ; 2 ; 0) ; (1 ; 1 ; 2 ; 3) where s = 14 · 64 · 75 happened to have sum 0 mod 2. and t = 2 4 3 2 5 4 7 2 . So each prime > 7 dividing 611 But we didn’t need this luck! divides either s − t or s + t . Given long sequence of vectors, easily find nonempty subsequence Not terribly surprising with sum 0 mod 2. (but not guaranteed in advance!) that one prime divided s − t and the other divided s + t .
4 5 did this find a factor of 611? Why did the first three This is linea just blind luck: completely factored congruences Guaranteed 611 ; random } = 47? have square product? if number Was it just blind luck? exceeds length Yes. The exponent vectors e.g. for n construction 611 divides s 2 − t 2 (1 ; 0 ; 4 ; 1) ; (6 ; 3 ; 2 ; 0) ; (1 ; 1 ; 2 ; 3) 1( n + s = 14 · 64 · 75 happened to have sum 0 mod 2. 4( n + = 2 4 3 2 5 4 7 2 . 15( n + 15) each prime > 7 dividing 611 But we didn’t need this luck! 49( n + 49) either s − t or s + t . Given long sequence of vectors, 64( n + 64) easily find nonempty subsequence terribly surprising with sum 0 mod 2. F 2 -kernel not guaranteed in advance!) gen by (0 one prime divided s − t e.g., 1( n the other divided s + t . is a squa
4 5 find a factor of 611? Why did the first three This is linear algeb luck: completely factored congruences Guaranteed to find } = 47? have square product? if number of vecto Was it just blind luck? exceeds length of each Yes. The exponent vectors e.g. for n = 671: 611 divides s 2 − t 2 1( n + 1) = 2 5 3 1 (1 ; 0 ; 4 ; 1) ; (6 ; 3 ; 2 ; 0) ; (1 ; 1 ; 2 ; 3) 64 · 75 4( n + 4) = 2 2 3 3 happened to have sum 0 mod 2. 2 . 15( n + 15) = 2 1 3 1 7 dividing 611 But we didn’t need this luck! 49( n + 49) = 2 4 3 2 − t or s + t . Given long sequence of vectors, 64( n + 64) = 2 6 3 1 easily find nonempty subsequence rising with sum 0 mod 2. F 2 -kernel of exponent ranteed in advance!) gen by (0 1 0 1 1) divided s − t e.g., 1( n +1)15( n divided s + t . is a square.
4 5 of 611? Why did the first three This is linear algebra over F 2 completely factored congruences Guaranteed to find subsequence have square product? if number of vectors Was it just blind luck? exceeds length of each vecto Yes. The exponent vectors e.g. for n = 671: divides s 2 − t 2 1( n + 1) = 2 5 3 1 5 0 7 1 ; (1 ; 0 ; 4 ; 1) ; (6 ; 3 ; 2 ; 0) ; (1 ; 1 ; 2 ; 3) 4( n + 4) = 2 2 3 3 5 2 7 0 ; happened to have sum 0 mod 2. 15( n + 15) = 2 1 3 1 5 1 7 3 ; dividing 611 But we didn’t need this luck! 49( n + 49) = 2 4 3 2 5 1 7 2 ; t . Given long sequence of vectors, 64( n + 64) = 2 6 3 1 5 1 7 2 . easily find nonempty subsequence with sum 0 mod 2. F 2 -kernel of exponent matrix advance!) gen by (0 1 0 1 1) and (1 0 1 t e.g., 1( n +1)15( n +15)49( n t . is a square.
5 6 Why did the first three This is linear algebra over F 2 . completely factored congruences Guaranteed to find subsequence have square product? if number of vectors Was it just blind luck? exceeds length of each vector. Yes. The exponent vectors e.g. for n = 671: 1( n + 1) = 2 5 3 1 5 0 7 1 ; (1 ; 0 ; 4 ; 1) ; (6 ; 3 ; 2 ; 0) ; (1 ; 1 ; 2 ; 3) 4( n + 4) = 2 2 3 3 5 2 7 0 ; happened to have sum 0 mod 2. 15( n + 15) = 2 1 3 1 5 1 7 3 ; But we didn’t need this luck! 49( n + 49) = 2 4 3 2 5 1 7 2 ; Given long sequence of vectors, 64( n + 64) = 2 6 3 1 5 1 7 2 . easily find nonempty subsequence with sum 0 mod 2. F 2 -kernel of exponent matrix is gen by (0 1 0 1 1) and (1 0 1 1 0); e.g., 1( n +1)15( n +15)49( n +49) is a square.
5 6 did the first three This is linear algebra over F 2 . Plausible completely factored congruences Guaranteed to find subsequence separate square product? if number of vectors of any n just blind luck? exceeds length of each vector. Given n The exponent vectors e.g. for n = 671: Try to completely 1( n + 1) = 2 5 3 1 5 0 7 1 ; ; 1) ; (6 ; 3 ; 2 ; 0) ; (1 ; 1 ; 2 ; 3) ˘ for i ∈ 4( n + 4) = 2 2 3 3 5 2 7 0 ; ened to have sum 0 mod 2. into products 15( n + 15) = 2 1 3 1 5 1 7 3 ; e didn’t need this luck! 49( n + 49) = 2 4 3 2 5 1 7 2 ; Look for long sequence of vectors, 64( n + 64) = 2 6 3 1 5 1 7 2 . with i ( n find nonempty subsequence and with sum 0 mod 2. F 2 -kernel of exponent matrix is gen by (0 1 0 1 1) and (1 0 1 1 0); Compute e.g., 1( n +1)15( n +15)49( n +49) s = Q i is a square. i ∈ I
5 6 first three This is linear algebra over F 2 . Plausible conjecture: red congruences Guaranteed to find subsequence separate the odd p duct? if number of vectors of any n , not just luck? exceeds length of each vector. Given n and parameter onent vectors e.g. for n = 671: Try to completely 1( n + 1) = 2 5 3 1 5 0 7 1 ; 2 ; 0) ; (1 ; 1 ; 2 ; 3) ˘ for i ∈ 1 ; 2 ; 3 ; : : : 4( n + 4) = 2 2 3 3 5 2 7 0 ; have sum 0 mod 2. into products of primes 15( n + 15) = 2 1 3 1 5 1 7 3 ; need this luck! 49( n + 49) = 2 4 3 2 5 1 7 2 ; Look for nonempty sequence of vectors, 64( n + 64) = 2 6 3 1 5 1 7 2 . with i ( n + i ) completely nonempty subsequence and with Q i ( n + 2. F 2 -kernel of exponent matrix is i ∈ I gen by (0 1 0 1 1) and (1 0 1 1 0); Compute gcd { n; s e.g., 1( n +1)15( n +15)49( n +49) r s = Q i and t = is a square. i ∈ I
5 6 This is linear algebra over F 2 . Plausible conjecture: Q sieve congruences Guaranteed to find subsequence separate the odd prime diviso if number of vectors of any n , not just 611. exceeds length of each vector. Given n and parameter y : rs e.g. for n = 671: Try to completely factor i ( n 1( n + 1) = 2 5 3 1 5 0 7 1 ; ; 2 ; 3) 1 ; 2 ; 3 ; : : : ; y 2 ¯ ˘ for i ∈ 4( n + 4) = 2 2 3 3 5 2 7 0 ; mod 2. into products of primes ≤ y . 15( n + 15) = 2 1 3 1 5 1 7 3 ; luck! 49( n + 49) = 2 4 3 2 5 1 7 2 ; Look for nonempty set I of i vectors, 64( n + 64) = 2 6 3 1 5 1 7 2 . with i ( n + i ) completely facto subsequence and with Q i ( n + i ) square. F 2 -kernel of exponent matrix is i ∈ I gen by (0 1 0 1 1) and (1 0 1 1 0); Compute gcd { n; s − t } where e.g., 1( n +1)15( n +15)49( n +49) r Q s = Q i and t = i ( n + is a square. i ∈ I i ∈ I
6 7 This is linear algebra over F 2 . Plausible conjecture: Q sieve can Guaranteed to find subsequence separate the odd prime divisors if number of vectors of any n , not just 611. exceeds length of each vector. Given n and parameter y : e.g. for n = 671: Try to completely factor i ( n + i ) 1( n + 1) = 2 5 3 1 5 0 7 1 ; 1 ; 2 ; 3 ; : : : ; y 2 ¯ ˘ for i ∈ 4( n + 4) = 2 2 3 3 5 2 7 0 ; into products of primes ≤ y . 15( n + 15) = 2 1 3 1 5 1 7 3 ; 49( n + 49) = 2 4 3 2 5 1 7 2 ; Look for nonempty set I of i ’s 64( n + 64) = 2 6 3 1 5 1 7 2 . with i ( n + i ) completely factored and with Q i ( n + i ) square. F 2 -kernel of exponent matrix is i ∈ I gen by (0 1 0 1 1) and (1 0 1 1 0); Compute gcd { n; s − t } where e.g., 1( n +1)15( n +15)49( n +49) r Q s = Q i and t = i ( n + i ). is a square. i ∈ I i ∈ I
6 7 linear algebra over F 2 . Plausible conjecture: Q sieve can How large ranteed to find subsequence separate the odd prime divisors for this to number of vectors of any n , not just 611. Uniform exceeds length of each vector. has n 1 =u Given n and parameter y : r n = 671: roughly u Try to completely factor i ( n + i ) 1) = 2 5 3 1 5 0 7 1 ; 1 ; 2 ; 3 ; : : : ; y 2 ¯ ˘ for i ∈ Plausible 4) = 2 2 3 3 5 2 7 0 ; into products of primes ≤ y . Q sieve succe 15) = 2 1 3 1 5 1 7 3 ; with y = 49) = 2 4 3 2 5 1 7 2 ; Look for nonempty set I of i ’s for all n 64) = 2 6 3 1 5 1 7 2 . with i ( n + i ) completely factored here o (1) and with Q i ( n + i ) square. ernel of exponent matrix is i ∈ I (0 1 0 1 1) and (1 0 1 1 0); Compute gcd { n; s − t } where 1( n +1)15( n +15)49( n +49) r Q s = Q i and t = i ( n + i ). square. i ∈ I i ∈ I
6 7 algebra over F 2 . Plausible conjecture: Q sieve can How large does y have find subsequence separate the odd prime divisors for this to find a squa vectors of any n , not just 611. Uniform random integer of each vector. has n 1 =u -smoothness Given n and parameter y : roughly u − u . 671: Try to completely factor i ( n + i ) 3 1 5 0 7 1 ; 1 ; 2 ; 3 ; : : : ; y 2 ¯ ˘ for i ∈ Plausible conjecture: 3 3 5 2 7 0 ; into products of primes ≤ y . Q sieve succeeds 3 1 5 1 7 3 ; with y = ⌊ n 1 =u ⌋ 3 2 5 1 7 2 ; Look for nonempty set I of i ’s for all n ≥ u (1+ o (1)) 3 1 5 1 7 2 . with i ( n + i ) completely factored here o (1) is as u → and with Q i ( n + i ) square. onent matrix is i ∈ I 1) and (1 0 1 1 0); Compute gcd { n; s − t } where n +15)49( n +49) r Q s = Q i and t = i ( n + i ). i ∈ I i ∈ I
6 7 F 2 . Plausible conjecture: Q sieve can How large does y have to be subsequence separate the odd prime divisors for this to find a square? of any n , not just 611. Uniform random integer in [1 vector. has n 1 =u -smoothness chance Given n and parameter y : roughly u − u . Try to completely factor i ( n + i ) 1 ; 2 ; 3 ; : : : ; y 2 ¯ ˘ for i ∈ Plausible conjecture: into products of primes ≤ y . Q sieve succeeds with y = ⌊ n 1 =u ⌋ Look for nonempty set I of i ’s for all n ≥ u (1+ o (1)) u 2 ; with i ( n + i ) completely factored here o (1) is as u → ∞ . and with Q i ( n + i ) square. matrix is i ∈ I 0 1 1 0); Compute gcd { n; s − t } where 15)49( n +49) r Q s = Q i and t = i ( n + i ). i ∈ I i ∈ I
7 8 Plausible conjecture: Q sieve can How large does y have to be separate the odd prime divisors for this to find a square? of any n , not just 611. Uniform random integer in [1 ; n ] has n 1 =u -smoothness chance Given n and parameter y : roughly u − u . Try to completely factor i ( n + i ) 1 ; 2 ; 3 ; : : : ; y 2 ¯ ˘ for i ∈ Plausible conjecture: into products of primes ≤ y . Q sieve succeeds with y = ⌊ n 1 =u ⌋ Look for nonempty set I of i ’s for all n ≥ u (1+ o (1)) u 2 ; with i ( n + i ) completely factored here o (1) is as u → ∞ . and with Q i ( n + i ) square. i ∈ I Compute gcd { n; s − t } where r Q s = Q i and t = i ( n + i ). i ∈ I i ∈ I
7 8 Plausible conjecture: Q sieve can How large does y have to be More generally q` 1 rate the odd prime divisors for this to find a square? exp 2 n , not just 611. conjectured Uniform random integer in [1 ; n ] is 1 =y c + o has n 1 =u -smoothness chance n and parameter y : roughly u − u . Find enough completely factor i ( n + i ) by changing 1 ; 2 ; 3 ; : : : ; y 2 ¯ ˘ Plausible conjecture: replace y roducts of primes ≤ y . Q sieve succeeds r“ ( with y = ⌊ n 1 =u ⌋ exp for nonempty set I of i ’s for all n ≥ u (1+ o (1)) u 2 ; ( n + i ) completely factored Increasing here o (1) is as u → ∞ . with Q i ( n + i ) square. increases i ∈ I reduces linea Compute gcd { n; s − t } where So linear r Q i and t = i ( n + i ). when y is i ∈ I
7 8 conjecture: Q sieve can How large does y have to be More generally, if y q` 1 prime divisors for this to find a square? ´ exp 2 c + o (1) log just 611. conjectured y -smo Uniform random integer in [1 ; n ] is 1 =y c + o (1) . has n 1 =u -smoothness chance rameter y : roughly u − u . Find enough smooth completely factor i ( n + i ) by changing the range : : ; y 2 ¯ Plausible conjecture: replace y 2 with y c +1+ primes ≤ y . Q sieve succeeds r“ ( c +1) 2 + o (1) with y = ⌊ n 1 =u ⌋ exp 2 c mpty set I of i ’s for all n ≥ u (1+ o (1)) u 2 ; completely factored Increasing c past 1 here o (1) is as u → ∞ . + i ) square. increases number of reduces linear-algeb n; s − t } where So linear algebra never r Q i ( n + i ). when y is chosen p i ∈ I
7 8 sieve can How large does y have to be More generally, if y ∈ q` 1 divisors for this to find a square? ´ exp 2 c + o (1) log n log log conjectured y -smoothness chance Uniform random integer in [1 ; n ] is 1 =y c + o (1) . has n 1 =u -smoothness chance roughly u − u . Find enough smooth congruences ( n + i ) by changing the range of i ’s: Plausible conjecture: replace y 2 with y c +1+ o (1) = y . Q sieve succeeds r“ ( c +1) 2 + o (1) ” with y = ⌊ n 1 =u ⌋ exp log n log 2 c of i ’s for all n ≥ u (1+ o (1)) u 2 ; factored Increasing c past 1 here o (1) is as u → ∞ . re. increases number of i ’s but reduces linear-algebra cost. where So linear algebra never domin + i ). when y is chosen properly.
8 9 How large does y have to be More generally, if y ∈ q` 1 for this to find a square? ´ exp 2 c + o (1) log n log log n , conjectured y -smoothness chance Uniform random integer in [1 ; n ] is 1 =y c + o (1) . has n 1 =u -smoothness chance roughly u − u . Find enough smooth congruences by changing the range of i ’s: Plausible conjecture: replace y 2 with y c +1+ o (1) = Q sieve succeeds r“ ( c +1) 2 + o (1) ” with y = ⌊ n 1 =u ⌋ exp log n log log n . 2 c for all n ≥ u (1+ o (1)) u 2 ; Increasing c past 1 here o (1) is as u → ∞ . increases number of i ’s but reduces linear-algebra cost. So linear algebra never dominates when y is chosen properly.
8 9 large does y have to be More generally, if y ∈ Improving q` 1 is to find a square? ´ exp 2 c + o (1) log n log log n , Smoothness conjectured y -smoothness chance rm random integer in [1 ; n ] degrades is 1 =y c + o (1) . =u -smoothness chance Smaller fo roughly u − u . Find enough smooth congruences Crude analysis: by changing the range of i ’s: Plausible conjecture: ≈ yn if i replace y 2 with y c +1+ o (1) = ≈ y 2 n if sieve succeeds r“ ( c +1) 2 + o (1) ” = ⌊ n 1 =u ⌋ exp log n log log n . 2 c More careful n ≥ u (1+ o (1)) u 2 ; n + i do Increasing c past 1 (1) is as u → ∞ . i is alwa increases number of i ’s but only 30% reduces linear-algebra cost. So linear algebra never dominates Can we select when y is chosen properly. to avoid
8 9 y have to be More generally, if y ∈ Improving smoothness q` 1 square? ´ exp 2 c + o (1) log n log log n , Smoothness chance conjectured y -smoothness chance integer in [1 ; n ] degrades as i grows. is 1 =y c + o (1) . Smaller for i ≈ y 2 othness chance Find enough smooth congruences Crude analysis: i ( n by changing the range of i ’s: conjecture: ≈ yn if i ≈ y ; replace y 2 with y c +1+ o (1) = ≈ y 2 n if i ≈ y 2 . eds r“ ( c +1) 2 + o (1) ” ⌋ exp log n log log n . 2 c More careful analysis: (1)) u 2 ; n + i doesn’t degrade, Increasing c past 1 → ∞ . i is always smooth increases number of i ’s but only 30% chance fo reduces linear-algebra cost. So linear algebra never dominates Can we select congruences when y is chosen properly. to avoid this degradation?
8 9 be More generally, if y ∈ Improving smoothness chances q` 1 ´ exp 2 c + o (1) log n log log n , Smoothness chance of i ( n + conjectured y -smoothness chance [1 ; n ] degrades as i grows. is 1 =y c + o (1) . Smaller for i ≈ y 2 than for i chance Find enough smooth congruences Crude analysis: i ( n + i ) gro by changing the range of i ’s: ≈ yn if i ≈ y ; replace y 2 with y c +1+ o (1) = ≈ y 2 n if i ≈ y 2 . r“ ( c +1) 2 + o (1) ” exp log n log log n . 2 c More careful analysis: n + i doesn’t degrade, but Increasing c past 1 i is always smooth for i ≤ y increases number of i ’s but only 30% chance for i ≈ y 2 . reduces linear-algebra cost. So linear algebra never dominates Can we select congruences when y is chosen properly. to avoid this degradation?
9 10 More generally, if y ∈ Improving smoothness chances q` 1 ´ exp 2 c + o (1) log n log log n , Smoothness chance of i ( n + i ) conjectured y -smoothness chance degrades as i grows. is 1 =y c + o (1) . Smaller for i ≈ y 2 than for i ≈ y . Find enough smooth congruences Crude analysis: i ( n + i ) grows. by changing the range of i ’s: ≈ yn if i ≈ y ; replace y 2 with y c +1+ o (1) = ≈ y 2 n if i ≈ y 2 . r“ ( c +1) 2 + o (1) ” exp log n log log n . 2 c More careful analysis: n + i doesn’t degrade, but Increasing c past 1 i is always smooth for i ≤ y , increases number of i ’s but only 30% chance for i ≈ y 2 . reduces linear-algebra cost. So linear algebra never dominates Can we select congruences when y is chosen properly. to avoid this degradation?
9 10 generally, if y ∈ Improving smoothness chances Choose q ` 1 Choose a ´ 2 c + o (1) log n log log n , Smoothness chance of i ( n + i ) arithmetic conjectured y -smoothness chance degrades as i grows. + o (1) . where q Smaller for i ≈ y 2 than for i ≈ y . e.g. progression enough smooth congruences Crude analysis: i ( n + i ) grows. 2 q − ( n mo changing the range of i ’s: ≈ yn if i ≈ y ; etc. replace y 2 with y c +1+ o (1) = ≈ y 2 n if i ≈ y 2 . “ ( c +1) 2 + o (1) Check smo ” log n log log n . 2 c More careful analysis: generalized n + i doesn’t degrade, but for i ’s in Increasing c past 1 i is always smooth for i ≤ y , e.g. check increases number of i ’s but only 30% chance for i ≈ y 2 . smooth fo reduces linear-algebra cost. linear algebra never dominates Can we select congruences Try many y is chosen properly. to avoid this degradation? Rare for
9 10 if y ∈ Improving smoothness chances Choose q , square of Choose a “ q -sublattice” ´ (1) log n log log n , Smoothness chance of i ( n + i ) arithmetic progression -smoothness chance degrades as i grows. where q divides each Smaller for i ≈ y 2 than for i ≈ y . e.g. progression q smooth congruences Crude analysis: i ( n + i ) grows. 2 q − ( n mod q ), 3 q range of i ’s: ≈ yn if i ≈ y ; etc. y c +1+ o (1) = ≈ y 2 n if i ≈ y 2 . Check smoothness (1) ” log n log log n . More careful analysis: generalized congruence n + i doesn’t degrade, but for i ’s in this sublattice. past 1 i is always smooth for i ≤ y , e.g. check whethe er of i ’s but only 30% chance for i ≈ y 2 . smooth for i = q − r-algebra cost. never dominates Can we select congruences Try many large q ’s. chosen properly. to avoid this degradation? Rare for i ’s to overlap.
9 10 Improving smoothness chances Choose q , square of large prime. Choose a “ q -sublattice” of i log n , Smoothness chance of i ( n + i ) arithmetic progression of i ’s chance degrades as i grows. where q divides each i ( n + i Smaller for i ≈ y 2 than for i ≈ y . e.g. progression q − ( n mod congruences Crude analysis: i ( n + i ) grows. 2 q − ( n mod q ), 3 q − ( n mod ’s: ≈ yn if i ≈ y ; etc. = ≈ y 2 n if i ≈ y 2 . Check smoothness of log log n . More careful analysis: generalized congruence i ( n + n + i doesn’t degrade, but for i ’s in this sublattice. i is always smooth for i ≤ y , e.g. check whether i; ( n + i ) but only 30% chance for i ≈ y 2 . smooth for i = q − ( n mod q cost. ominates Can we select congruences Try many large q ’s. . to avoid this degradation? Rare for i ’s to overlap.
10 11 Improving smoothness chances Choose q , square of large prime. Choose a “ q -sublattice” of i ’s: Smoothness chance of i ( n + i ) arithmetic progression of i ’s degrades as i grows. where q divides each i ( n + i ). Smaller for i ≈ y 2 than for i ≈ y . e.g. progression q − ( n mod q ), Crude analysis: i ( n + i ) grows. 2 q − ( n mod q ), 3 q − ( n mod q ), ≈ yn if i ≈ y ; etc. ≈ y 2 n if i ≈ y 2 . Check smoothness of More careful analysis: generalized congruence i ( n + i ) =q n + i doesn’t degrade, but for i ’s in this sublattice. i is always smooth for i ≤ y , e.g. check whether i; ( n + i ) =q are only 30% chance for i ≈ y 2 . smooth for i = q − ( n mod q ) etc. Can we select congruences Try many large q ’s. to avoid this degradation? Rare for i ’s to overlap.
10 11 roving smoothness chances Choose q , square of large prime. e.g. n = Choose a “ q -sublattice” of i ’s: othness chance of i ( n + i ) Original arithmetic progression of i ’s degrades as i grows. i n where q divides each i ( n + i ). Smaller for i ≈ y 2 than for i ≈ y . 1 314159265358979324 e.g. progression q − ( n mod q ), 2 314159265358979325 analysis: i ( n + i ) grows. 2 q − ( n mod q ), 3 q − ( n mod q ), 3 314159265358979326 if i ≈ y ; etc. if i ≈ y 2 . Use 997 2 Check smoothness of i ∈ 802458 careful analysis: generalized congruence i ( n + i ) =q doesn’t degrade, but for i ’s in this sublattice. ays smooth for i ≤ y , 802458 e.g. check whether i; ( n + i ) =q are 30% chance for i ≈ y 2 . 1796467 smooth for i = q − ( n mod q ) etc. 2790476 e select congruences Try many large q ’s. avoid this degradation? Rare for i ’s to overlap.
10 11 othness chances Choose q , square of large prime. e.g. n = 314159265358979323: Choose a “ q -sublattice” of i ’s: chance of i ( n + i ) Original Q sieve: arithmetic progression of i ’s grows. i n + i where q divides each i ( n + i ). 2 than for i ≈ y . 1 314159265358979324 e.g. progression q − ( n mod q ), 2 314159265358979325 i ( n + i ) grows. 2 q − ( n mod q ), 3 q − ( n mod q ), 3 314159265358979326 etc. . Use 997 2 -sublattice, Check smoothness of i ∈ 802458 + 994009 analysis: generalized congruence i ( n + i ) =q degrade, but i ( n + for i ’s in this sublattice. oth for i ≤ y , 802458 316052737309 e.g. check whether i; ( n + i ) =q are chance for i ≈ y 2 . 1796467 316052737310 smooth for i = q − ( n mod q ) etc. 2790476 316052737311 congruences Try many large q ’s. degradation? Rare for i ’s to overlap.
10 11 chances Choose q , square of large prime. e.g. n = 314159265358979323: Choose a “ q -sublattice” of i ’s: + i ) Original Q sieve: arithmetic progression of i ’s i n + i where q divides each i ( n + i ). r i ≈ y . 1 314159265358979324 e.g. progression q − ( n mod q ), 2 314159265358979325 grows. 2 q − ( n mod q ), 3 q − ( n mod q ), 3 314159265358979326 etc. Use 997 2 -sublattice, Check smoothness of i ∈ 802458 + 994009 Z : generalized congruence i ( n + i ) =q ( n + i ) = 997 2 but i for i ’s in this sublattice. y , 802458 316052737309 e.g. check whether i; ( n + i ) =q are 2 . 1796467 316052737310 smooth for i = q − ( n mod q ) etc. 2790476 316052737311 Try many large q ’s. Rare for i ’s to overlap.
11 12 Choose q , square of large prime. e.g. n = 314159265358979323: Choose a “ q -sublattice” of i ’s: Original Q sieve: arithmetic progression of i ’s i n + i where q divides each i ( n + i ). 1 314159265358979324 e.g. progression q − ( n mod q ), 2 314159265358979325 2 q − ( n mod q ), 3 q − ( n mod q ), 3 314159265358979326 etc. Use 997 2 -sublattice, Check smoothness of i ∈ 802458 + 994009 Z : generalized congruence i ( n + i ) =q ( n + i ) = 997 2 i for i ’s in this sublattice. 802458 316052737309 e.g. check whether i; ( n + i ) =q are 1796467 316052737310 smooth for i = q − ( n mod q ) etc. 2790476 316052737311 Try many large q ’s. Rare for i ’s to overlap.
11 12 ose q , square of large prime. e.g. n = 314159265358979323: Crude analysis: ose a “ q -sublattice” of i ’s: eliminate Original Q sieve: rithmetic progression of i ’s Have practically i n + i q divides each i ( n + i ). of generalized 1 314159265358979324 rogression q − ( n mod q ), ( q − ( n mo 2 314159265358979325 n mod q ), 3 q − ( n mod q ), between 3 314159265358979326 More careful Use 997 2 -sublattice, smoothness of are even i ∈ 802458 + 994009 Z : generalized congruence i ( n + i ) =q For q ≈ n ( n + i ) = 997 2 i in this sublattice. i ≈ ( n + 802458 316052737309 check whether i; ( n + i ) =q are so smoothness 1796467 316052737310 oth for i = q − ( n mod q ) etc. ( u= 2) − u= 2790476 316052737311 2 u times many large q ’s. for i ’s to overlap.
11 12 re of large prime. e.g. n = 314159265358979323: Crude analysis: Sublattices -sublattice” of i ’s: eliminate the growth Original Q sieve: rogression of i ’s Have practically unlimited i n + i each i ( n + i ). of generalized congruences 1 314159265358979324 ( q − ( n mod q )) n + q − ( n mod q ), 2 314159265358979325 3 q − ( n mod q ), between 0 and n . 3 314159265358979326 More careful analysis: Use 997 2 -sublattice, othness of are even better than i ∈ 802458 + 994009 Z : congruence i ( n + i ) =q For q ≈ n 1 = 2 have ( n + i ) = 997 2 i sublattice. i ≈ ( n + i ) =q ≈ n 802458 316052737309 her i; ( n + i ) =q are so smoothness chance 1796467 316052737310 − ( n mod q ) etc. ( u= 2) − u= 2 ( u= 2) − u= 2790476 316052737311 2 u times larger than q ’s. overlap.
11 12 prime. e.g. n = 314159265358979323: Crude analysis: Sublattices of i ’s: eliminate the growth problem. Original Q sieve: ’s Have practically unlimited supply i n + i i ). of generalized congruences 1 314159265358979324 ( q − ( n mod q )) n + q − ( n mod d q ), 2 314159265358979325 q mod q ), between 0 and n . 3 314159265358979326 More careful analysis: Sublattices Use 997 2 -sublattice, are even better than that! i ∈ 802458 + 994009 Z : + i ) =q For q ≈ n 1 = 2 have ( n + i ) = 997 2 i i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 802458 316052737309 i ) =q are so smoothness chance is roughly 1796467 316052737310 d q ) etc. ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u 2790476 316052737311 2 u times larger than before.
12 13 e.g. n = 314159265358979323: Crude analysis: Sublattices eliminate the growth problem. Original Q sieve: Have practically unlimited supply i n + i of generalized congruences 1 314159265358979324 ( q − ( n mod q )) n + q − ( n mod q ) 2 314159265358979325 q between 0 and n . 3 314159265358979326 More careful analysis: Sublattices Use 997 2 -sublattice, are even better than that! i ∈ 802458 + 994009 Z : For q ≈ n 1 = 2 have ( n + i ) = 997 2 i i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 802458 316052737309 so smoothness chance is roughly 1796467 316052737310 ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u u , 2790476 316052737311 2 u times larger than before.
12 13 = 314159265358979323: Crude analysis: Sublattices Even larger eliminate the growth problem. from changing Original Q sieve: Have practically unlimited supply “Quadratic n + i of generalized congruences i 2 − n with 314159265358979324 ( q − ( n mod q )) n + q − ( n mod q ) have i 2 − 314159265358979325 q between 0 and n . much smaller 314159265358979326 More careful analysis: Sublattices 997 2 -sublattice, are even better than that! 802458 + 994009 Z : For q ≈ n 1 = 2 have ( n + i ) = 997 2 i i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 802458 316052737309 so smoothness chance is roughly 1796467 316052737310 ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u u , 2790476 316052737311 2 u times larger than before.
12 13 314159265358979323: Crude analysis: Sublattices Even larger improvements eliminate the growth problem. from changing polynomial sieve: Have practically unlimited supply “Quadratic sieve” i 2 − n with i ≈ √ of generalized congruences 314159265358979324 ( q − ( n mod q )) n + q − ( n mod q ) have i 2 − n ≈ n 1 = 314159265358979325 q between 0 and n . much smaller than 314159265358979326 More careful analysis: Sublattices -sublattice, are even better than that! 994009 Z : For q ≈ n 1 = 2 have + i ) = 997 2 i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 316052737309 so smoothness chance is roughly 316052737310 ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u u , 316052737311 2 u times larger than before.
12 13 314159265358979323: Crude analysis: Sublattices Even larger improvements eliminate the growth problem. from changing polynomial i ( Have practically unlimited supply “Quadratic sieve” (QS) uses i 2 − n with i ≈ √ n ; of generalized congruences 314159265358979324 ( q − ( n mod q )) n + q − ( n mod q ) have i 2 − n ≈ n 1 = 2+ o (1) , 314159265358979325 q between 0 and n . much smaller than n . 314159265358979326 More careful analysis: Sublattices are even better than that! For q ≈ n 1 = 2 have 2 i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 316052737309 so smoothness chance is roughly 316052737310 ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u u , 316052737311 2 u times larger than before.
13 14 Crude analysis: Sublattices Even larger improvements eliminate the growth problem. from changing polynomial i ( n + i ). Have practically unlimited supply “Quadratic sieve” (QS) uses i 2 − n with i ≈ √ n ; of generalized congruences ( q − ( n mod q )) n + q − ( n mod q ) have i 2 − n ≈ n 1 = 2+ o (1) , q between 0 and n . much smaller than n . More careful analysis: Sublattices are even better than that! For q ≈ n 1 = 2 have i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 so smoothness chance is roughly ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u u , 2 u times larger than before.
13 14 Crude analysis: Sublattices Even larger improvements eliminate the growth problem. from changing polynomial i ( n + i ). Have practically unlimited supply “Quadratic sieve” (QS) uses i 2 − n with i ≈ √ n ; of generalized congruences ( q − ( n mod q )) n + q − ( n mod q ) have i 2 − n ≈ n 1 = 2+ o (1) , q between 0 and n . much smaller than n . More careful analysis: Sublattices “MPQS” improves o (1) using sublattices: ( i 2 − n ) =q . are even better than that! For q ≈ n 1 = 2 have But still ≈ n 1 = 2 . i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 so smoothness chance is roughly ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u u , 2 u times larger than before.
13 14 Crude analysis: Sublattices Even larger improvements eliminate the growth problem. from changing polynomial i ( n + i ). Have practically unlimited supply “Quadratic sieve” (QS) uses i 2 − n with i ≈ √ n ; of generalized congruences ( q − ( n mod q )) n + q − ( n mod q ) have i 2 − n ≈ n 1 = 2+ o (1) , q between 0 and n . much smaller than n . More careful analysis: Sublattices “MPQS” improves o (1) using sublattices: ( i 2 − n ) =q . are even better than that! For q ≈ n 1 = 2 have But still ≈ n 1 = 2 . i ≈ ( n + i ) =q ≈ n 1 = 2 ≈ y u= 2 “Number-field sieve” (NFS) so smoothness chance is roughly achieves n o (1) . ( u= 2) − u= 2 ( u= 2) − u= 2 = 2 u =u u , 2 u times larger than before.
13 14 analysis: Sublattices Even larger improvements Generalizing eliminate the growth problem. from changing polynomial i ( n + i ). The Q sieve ractically unlimited supply “Quadratic sieve” (QS) uses the numb i 2 − n with i ≈ √ n ; generalized congruences mod q )) n + q − ( n mod q ) Recall ho have i 2 − n ≈ n 1 = 2+ o (1) , q factors 611: een 0 and n . much smaller than n . Form a squa careful analysis: Sublattices “MPQS” improves o (1) as product using sublattices: ( i 2 − n ) =q . even better than that! for several ≈ n 1 = 2 have But still ≈ n 1 = 2 . 14(625) + i ) =q ≈ n 1 = 2 ≈ y u= 2 “Number-field sieve” (NFS) = 4410000 othness chance is roughly achieves n o (1) . u= 2 ( u= 2) − u= 2 = 2 u =u u , gcd { 611 ; = 47. times larger than before.
13 14 Sublattices Even larger improvements Generalizing beyond growth problem. from changing polynomial i ( n + i ). The Q sieve is a sp unlimited supply “Quadratic sieve” (QS) uses the number-field sie i 2 − n with i ≈ √ n ; congruences + q − ( n mod q ) Recall how the Q sieve have i 2 − n ≈ n 1 = 2+ o (1) , q factors 611: . much smaller than n . Form a square analysis: Sublattices “MPQS” improves o (1) as product of i ( i + using sublattices: ( i 2 − n ) =q . than that! for several pairs ( i; But still ≈ n 1 = 2 . have 14(625) · 64(675) · n 1 = 2 ≈ y u= 2 = 4410000 2 . “Number-field sieve” (NFS) chance is roughly achieves n o (1) . − u= 2 = 2 u =u u , gcd { 611 ; 14 · 64 · 75 = 47. than before.
13 14 Sublattices Even larger improvements Generalizing beyond Q roblem. from changing polynomial i ( n + i ). The Q sieve is a special case supply “Quadratic sieve” (QS) uses the number-field sieve. i 2 − n with i ≈ √ n ; congruences mod q ) Recall how the Q sieve have i 2 − n ≈ n 1 = 2+ o (1) , factors 611: much smaller than n . Form a square Sublattices “MPQS” improves o (1) as product of i ( i + 611 j ) using sublattices: ( i 2 − n ) =q . for several pairs ( i; j ): But still ≈ n 1 = 2 . 14(625) · 64(675) · 75(686) u= 2 = 4410000 2 . “Number-field sieve” (NFS) roughly achieves n o (1) . =u u , gcd { 611 ; 14 · 64 · 75 − 4410000 = 47. re.
14 15 Even larger improvements Generalizing beyond Q from changing polynomial i ( n + i ). The Q sieve is a special case of “Quadratic sieve” (QS) uses the number-field sieve. i 2 − n with i ≈ √ n ; Recall how the Q sieve have i 2 − n ≈ n 1 = 2+ o (1) , factors 611: much smaller than n . Form a square “MPQS” improves o (1) as product of i ( i + 611 j ) using sublattices: ( i 2 − n ) =q . for several pairs ( i; j ): But still ≈ n 1 = 2 . 14(625) · 64(675) · 75(686) = 4410000 2 . “Number-field sieve” (NFS) achieves n o (1) . gcd { 611 ; 14 · 64 · 75 − 4410000 } = 47.
√ 14 15 larger improvements Generalizing beyond Q The Q ( changing polynomial i ( n + i ). factors 611 The Q sieve is a special case of “Quadratic sieve” (QS) uses the number-field sieve. Form a squa with i ≈ √ n ; as product Recall how the Q sieve − n ≈ n 1 = 2+ o (1) , for several factors 611: ( − 11 + 3 smaller than n . Form a square · (3 “MPQS” improves o (1) as product of i ( i + 611 j ) = (112 − sublattices: ( i 2 − n ) =q . for several pairs ( i; j ): still ≈ n 1 = 2 . Compute 14(625) · 64(675) · 75(686) s = ( − 11 = 4410000 2 . “Number-field sieve” (NFS) t = 112 − achieves n o (1) . gcd { 611 ; 14 · 64 · 75 − 4410000 } gcd { 611 ; = 47.
√ 14 15 rovements Generalizing beyond Q The Q ( 14) sieve olynomial i ( n + i ). factors 611 as follo The Q sieve is a special case of sieve” (QS) uses the number-field sieve. Form a square √ n ; as product of ( i + Recall how the Q sieve 1 = 2+ o (1) , for several pairs ( i; factors 611: ( − 11 + 3 · 25)( − 11 than n . Form a square · (3 + 25)(3 + √ roves o (1) as product of i ( i + 611 j ) 14) 2 = (112 − 16 sublattices: ( i 2 − n ) =q . for several pairs ( i; j ): . Compute 14(625) · 64(675) · 75(686) s = ( − 11 + 3 · 25) = 4410000 2 . sieve” (NFS) t = 112 − 16 · 25, gcd { 611 ; 14 · 64 · 75 − 4410000 } gcd { 611 ; s − t } = = 47.
√ 14 15 Generalizing beyond Q The Q ( 14) sieve i ( n + i ). factors 611 as follows: The Q sieve is a special case of uses the number-field sieve. Form a square √ as product of ( i + 25 j )( i + Recall how the Q sieve for several pairs ( i; j ): √ factors 611: ( − 11 + 3 · 25)( − 11 + 3 14) √ Form a square · (3 + 25)(3 + 14) √ as product of i ( i + 611 j ) 14) 2 . = (112 − 16 =q . for several pairs ( i; j ): Compute 14(625) · 64(675) · 75(686) s = ( − 11 + 3 · 25) · (3 + 25), = 4410000 2 . (NFS) t = 112 − 16 · 25, gcd { 611 ; 14 · 64 · 75 − 4410000 } gcd { 611 ; s − t } = 13. = 47.
√ 15 16 Generalizing beyond Q The Q ( 14) sieve factors 611 as follows: The Q sieve is a special case of the number-field sieve. Form a square √ as product of ( i + 25 j )( i + 14 j ) Recall how the Q sieve for several pairs ( i; j ): √ factors 611: ( − 11 + 3 · 25)( − 11 + 3 14) √ Form a square · (3 + 25)(3 + 14) √ as product of i ( i + 611 j ) 14) 2 . = (112 − 16 for several pairs ( i; j ): Compute 14(625) · 64(675) · 75(686) s = ( − 11 + 3 · 25) · (3 + 25), = 4410000 2 . t = 112 − 16 · 25, gcd { 611 ; 14 · 64 · 75 − 4410000 } gcd { 611 ; s − t } = 13. = 47.
√ 15 16 Generalizing beyond Q The Q ( 14) sieve Why does factors 611 as follows: sieve is a special case of Answer: √ number-field sieve. Form a square Z [ 14] → √ since 25 2 as product of ( i + 25 j )( i + 14 j ) how the Q sieve for several pairs ( i; j ): √ 611: Apply ring ( − 11 + 3 · 25)( − 11 + 3 14) √ ( − 11 + 3 a square · (3 + 25)(3 + 14) √ · (3 duct of i ( i + 611 j ) 14) 2 . = (112 − 16 = (112 − everal pairs ( i; j ): Compute i.e. s 2 = 14(625) · 64(675) · 75(686) s = ( − 11 + 3 · 25) · (3 + 25), 4410000 2 . Unsurprising t = 112 − 16 · 25, 611 ; 14 · 64 · 75 − 4410000 } gcd { 611 ; s − t } = 13.
√ 15 16 ond Q The Q ( 14) sieve Why does this work? factors 611 as follows: special case of Answer: Have ring √ sieve. Form a square Z [ 14] → Z = 611, √ since 25 2 = 14 in Z as product of ( i + 25 j )( i + 14 j ) Q sieve for several pairs ( i; j ): √ Apply ring morphism ( − 11 + 3 · 25)( − 11 + 3 14) √ ( − 11 + 3 · 25)( − 11 · (3 + 25)(3 + 14) √ · (3 + 25)(3 + i + 611 j ) 14) 2 . = (112 − 16 = (112 − 16 · 25) 2 ( i; j ): Compute i.e. s 2 = t 2 in Z = 611. 64(675) · 75(686) s = ( − 11 + 3 · 25) · (3 + 25), Unsurprising to find t = 112 − 16 · 25, · 75 − 4410000 } gcd { 611 ; s − t } = 13.
√ 15 16 The Q ( 14) sieve Why does this work? factors 611 as follows: case of Answer: Have ring morphism √ √ Form a square Z [ 14] → Z = 611, 14 �→ 25, √ since 25 2 = 14 in Z = 611. as product of ( i + 25 j )( i + 14 j ) for several pairs ( i; j ): √ Apply ring morphism to squa ( − 11 + 3 · 25)( − 11 + 3 14) √ ( − 11 + 3 · 25)( − 11 + 3 · 25) · (3 + 25)(3 + 14) √ · (3 + 25)(3 + 25) 14) 2 . = (112 − 16 = (112 − 16 · 25) 2 in Z = 611. Compute i.e. s 2 = t 2 in Z = 611. 75(686) s = ( − 11 + 3 · 25) · (3 + 25), Unsurprising to find factor. t = 112 − 16 · 25, 4410000 } gcd { 611 ; s − t } = 13.
√ 16 17 The Q ( 14) sieve Why does this work? factors 611 as follows: Answer: Have ring morphism √ √ Form a square Z [ 14] → Z = 611, 14 �→ 25, √ since 25 2 = 14 in Z = 611. as product of ( i + 25 j )( i + 14 j ) for several pairs ( i; j ): √ Apply ring morphism to square: ( − 11 + 3 · 25)( − 11 + 3 14) √ ( − 11 + 3 · 25)( − 11 + 3 · 25) · (3 + 25)(3 + 14) √ · (3 + 25)(3 + 25) 14) 2 . = (112 − 16 = (112 − 16 · 25) 2 in Z = 611. Compute i.e. s 2 = t 2 in Z = 611. s = ( − 11 + 3 · 25) · (3 + 25), Unsurprising to find factor. t = 112 − 16 · 25, gcd { 611 ; s − t } = 13.
√ 16 17 ( 14) sieve Why does this work? Generalize 611 as follows: to ( f ; m Answer: Have ring morphism √ √ m ∈ Z , f a square Z [ 14] → Z = 611, 14 �→ 25, √ since 25 2 = 14 in Z = 611. duct of ( i + 25 j )( i + 14 j ) Write d = f = f d x d everal pairs ( i; j ): √ Apply ring morphism to square: 3 · 25)( − 11 + 3 14) √ ( − 11 + 3 · 25)( − 11 + 3 · 25) Can take (3 + 25)(3 + 14) √ · (3 + 25)(3 + 25) but larger 14) 2 . (112 − 16 = (112 − 16 · 25) 2 in Z = 611. better pa Compute i.e. s 2 = t 2 in Z = 611. Pick ¸ ∈ 11 + 3 · 25) · (3 + 25), Then f d ¸ Unsurprising to find factor. 112 − 16 · 25, monic g 611 ; s − t } = 13. Q ( ¸ ) ←O
16 17 sieve Why does this work? Generalize from ( x follows: to ( f ; m ) with irred Answer: Have ring morphism √ √ m ∈ Z , f ( m ) ∈ n Z Z [ 14] → Z = 611, 14 �→ 25, √ since 25 2 = 14 in Z = 611. + 25 j )( i + 14 j ) Write d = deg f , f = f d x d + · · · + f ( i; j ): √ Apply ring morphism to square: 11 + 3 14) √ ( − 11 + 3 · 25)( − 11 + 3 · 25) Can take f d = 1 fo 25)(3 + 14) · (3 + 25)(3 + 25) but larger f d allows 14) 2 . = (112 − 16 · 25) 2 in Z = 611. better parameter selection. i.e. s 2 = t 2 in Z = 611. Pick ¸ ∈ C , root of 25) · (3 + 25), Then f d ¸ is a root Unsurprising to find factor. 25, monic g = f d − 1 f ( d = 13. Q ( ¸ ) ←O← Z [ f d ¸
16 17 Generalize from ( x 2 − 14 ; 25) Why does this work? to ( f ; m ) with irred f ∈ Z [ x Answer: Have ring morphism √ √ m ∈ Z , f ( m ) ∈ n Z . Z [ 14] → Z = 611, 14 �→ 25, √ since 25 2 = 14 in Z = 611. 14 j ) Write d = deg f , f = f d x d + · · · + f 1 x 1 + f 0 x Apply ring morphism to square: 14) ( − 11 + 3 · 25)( − 11 + 3 · 25) Can take f d = 1 for simplicit · (3 + 25)(3 + 25) but larger f d allows = (112 − 16 · 25) 2 in Z = 611. better parameter selection. i.e. s 2 = t 2 in Z = 611. Pick ¸ ∈ C , root of f . 25), Then f d ¸ is a root of Unsurprising to find factor. monic g = f d − 1 f ( x=f d ) ∈ Z d f d ¸ �→ f d m Q ( ¸ ) ←O← Z [ f d ¸ ] − − − − − − − →
17 18 Generalize from ( x 2 − 14 ; 25) Why does this work? to ( f ; m ) with irred f ∈ Z [ x ], Answer: Have ring morphism √ √ m ∈ Z , f ( m ) ∈ n Z . Z [ 14] → Z = 611, 14 �→ 25, since 25 2 = 14 in Z = 611. Write d = deg f , f = f d x d + · · · + f 1 x 1 + f 0 x 0 . Apply ring morphism to square: ( − 11 + 3 · 25)( − 11 + 3 · 25) Can take f d = 1 for simplicity, · (3 + 25)(3 + 25) but larger f d allows = (112 − 16 · 25) 2 in Z = 611. better parameter selection. i.e. s 2 = t 2 in Z = 611. Pick ¸ ∈ C , root of f . Then f d ¸ is a root of Unsurprising to find factor. monic g = f d − 1 f ( x=f d ) ∈ Z [ x ]. d f d ¸ �→ f d m Q ( ¸ ) ←O← Z [ f d ¸ ] − − − − − − − → Z =n
17 18 Generalize from ( x 2 − 14 ; 25) does this work? Build squa to ( f ; m ) with irred f ∈ Z [ x ], congruences er: Have ring morphism √ m ∈ Z , f ( m ) ∈ n Z . with i Z + 14] → Z = 611, 14 �→ 25, 25 2 = 14 in Z = 611. Write d = deg f , Could replace f = f d x d + · · · + f 1 x 1 + f 0 x 0 . higher-deg ring morphism to square: quadratics 3 · 25)( − 11 + 3 · 25) Can take f d = 1 for simplicity, for some (3 + 25)(3 + 25) but larger f d allows But let’s (112 − 16 · 25) 2 in Z = 611. better parameter selection. Say we have = t 2 in Z = 611. Pick ¸ ∈ C , root of f . Q ( i;j ) ∈ S Then f d ¸ is a root of Unsurprising to find factor. in Q ( ¸ ); monic g = f d − 1 f ( x=f d ) ∈ Z [ x ]. d f d ¸ �→ f d m Q ( ¸ ) ←O← Z [ f d ¸ ] − − − − − − − → Z =n
17 18 Generalize from ( x 2 − 14 ; 25) ork? Build square in Q ( to ( f ; m ) with irred f ∈ Z [ x ], congruences ( i − j ring morphism √ m ∈ Z , f ( m ) ∈ n Z . with i Z + j Z = Z 611, 14 �→ 25, in Z = 611. Write d = deg f , Could replace i − j f = f d x d + · · · + f 1 x 1 + f 0 x 0 . higher-deg irred in rphism to square: quadratics seem fairly 11 + 3 · 25) Can take f d = 1 for simplicity, for some number fields. 25)(3 + 25) but larger f d allows But let’s not bother. 25) 2 in Z = 611. better parameter selection. Say we have a squa = 611. Pick ¸ ∈ C , root of f . Q ( i;j ) ∈ S ( i − j m )( Then f d ¸ is a root of find factor. in Q ( ¸ ); now what? monic g = f d − 1 f ( x=f d ) ∈ Z [ x ]. d f d ¸ �→ f d m Q ( ¸ ) ←O← Z [ f d ¸ ] − − − − − − − → Z =n
17 18 Generalize from ( x 2 − 14 ; 25) Build square in Q ( ¸ ) from to ( f ; m ) with irred f ∈ Z [ x ], congruences ( i − j m )( i − j ¸ rphism m ∈ Z , f ( m ) ∈ n Z . with i Z + j Z = Z and j > 0. 25, Write d = deg f , Could replace i − j x by f = f d x d + · · · + f 1 x 1 + f 0 x 0 . higher-deg irred in Z [ x ]; square: quadratics seem fairly small 25) Can take f d = 1 for simplicity, for some number fields. but larger f d allows But let’s not bother. 611. better parameter selection. Say we have a square Pick ¸ ∈ C , root of f . Q ( i;j ) ∈ S ( i − j m )( i − j ¸ ) Then f d ¸ is a root of r. in Q ( ¸ ); now what? monic g = f d − 1 f ( x=f d ) ∈ Z [ x ]. d f d ¸ �→ f d m Q ( ¸ ) ←O← Z [ f d ¸ ] − − − − − − − → Z =n
18 19 Generalize from ( x 2 − 14 ; 25) Build square in Q ( ¸ ) from to ( f ; m ) with irred f ∈ Z [ x ], congruences ( i − j m )( i − j ¸ ) m ∈ Z , f ( m ) ∈ n Z . with i Z + j Z = Z and j > 0. Write d = deg f , Could replace i − j x by f = f d x d + · · · + f 1 x 1 + f 0 x 0 . higher-deg irred in Z [ x ]; quadratics seem fairly small Can take f d = 1 for simplicity, for some number fields. but larger f d allows But let’s not bother. better parameter selection. Say we have a square Pick ¸ ∈ C , root of f . Q ( i;j ) ∈ S ( i − j m )( i − j ¸ ) Then f d ¸ is a root of in Q ( ¸ ); now what? monic g = f d − 1 f ( x=f d ) ∈ Z [ x ]. d f d ¸ �→ f d m Q ( ¸ ) ←O← Z [ f d ¸ ] − − − − − − − → Z =n
18 19 Generalize from ( x 2 − 14 ; 25) Q ( i − j m Build square in Q ( ¸ ) from m ) with irred f ∈ Z [ x ], congruences ( i − j m )( i − j ¸ ) is a squa , f ( m ) ∈ n Z . with i Z + j Z = Z and j > 0. ring of in d = deg f , Could replace i − j x by Multiply x d + · · · + f 1 x 1 + f 0 x 0 . higher-deg irred in Z [ x ]; putting squa quadratics seem fairly small compute take f d = 1 for simplicity, Q ( i − j m for some number fields. rger f d allows But let’s not bother. parameter selection. Then apply Say we have a square ’ : Z [ f d ¸ ∈ C , root of f . Q ( i;j ) ∈ S ( i − j m )( i − j ¸ ) f d ¸ to f f d ¸ is a root of in Q ( ¸ ); now what? ’ ( r ) − g g = f d − 1 f ( x=f d ) ∈ Z [ x ]. d In Z =n have f d ¸ �→ f d m g ′ ( f d m ) 2 ←O← Z [ f d ¸ ] − − − − − − − → Z =n
18 19 ( x 2 − 14 ; 25) Q ( i − j m )( i − j ¸ Build square in Q ( ¸ ) from irred f ∈ Z [ x ], congruences ( i − j m )( i − j ¸ ) is a square in O , n Z . with i Z + j Z = Z and j > 0. ring of integers of Multiply by g ′ ( f d ¸ , Could replace i − j x by f 1 x 1 + f 0 x 0 . higher-deg irred in Z [ x ]; putting square root compute r with r 2 quadratics seem fairly small for simplicity, Q ( i − j m )( i − j ¸ for some number fields. allows But let’s not bother. rameter selection. Then apply the ring ’ : Z [ f d ¸ ] → Z =n Say we have a square ot of f . Q ( i;j ) ∈ S ( i − j m )( i − j ¸ ) f d ¸ to f d m . Compute ot of ’ ( r ) − g ′ ( f d m ) Q in Q ( ¸ ); now what? f ( x=f d ) ∈ Z [ x ]. In Z =n have ’ ( r ) 2 f d ¸ �→ f d m g ′ ( f d m ) 2 Q ( i − j m ¸ ] − − − − − − − → Z =n
18 19 Q ( i − j m )( i − j ¸ ) f 2 25) Build square in Q ( ¸ ) from d [ x ], congruences ( i − j m )( i − j ¸ ) is a square in O , with i Z + j Z = Z and j > 0. ring of integers of Q ( ¸ ). Multiply by g ′ ( f d ¸ ) 2 , Could replace i − j x by 0 x 0 . higher-deg irred in Z [ x ]; putting square root into Z [ f d compute r with r 2 = g ′ ( f d ¸ quadratics seem fairly small implicity, Q ( i − j m )( i − j ¸ ) f 2 for some number fields. d . But let’s not bother. selection. Then apply the ring morphism ’ : Z [ f d ¸ ] → Z =n taking Say we have a square Q ( i;j ) ∈ S ( i − j m )( i − j ¸ ) f d ¸ to f d m . Compute gcd { ’ ( r ) − g ′ ( f d m ) Q ( i − j m ) f in Q ( ¸ ); now what? Z [ x ]. In Z =n have ’ ( r ) 2 = m g ′ ( f d m ) 2 Q ( i − j m ) 2 f 2 d . − → Z =n
19 20 Q ( i − j m )( i − j ¸ ) f 2 Build square in Q ( ¸ ) from d congruences ( i − j m )( i − j ¸ ) is a square in O , with i Z + j Z = Z and j > 0. ring of integers of Q ( ¸ ). Multiply by g ′ ( f d ¸ ) 2 , Could replace i − j x by higher-deg irred in Z [ x ]; putting square root into Z [ f d ¸ ]: compute r with r 2 = g ′ ( f d ¸ ) 2 · quadratics seem fairly small Q ( i − j m )( i − j ¸ ) f 2 for some number fields. d . But let’s not bother. Then apply the ring morphism ’ : Z [ f d ¸ ] → Z =n taking Say we have a square Q ( i;j ) ∈ S ( i − j m )( i − j ¸ ) f d ¸ to f d m . Compute gcd { n; ’ ( r ) − g ′ ( f d m ) Q ( i − j m ) f d } . in Q ( ¸ ); now what? In Z =n have ’ ( r ) 2 = g ′ ( f d m ) 2 Q ( i − j m ) 2 f 2 d .
19 20 Q ( i − j m )( i − j ¸ ) f 2 square in Q ( ¸ ) from How to find d congruences ( i − j m )( i − j ¸ ) is a square in O , of congruences Z + j Z = Z and j > 0. ring of integers of Q ( ¸ ). Start with e.g., y 2 pairs Multiply by g ′ ( f d ¸ ) 2 , replace i − j x by higher-deg irred in Z [ x ]; putting square root into Z [ f d ¸ ]: Look for compute r with r 2 = g ′ ( f d ¸ ) 2 · quadratics seem fairly small y -smooth Q ( i − j m )( i − j ¸ ) f 2 ome number fields. d . y -smooth let’s not bother. f d i d + · Then apply the ring morphism ’ : Z [ f d ¸ ] → Z =n taking e have a square Here “ y -smo S ( i − j m )( i − j ¸ ) f d ¸ to f d m . Compute gcd { n; “has no ’ ( r ) − g ′ ( f d m ) Q ( i − j m ) f d } . ); now what? Find enough In Z =n have ’ ( r ) 2 = Perform g ′ ( f d m ) 2 Q ( i − j m ) 2 f 2 d . exponent
19 20 Q ( i − j m )( i − j ¸ ) f 2 Q ( ¸ ) from How to find square d j m )( i − j ¸ ) is a square in O , of congruences ( i − Z and j > 0. ring of integers of Q ( ¸ ). Start with congruences e.g., y 2 pairs ( i; j ). Multiply by g ′ ( f d ¸ ) 2 , − j x by in Z [ x ]; putting square root into Z [ f d ¸ ]: Look for y -smooth compute r with r 2 = g ′ ( f d ¸ ) 2 · fairly small y -smooth i − j m Q ( i − j m )( i − j ¸ ) f 2 er fields. d . y -smooth f d norm( other. f d i d + · · · + f 0 j d = Then apply the ring morphism ’ : Z [ f d ¸ ] → Z =n taking square Here “ y -smooth” means )( i − j ¸ ) f d ¸ to f d m . Compute gcd { n; “has no prime diviso ’ ( r ) − g ′ ( f d m ) Q ( i − j m ) f d } . what? Find enough smooth In Z =n have ’ ( r ) 2 = Perform linear algeb g ′ ( f d m ) 2 Q ( i − j m ) 2 f 2 d . exponent vectors mo
19 20 Q ( i − j m )( i − j ¸ ) f 2 How to find square product d j ¸ ) is a square in O , of congruences ( i − j m )( i − 0. ring of integers of Q ( ¸ ). Start with congruences for, e.g., y 2 pairs ( i; j ). Multiply by g ′ ( f d ¸ ) 2 , putting square root into Z [ f d ¸ ]: Look for y -smooth congruences: compute r with r 2 = g ′ ( f d ¸ ) 2 · small y -smooth i − j m and Q ( i − j m )( i − j ¸ ) f 2 d . y -smooth f d norm( i − j ¸ ) = f d i d + · · · + f 0 j d = j d f ( i=j Then apply the ring morphism ’ : Z [ f d ¸ ] → Z =n taking Here “ y -smooth” means f d ¸ to f d m . Compute gcd { n; “has no prime divisor > y .” ’ ( r ) − g ′ ( f d m ) Q ( i − j m ) f d } . Find enough smooth congruences. In Z =n have ’ ( r ) 2 = Perform linear algebra on g ′ ( f d m ) 2 Q ( i − j m ) 2 f 2 d . exponent vectors mod 2.
20 21 Q ( i − j m )( i − j ¸ ) f 2 How to find square product d is a square in O , of congruences ( i − j m )( i − j ¸ )? ring of integers of Q ( ¸ ). Start with congruences for, e.g., y 2 pairs ( i; j ). Multiply by g ′ ( f d ¸ ) 2 , putting square root into Z [ f d ¸ ]: Look for y -smooth congruences: compute r with r 2 = g ′ ( f d ¸ ) 2 · y -smooth i − j m and Q ( i − j m )( i − j ¸ ) f 2 d . y -smooth f d norm( i − j ¸ ) = f d i d + · · · + f 0 j d = j d f ( i=j ). Then apply the ring morphism ’ : Z [ f d ¸ ] → Z =n taking Here “ y -smooth” means f d ¸ to f d m . Compute gcd { n; “has no prime divisor > y .” ’ ( r ) − g ′ ( f d m ) Q ( i − j m ) f d } . Find enough smooth congruences. In Z =n have ’ ( r ) 2 = Perform linear algebra on g ′ ( f d m ) 2 Q ( i − j m ) 2 f 2 d . exponent vectors mod 2.
20 21 j m )( i − j ¸ ) f 2 How to find square product Asymptotic d square in O , of congruences ( i − j m )( i − j ¸ )? Number of integers of Q ( ¸ ). Start with congruences for, in number-field e.g., y 2 pairs ( i; j ). Multiply by g ′ ( f d ¸ ) 2 , with theo is L 1 : 90 ::: putting square root into Z [ f d ¸ ]: Look for y -smooth congruences: compute r with r 2 = g ′ ( f d ¸ ) 2 · exp((log y -smooth i − j m and j m )( i − j ¸ ) f 2 d . y -smooth f d norm( i − j ¸ ) = What are f d i d + · · · + f 0 j d = j d f ( i=j ). apply the ring morphism Choose degree d ¸ ] → Z =n taking Here “ y -smooth” means d= (log n ) f d m . Compute gcd { n; “has no prime divisor > y .” ∈ 1 : 40 : : g ′ ( f d m ) Q ( i − j m ) f d } . Find enough smooth congruences. have ’ ( r ) 2 = Perform linear algebra on ) 2 Q ( i − j m ) 2 f 2 d . exponent vectors mod 2.
20 21 j ¸ ) f 2 How to find square product Asymptotic cost exp d , of congruences ( i − j m )( i − j ¸ )? Number of bit operations of Q ( ¸ ). Start with congruences for, in number-field sieve e.g., y 2 pairs ( i; j ). ¸ ) 2 , with theorists’ parameters, is L 1 : 90 ::: + o (1) where root into Z [ f d ¸ ]: Look for y -smooth congruences: r 2 = g ′ ( f d ¸ ) 2 · exp((log n ) 1 = 3 (log y -smooth i − j m and j ¸ ) f 2 d . y -smooth f d norm( i − j ¸ ) = What are theorists’ f d i d + · · · + f 0 j d = j d f ( i=j ). ring morphism Choose degree d with =n taking Here “ y -smooth” means d= (log n ) 1 = 3 (log log Compute gcd { n; “has no prime divisor > y .” ∈ 1 : 40 : : : + o (1). Q ( i − j m ) f d } . Find enough smooth congruences. ) 2 = Perform linear algebra on j m ) 2 f 2 d . exponent vectors mod 2.
20 21 How to find square product Asymptotic cost exponents of congruences ( i − j m )( i − j ¸ )? Number of bit operations Start with congruences for, in number-field sieve, e.g., y 2 pairs ( i; j ). with theorists’ parameters, is L 1 : 90 ::: + o (1) where L = [ f d ¸ ]: Look for y -smooth congruences: ¸ ) 2 · exp((log n ) 1 = 3 (log log n ) 2 = 3 ). y -smooth i − j m and y -smooth f d norm( i − j ¸ ) = What are theorists’ paramete f d i d + · · · + f 0 j d = j d f ( i=j ). rphism Choose degree d with Here “ y -smooth” means d= (log n ) 1 = 3 (log log n ) − 1 = 3 gcd { n; “has no prime divisor > y .” ∈ 1 : 40 : : : + o (1). ) f d } . Find enough smooth congruences. Perform linear algebra on exponent vectors mod 2.
21 22 How to find square product Asymptotic cost exponents of congruences ( i − j m )( i − j ¸ )? Number of bit operations Start with congruences for, in number-field sieve, e.g., y 2 pairs ( i; j ). with theorists’ parameters, is L 1 : 90 ::: + o (1) where L = Look for y -smooth congruences: exp((log n ) 1 = 3 (log log n ) 2 = 3 ). y -smooth i − j m and y -smooth f d norm( i − j ¸ ) = What are theorists’ parameters? f d i d + · · · + f 0 j d = j d f ( i=j ). Choose degree d with Here “ y -smooth” means d= (log n ) 1 = 3 (log log n ) − 1 = 3 “has no prime divisor > y .” ∈ 1 : 40 : : : + o (1). Find enough smooth congruences. Perform linear algebra on exponent vectors mod 2.
21 22 to find square product Asymptotic cost exponents Choose integer congruences ( i − j m )( i − j ¸ )? Write n Number of bit operations m d + f d ith congruences for, in number-field sieve, with each 2 pairs ( i; j ). with theorists’ parameters, Choose f is L 1 : 90 ::: + o (1) where L = for y -smooth congruences: in case there exp((log n ) 1 = 3 (log log n ) 2 = 3 ). oth i − j m and Test smo oth f d norm( i − j ¸ ) = What are theorists’ parameters? for all cop · · · + f 0 j d = j d f ( i=j ). Choose degree d with with 1 ≤ y -smooth” means d= (log n ) 1 = 3 (log log n ) − 1 = 3 using prime no prime divisor > y .” ∈ 1 : 40 : : : + o (1). L 1 : 90 ::: + o enough smooth congruences. Conjecturally rm linear algebra on smooth values onent vectors mod 2.
21 22 square product Asymptotic cost exponents Choose integer m i − j m )( i − j ¸ )? Write n as Number of bit operations m d + f d − 1 m d − 1 + congruences for, in number-field sieve, with each f k below j ). with theorists’ parameters, Choose f with some is L 1 : 90 ::: + o (1) where L = oth congruences: in case there are b exp((log n ) 1 = 3 (log log n ) 2 = 3 ). and Test smoothness of rm( i − j ¸ ) = What are theorists’ parameters? for all coprime pairs d = j d f ( i=j ). Choose degree d with with 1 ≤ i; j ≤ L 0 : oth” means d= (log n ) 1 = 3 (log log n ) − 1 = 3 using primes ≤ L 0 : 95 divisor > y .” ∈ 1 : 40 : : : + o (1). L 1 : 90 ::: + o (1) pairs. smooth congruences. Conjecturally L 1 : 65 algebra on smooth values of i rs mod 2.
21 22 Choose integer m ≈ n 1 =d . duct Asymptotic cost exponents − j ¸ )? Write n as Number of bit operations m d + f d − 1 m d − 1 + · · · + f 1 m r, in number-field sieve, with each f k below n (1+ o (1)) with theorists’ parameters, Choose f with some randomness is L 1 : 90 ::: + o (1) where L = congruences: in case there are bad f ’s. exp((log n ) 1 = 3 (log log n ) 2 = 3 ). Test smoothness of i − j m = What are theorists’ parameters? for all coprime pairs ( i; j ) i=j ). Choose degree d with with 1 ≤ i; j ≤ L 0 : 95 ::: + o (1) , d= (log n ) 1 = 3 (log log n ) − 1 = 3 using primes ≤ L 0 : 95 ::: + o (1) . .” ∈ 1 : 40 : : : + o (1). L 1 : 90 ::: + o (1) pairs. congruences. Conjecturally L 1 : 65 ::: + o (1) smooth values of i − j m .
22 23 Choose integer m ≈ n 1 =d . Asymptotic cost exponents Write n as Number of bit operations m d + f d − 1 m d − 1 + · · · + f 1 m + f 0 in number-field sieve, with each f k below n (1+ o (1)) =d . with theorists’ parameters, Choose f with some randomness is L 1 : 90 ::: + o (1) where L = in case there are bad f ’s. exp((log n ) 1 = 3 (log log n ) 2 = 3 ). Test smoothness of i − j m What are theorists’ parameters? for all coprime pairs ( i; j ) Choose degree d with with 1 ≤ i; j ≤ L 0 : 95 ::: + o (1) , d= (log n ) 1 = 3 (log log n ) − 1 = 3 using primes ≤ L 0 : 95 ::: + o (1) . ∈ 1 : 40 : : : + o (1). L 1 : 90 ::: + o (1) pairs. Conjecturally L 1 : 65 ::: + o (1) smooth values of i − j m .
22 23 Choose integer m ≈ n 1 =d . Use L 0 : 12 Asymptotic cost exponents Write n as er of bit operations For each m d + f d − 1 m d − 1 + · · · + f 1 m + f 0 number-field sieve, with smo with each f k below n (1+ o (1)) =d . theorists’ parameters, test smo Choose f with some randomness ::: + o (1) where L = and i − j in case there are bad f ’s. exp((log n ) 1 = 3 (log log n ) 2 = 3 ). using prime Test smoothness of i − j m L 1 : 77 ::: + o are theorists’ parameters? for all coprime pairs ( i; j ) Each | j d ose degree d with with 1 ≤ i; j ≤ L 0 : 95 ::: + o (1) , Conjecturally n ) 1 = 3 (log log n ) − 1 = 3 using primes ≤ L 0 : 95 ::: + o (1) . smooth congruences. : : : + o (1). L 1 : 90 ::: + o (1) pairs. L 0 : 95 ::: + o Conjecturally L 1 : 65 ::: + o (1) in the exp smooth values of i − j m .
22 23 Use L 0 : 12 ::: + o (1) numb Choose integer m ≈ n 1 =d . exponents Write n as operations For each ( i; j ) m d + f d − 1 m d − 1 + · · · + f 1 m + f 0 sieve, with smooth i − j m with each f k below n (1+ o (1)) =d . parameters, test smoothness of Choose f with some randomness where L = and i − j ˛ and so in case there are bad f ’s. (log log n ) 2 = 3 ). using primes ≤ L 0 : 82 Test smoothness of i − j m L 1 : 77 ::: + o (1) tests. rists’ parameters? for all coprime pairs ( i; j ) Each | j d f ( i=j ) | ≤ with with 1 ≤ i; j ≤ L 0 : 95 ::: + o (1) , Conjecturally L 0 : 95 log n ) − 1 = 3 using primes ≤ L 0 : 95 ::: + o (1) . smooth congruences. (1). L 1 : 90 ::: + o (1) pairs. L 0 : 95 ::: + o (1) components Conjecturally L 1 : 65 ::: + o (1) in the exponent vecto smooth values of i − j m .
22 23 Use L 0 : 12 ::: + o (1) number fields. Choose integer m ≈ n 1 =d . onents Write n as For each ( i; j ) m d + f d − 1 m d − 1 + · · · + f 1 m + f 0 with smooth i − j m , with each f k below n (1+ o (1)) =d . rameters, test smoothness of i − j ¸ Choose f with some randomness and i − j ˛ and so on, in case there are bad f ’s. 3 ). using primes ≤ L 0 : 82 ::: + o (1) . Test smoothness of i − j m L 1 : 77 ::: + o (1) tests. rameters? for all coprime pairs ( i; j ) Each | j d f ( i=j ) | ≤ m 2 : 86 ::: + o with 1 ≤ i; j ≤ L 0 : 95 ::: + o (1) , Conjecturally L 0 : 95 ::: + o (1) using primes ≤ L 0 : 95 ::: + o (1) . smooth congruences. L 1 : 90 ::: + o (1) pairs. L 0 : 95 ::: + o (1) components Conjecturally L 1 : 65 ::: + o (1) in the exponent vectors. smooth values of i − j m .
23 24 Use L 0 : 12 ::: + o (1) number fields. Choose integer m ≈ n 1 =d . Write n as For each ( i; j ) m d + f d − 1 m d − 1 + · · · + f 1 m + f 0 with smooth i − j m , with each f k below n (1+ o (1)) =d . test smoothness of i − j ¸ Choose f with some randomness and i − j ˛ and so on, in case there are bad f ’s. using primes ≤ L 0 : 82 ::: + o (1) . Test smoothness of i − j m L 1 : 77 ::: + o (1) tests. for all coprime pairs ( i; j ) Each | j d f ( i=j ) | ≤ m 2 : 86 ::: + o (1) . with 1 ≤ i; j ≤ L 0 : 95 ::: + o (1) , Conjecturally L 0 : 95 ::: + o (1) using primes ≤ L 0 : 95 ::: + o (1) . smooth congruences. L 1 : 90 ::: + o (1) pairs. L 0 : 95 ::: + o (1) components Conjecturally L 1 : 65 ::: + o (1) in the exponent vectors. smooth values of i − j m .
23 24 Use L 0 : 12 ::: + o (1) number fields. ose integer m ≈ n 1 =d . Three sizes n as (log n ) 1 = For each ( i; j ) f d − 1 m d − 1 + · · · + f 1 m + f 0 with smooth i − j m , y , i , j . each f k below n (1+ o (1)) =d . test smoothness of i − j ¸ (log n ) 2 = ose f with some randomness and i − j ˛ and so on, m , i − j there are bad f ’s. using primes ≤ L 0 : 82 ::: + o (1) . log n bits: smoothness of i − j m L 1 : 77 ::: + o (1) tests. coprime pairs ( i; j ) Each | j d f ( i=j ) | ≤ m 2 : 86 ::: + o (1) . Unavoidably ≤ i; j ≤ L 0 : 95 ::: + o (1) , Conjecturally L 0 : 95 ::: + o (1) usual smo primes ≤ L 0 : 95 ::: + o (1) . forces (log smooth congruences. + o (1) pairs. balancing L 0 : 95 ::: + o (1) components Conjecturally L 1 : 65 ::: + o (1) forces d log in the exponent vectors. and d log oth values of i − j m .
23 24 Use L 0 : 12 ::: + o (1) number fields. ≈ n 1 =d . Three sizes of numb (log n ) 1 = 3 (log log n For each ( i; j ) + · · · + f 1 m + f 0 with smooth i − j m , y , i , j . elow n (1+ o (1)) =d . test smoothness of i − j ¸ (log n ) 2 = 3 (log log n some randomness and i − j ˛ and so on, m , i − j m , j d f ( i=j bad f ’s. using primes ≤ L 0 : 82 ::: + o (1) . log n bits: n . of i − j m L 1 : 77 ::: + o (1) tests. pairs ( i; j ) Each | j d f ( i=j ) | ≤ m 2 : 86 ::: + o (1) . Unavoidably 1 = 3 in 0 : 95 ::: + o (1) , Conjecturally L 0 : 95 ::: + o (1) usual smoothness optim 0 : 95 ::: + o (1) . forces (log y ) 2 ≈ log smooth congruences. balancing norms with pairs. L 0 : 95 ::: + o (1) components 65 ::: + o (1) forces d log y ≈ log in the exponent vectors. and d log m ≈ log n of i − j m .
23 24 Use L 0 : 12 ::: + o (1) number fields. Three sizes of numbers here: (log n ) 1 = 3 (log log n ) 2 = 3 bits: For each ( i; j ) 1 m + f 0 with smooth i − j m , y , i , j . (1)) =d . test smoothness of i − j ¸ (log n ) 2 = 3 (log log n ) 1 = 3 bits: randomness and i − j ˛ and so on, m , i − j m , j d f ( i=j ). using primes ≤ L 0 : 82 ::: + o (1) . log n bits: n . L 1 : 77 ::: + o (1) tests. Each | j d f ( i=j ) | ≤ m 2 : 86 ::: + o (1) . Unavoidably 1 = 3 in exponent: (1) , Conjecturally L 0 : 95 ::: + o (1) usual smoothness optimization . forces (log y ) 2 ≈ log m ; smooth congruences. balancing norms with m L 0 : 95 ::: + o (1) components forces d log y ≈ log m ; in the exponent vectors. and d log m ≈ log n .
24 25 Use L 0 : 12 ::: + o (1) number fields. Three sizes of numbers here: (log n ) 1 = 3 (log log n ) 2 = 3 bits: For each ( i; j ) with smooth i − j m , y , i , j . test smoothness of i − j ¸ (log n ) 2 = 3 (log log n ) 1 = 3 bits: and i − j ˛ and so on, m , i − j m , j d f ( i=j ). using primes ≤ L 0 : 82 ::: + o (1) . log n bits: n . L 1 : 77 ::: + o (1) tests. Each | j d f ( i=j ) | ≤ m 2 : 86 ::: + o (1) . Unavoidably 1 = 3 in exponent: Conjecturally L 0 : 95 ::: + o (1) usual smoothness optimization forces (log y ) 2 ≈ log m ; smooth congruences. balancing norms with m L 0 : 95 ::: + o (1) components forces d log y ≈ log m ; in the exponent vectors. and d log m ≈ log n .
24 25 : 12 ::: + o (1) number fields. Three sizes of numbers here: Batch NFS (log n ) 1 = 3 (log log n ) 2 = 3 bits: each ( i; j ) The numb L 1 : 90 ::: + o smooth i − j m , y , i , j . oothness of i − j ¸ finding smo (log n ) 2 = 3 (log log n ) 1 = 3 bits: L 1 : 77 ::: + o − j ˛ and so on, m , i − j m , j d f ( i=j ). primes ≤ L 0 : 82 ::: + o (1) . finding smo log n bits: n . + o (1) tests. Many n ’s | j d f ( i=j ) | ≤ m 2 : 86 ::: + o (1) . L 1 : 90 ::: + o Unavoidably 1 = 3 in exponent: Conjecturally L 0 : 95 ::: + o (1) usual smoothness optimization to find squa forces (log y ) 2 ≈ log m ; oth congruences. Oops, linea balancing norms with m + o (1) components fix by reducing forces d log y ≈ log m ; exponent vectors. But still and d log m ≈ log n . batch in factoring
Recommend
More recommend