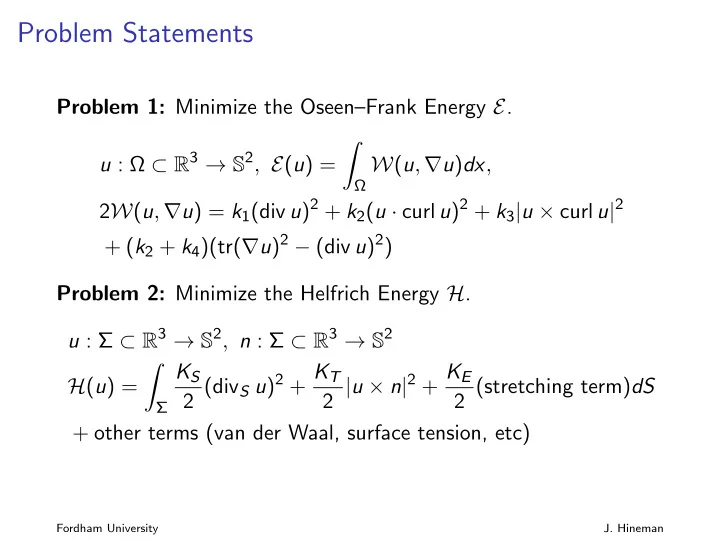
Problem Statements Problem 1: Minimize the OseenFrank Energy E . u - PowerPoint PPT Presentation

Problem Statements Problem 1: Minimize the Oseen–Frank Energy E . � u : Ω ⊂ R 3 → S 2 , E ( u ) = W ( u , ∇ u ) dx , Ω 2 W ( u , ∇ u ) = k 1 (div u ) 2 + k 2 ( u · curl u ) 2 + k 3 | u × curl u | 2 + ( k 2 + k 4 )(tr( ∇ u ) 2 − (div u ) 2 ) Problem 2: Minimize the Helfrich Energy H . u : Σ ⊂ R 3 → S 2 , n : Σ ⊂ R 3 → S 2 K S 2 (div S u ) 2 + K T 2 | u × n | 2 + K E � H ( u ) = 2 (stretching term) dS Σ + other terms (van der Waal, surface tension, etc) Fordham University J. Hineman

Present Work One-constant approximation: W HM ( u , ∇ u ) = K |∇ u | 2 ; W HM is 2 the harmonic map energy density. Alouges Algorithm: F. Alouges 1994, F. Alouges, J.M. Ghidaglia 1997, S. Bartel 2005 ◮ Initialize: choose u (0) in H 1 (Ω , S 2 ) ◮ Minimize: Compute w ( j ) ∈ H 1 (Ω , R 3 ) to satisfy E HM ( u ( j ) − w ( j ) ) ≤ E HM ( u ( j ) − v ) , ∀ v ∈ H 1 (Ω , R 3 ) ◮ Project: set u ( j +1) = u ( j ) − w ( j ) | u ( j ) − w ( j ) | Direct Minimization: R. Ryham et al 2013, 2015 ◮ min µ � x − x (0) � + 2∆ tH ( x ) ◮ Gradient descent µ ( x − x (0) ) = − ∆ t ∇ x H ( x ) ◮ Fully-implicit backwards Euler (solved efficiently via Newton iteration) x H ( x ) + µ ) δ x = µ ( x (0) − x ) − ∆ t ∇ x H ( x ) , x ← (∆ t ∇ 2 − x + δ x (1) Fordham University J. Hineman

Open Problems 1. Does finite element procedure of Bartels adapt to quads/hexes for W HM ? As written, a regular acute triangular mesh is required. 2. Minimization of E . There are few numerical results treat the full energy. 3. Complete description of (nematic) liquid crystals requires a coupled Navier–Stokes/Transported heat flow of harmonic maps system. There are few results using full Oseen–Frank energy and full constitutive relations. 4. Implement iterations like (1). This would be especially useful for the most general problem which allow the surface Σ to evolve (say using phase-field). So far, our simulations are axisymmetric. 4.1 Full disclosure: really, non-equilibrium problem, and thus requires a minimum energy path formulation = ⇒ string method = ⇒ parallelism. 4.2 Automatic mesh refinement probably a necessity for 3d. Fordham University J. Hineman