owl
play

OWL Three species of OWL OWL full is union of OWL syntax and RDF - PowerPoint PPT Presentation

Dec 06, 2022 •219 likes •967 views

OWL Three species of OWL OWL full is union of OWL syntax and RDF (Undecidable) OWL DL restricted to FOL fragment (decidable in NEXPTIME) OWL Lite is easier to implement subset of OWL DL (decidable in EXPTIME) Semantic

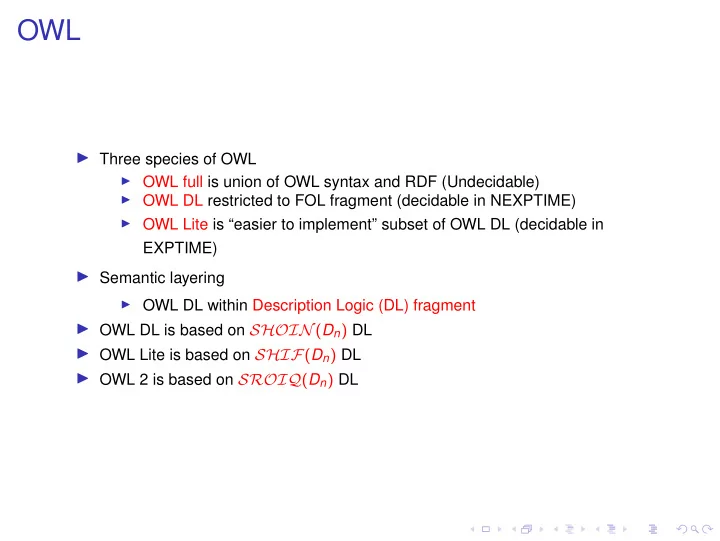
  1. OWL ◮ Three species of OWL ◮ OWL full is union of OWL syntax and RDF (Undecidable) ◮ OWL DL restricted to FOL fragment (decidable in NEXPTIME) ◮ OWL Lite is “easier to implement” subset of OWL DL (decidable in EXPTIME) ◮ Semantic layering ◮ OWL DL within Description Logic (DL) fragment ◮ OWL DL is based on SHOIN ( D n ) DL ◮ OWL Lite is based on SHIF ( D n ) DL ◮ OWL 2 is based on SROIQ ( D n ) DL

  2. Description Logics (DLs) ◮ The logics behind OWL-DL and OWL-Lite, http://dl.kr.org/ . ◮ Concept/Class: names are equivalent to unary predicates ◮ In general, concepts equiv to formulae with one free variable ◮ Role or attribute: names are equivalent to binary predicates ◮ In general, roles equiv to formulae with two free variables ◮ Taxonomy: Concept and role hierarchies can be expressed ◮ Individual: names are equivalent to constants ◮ Operators: restricted so that: ◮ Language is decidable and, if possible, of low complexity ◮ No need for explicit use of variables ◮ Restricted form of ∃ and ∀ ◮ Features such as counting can be succinctly expressed

  3. The DL Family ◮ A given DL is defined by set of concept and role forming operators ◮ Basic language: ALC ( A ttributive L anguage with C omplement) Syntax Semantics Example C , D → ⊤ | ⊤ ( x ) ⊥ | ⊥ ( x ) A | A ( x ) Human C ⊓ D | C ( x ) ∧ D ( x ) Human ⊓ Male C ⊔ D | C ( x ) ∨ D ( x ) Nice ⊔ Rich ¬ C | ¬ C ( x ) ¬ Meat ∃ R . C | ∃ y . R ( x , y ) ∧ C ( y ) ∃ has _ child . Blond ∀ R . C ∀ y . R ( x , y ) ⇒ C ( y ) ∀ has _ child . Human C ⊑ D ∀ x . C ( x ) ⇒ D ( x ) Happy _ Father ⊑ Man ⊓ ∃ has _ child . Female a : C C ( a ) John : Happy _ Father

  4. Toy Example Sex = Male ⊔ Female Male ⊓ Female ⊑ ⊥ Person ⊑ Human ⊓ ∃ hasSex . Sex MalePerson = Person ⊓ ∃ hasSex . Male functional ( hasSex ) umberto : Person ⊓ ∃ hasSex . ¬ Female KB | = umberto : MalePerson

  5. Note on DL Naming AL : − → ⊤ | ⊥ | A | C ⊓ D | ¬ A | ∃ R . ⊤ |∀ R . C C , D C : Concept negation, ¬ C . Thus, ALC = AL + C S : Used for ALC with transitive roles R + U : Concept disjunction, C 1 ⊔ C 2 E : Existential quantification, ∃ R . C H : Role inclusion axioms, R 1 ⊑ R 2 , e.g. is _ component _ of ⊑ is _ part _ of N : Number restrictions, ( ≥ n R ) and ( ≤ n R ) , e.g. ( ≥ 3 has _ Child ) (has at least 3 children) Q : Qualified number restrictions, ( ≥ n R . C ) and ( ≤ n R . C ) , e.g. ( ≤ 2 has _ Child . Adult ) (has at most 2 adult children) O : Nominals (singleton class), { a } , e.g. ∃ has _ child . { mary } . Note : a : C equiv to { a } ⊑ C and ( a , b ) : R equiv to { a } ⊑ ∃ R . { b } I : Inverse role, R − , e.g. isPartOf = hasPart − F : Functional role, f , e.g. functional ( hasAge ) R + : transitive role, e.g. transitive ( isPartOf ) For instance, SHIF = S + H + I + F = ALCR + HIF OWL-Lite SHOIN = S + H + O + I + N = ALCR + HOIN OWL-DL SROIQ = S + R + O + I + Q = ALCR + ROIN OWL 2

  6. Semantics of Additional Constructs = R 1 ⊑ R 2 iff R 1 I ⊆ R 1 I H : Role inclusion axioms, I | N : Number restrictions, ( ≥ n R ) I = { x ∈ ∆ I : |{ y | � x , y � ∈ R I }| ≥ n } , ( ≤ n R ) I = { x ∈ ∆ I : |{ y | � x , y � ∈ R I }| ≤ n } Q : Qualified number restrictions, ( ≥ n R . C ) I = { x ∈ |{ y | � x , y � ∈ R I ∧ y ∈ C I }| ≥ n } , ( ≤ n R . C ) I = { x ∈ ∆ I : |{ y | � x , y � ∈ R I ∧ y ∈ C I }| ≤ n } O : Nominals (singleton class), { a } I = { a I } I : Inverse role, ( R − ) I = {� x , y � | � y , x � ∈ R I } = fun ( f ) iff ∀ z ∀ y ∀ z if � x , y � ∈ f I and � x , z � ∈ f I F : Functional role, I | the y = z R + : transitive role, ( R + ) I = {� x , y � | ∃ z such that � x , z � ∈ R I ∧ � z , y � ∈ R I }

  7. Basics on Concrete Domains ◮ Concrete domains: reals, integers, strings, . . . ( tim , 14 ) :hasAge ( sf , “ SoftComputing ”) :hasAcronym ( source 1 , “ ComputerScience ”) :isAbout ( service 2 , “ InformationRetrievalTool ′′ ) :Matches Minor = Person ⊓ ∃ hasAge . ≤ 18 ◮ Semantics: a clean separation between “object” classes and concrete domains ◮ D = � ∆ D , Φ D � ◮ ∆ D is an interpretation domain ◮ Φ D is the set of concrete domain predicates d with a predefined arity n and fixed interpretation d D ⊆ ∆ n D ◮ Concrete properties: R I ⊆ ∆ I × ∆ D ◮ Notation: ( D ) . E.g., ALC ( D ) is ALC + concrete domains

  8. ◮ Example: assume I = (∆ I , · I ) such that ∆ I = { a , b , c , d , e , f , 1 , 2 , 4 , 5 , 8 } Person I = { a , b , c , d } ◮ Consider the following concrete domain with of some unary predicates ( n = 1) over reals ◮ ∆ D = R , ◮ Φ D = { = m , ≥ m , ≤ m , > m , < m | m ∈ R } ◮ the fixed interpretation of the predicates is (= m ) D = { m } ( > m ) D = { k | k > m } ( ≥ m ) D { k | k ≥ m } = ( < m ) D { k | k < m } = ( ≤ m ) D = { k | k ≤ m } Concrete properties: hasAge I ⊆ ∆ I × R ◮ hasAge I = {� a , 9 � , � c , 20 � , � b , 12 �} ◮ What is the interpretation of Person ⊓ ∃ hasAge . ≤ 18 ? ( Person ⊓ ∃ hasAge . ≤ 18 ) I = Person I ∩ { x | ∃ y ∈ R such that � x , y � ∈ hasAge I ∧ y ∈ ( ≤ 18 ) I = { a , b , c , d } ∩ { x | ∃ y . � x , y � ∈ {� a , 9 � , � c , 20 � , � b , 12 �} ∧ y ≤ 18 } = { a , b , c , d } ∩ { a , b } = { a , b }

  9. DL Knowledge Base ◮ A DL Knowledge Base is a pair KB = �T , A� , where ◮ T is a TBox ◮ containing general inclusion axioms of the form C ⊑ D , ◮ concept definitions of the form A = C ◮ primitive concept definitions of the form A ⊑ C ◮ role inclusions of the form R ⊑ P ◮ role equivalence of the form R = P ◮ A is a ABox ◮ containing assertions of the form a : C ◮ containing assertions of the form ( a , b ) : R ◮ containing (in) equality Axioms of the form a = b and a � = b ◮ An interpretation I is a model of KB , written I | = KB iff I | = T and I | = A , where ◮ I | = T ( I is a model of T ) iff I is a model of each element in T ◮ I | = A ( I is a model of A ) iff I is a model of each element in A

  10. OWL DL as Description Logic Concept/Class constructors: Abstract Syntax DL Syntax Example Descriptions ( C ) A (URI reference) A Conference ⊤ owl:Thing ⊥ owl:Nothing intersectionOf ( C 1 C 2 . . . ) C 1 ⊓ C 2 Reference ⊓ Journal unionOf ( C 1 C 2 . . . ) C 1 ⊔ C 2 Organization ⊔ Institution complementOf ( C ) ¬ C ¬ MasterThesis oneOf ( o 1 . . . ) { o 1 , . . . } {"WISE","ISWC",...} ∃ R . C ∃ parts.InCollection restriction ( R someValuesFrom ( C )) ∀ R . C ∀ date.Date restriction ( R allValuesFrom ( C )) ∃ R . { o } ∃ date.{2005} restriction ( R hasValue ( o )) ( ≥ n R ) restriction ( R minCardinality ( n )) ( � 1 location ) ( ≤ n R ) restriction ( R maxCardinality ( n )) ( � 1 publisher ) restriction ( U someValuesFrom ( D )) ∃ U . D ∃ issue.integer restriction ( U allValuesFrom ( D )) ∀ U . D ∀ name.string restriction ( U hasValue ( v )) ∃ U . = v } ∃ series . = ” LNCS ” restriction ( U minCardinality ( n )) ( ≥ n U ) ( � 1 title ) restriction ( U maxCardinality ( n )) ( ≤ n U ) ( � 1 author ) Note: R is an abstract role, while U is a concrete property of arity two.

  11. Axioms: Abstract Syntax DL Syntax Example Axioms Class ( A partial C 1 . . . C n ) A ⊑ C 1 ⊓ . . . ⊓ C n Human ⊑ Animal ⊓ Biped Class ( A complete C 1 . . . C n ) A = C 1 ⊓ . . . ⊓ C n Man = Human ⊓ Male EnumeratedClass ( A o 1 . . . o n ) A = { o 1 } ⊔ . . . ⊔ { o n } RGB = { r } ⊔ { g } ⊔ { b } SubClassOf ( C 1 C 2 ) C 1 ⊑ C 2 EquivalentClasses ( C 1 . . . C n ) C 1 = . . . = C n DisjointClasses ( C 1 . . . C n ) C i ⊓ C j = ⊥ , i � = j Male ⊓ Female ⊑⊥ R ⊑ R i HasDaughter ⊑ hasChild ObjectProperty ( R super ( R 1 ) . . . super ( R n ) ( ≥ 1 R ) ⊑ C i ( ≥ 1 hasChild ) ⊑ Human domain ( C 1 ) . . . domain ( C n ) ⊤ ⊑ ∀ R . C i ⊤ ⊑ ∀ hasChild . Human range ( C 1 ) . . . range ( C n ) R = P − hasChild = hasParent − [ inverseof ( P ) ] R ⊑ R − similar = similar − [ symmetric ] ⊤ ⊑ ( ≤ 1 R ) ⊤ ⊑ ( ≤ 1 hasMother ) [functional] ⊤ ⊑ ( ≤ 1 R − ) [Inversefunctional] [Transitive] ) Tr ( R ) Tr ( ancestor ) SubPropertyOf ( R 1 R 2 ) R 1 ⊑ R 2 EquivalentProperties ( R 1 . . . R n ) R 1 = . . . = R n cost = price AnnotationProperty ( S )

  12. Abstract Syntax DL Syntax Example DatatypeProperty ( U super ( U 1 ) . . . super ( U n ) U ⊑ U i domain ( C 1 ) . . . domain ( C n ) ( ≥ 1 U ) ⊑ C i ( ≥ 1 hasAge ) ⊑ Human range ( D 1 ) . . . range ( D n ) ⊤ ⊑ ∀ U . D i ⊤ ⊑ ∀ hasAge . posInteger [ functional ]) ⊤ ⊑ ( ≤ 1 U ) ⊤ ⊑ ( ≤ 1 hasAge ) SubPropertyOf ( U 1 U 2 ) U 1 ⊑ U 2 hasName ⊑ hasFirstName EquivalentProperties ( U 1 . . . U n ) U 1 = . . . = U n Individuals Individual ( o type ( C 1 ) . . . type ( C n )) o : C i tim : Human value ( R 1 o 1 ) . . . value ( R n o n ) ( o , o i ) : R i ( tim , mary ) : hasChild value ( U 1 v 1 ) . . . value ( U n v n ) ( o , v 1 ) : U i ( tim , 14 ) : hasAge SameIndividual ( o 1 . . . o n ) o 1 = . . . = o n president _ Bush = G . W . Bush o i � = o j , i � = j john � = peter DifferentIndividuals ( o 1 . . . o n ) Symbols Object Property R (URI reference) R hasChild Datatype Property U (URI reference) U hasAge Individual o (URI reference) U tim Data Value v (RDF literal) U “International Conference on Semantic Web”

Recommend

More recommend