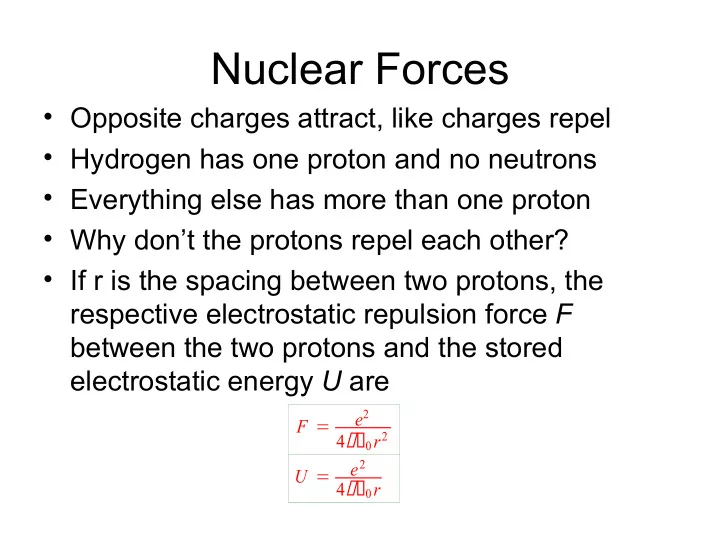
Nuclear Forces Opposite charges attract, like charges repel - PowerPoint PPT Presentation

Nuclear Forces • Opposite charges attract, like charges repel • Hydrogen has one proton and no neutrons • Everything else has more than one proton • Why don’t the protons repel each other? • If r is the spacing between two protons, the respective electrostatic repulsion force F between the two protons and the stored electrostatic energy U are e 2 F  4   0 r 2 e 2 U  4   0 r

What is r ? • It is difficult to determine nuclear size using scattering by charged particles because long range Coloumb force dominates (like trying to determine diameter of sun using orbit of a comet) • Need some head-on collisions • Rutherford shot alpha particles at aluminum and obtained some 180 0 scattering • At instant of direction reversal, the alpha particle must be stationary and all kinetic energy is converted into potential energy • This gives an upper bound for nuclear radius

Estimate upper bound on aluminum nucleus radius based on backscatter of 7.7 MeV alpha particle U  Z  Z Al e 2 4   0 r U  7.7 MeV Z   2 Z Al  13 2  13  1.6  10  19 r  Z  Z Al e 2 4   8.8  10  12  7.7  10 6  4. 9  10  15 m  4   0 U

Determining size of nucleus using neutron scattering • Much easier, since no Coulomb interaction (no electrostatic repulsion) • Simply use   R 1 R 0 nd • Neutrons either hit or miss (no grazing) • These give a nuclear radius scaling as r  r 0 A 1/3 where r 0  1.37  10  15 m

Implications of r  r 0 A 1/3 • Volume of a nucleus scales as A • Densities of all nuclei are about the same • Nuclear mass density is   Am 1 u 1.66043  10  27 3   1.37  10  15  3  1. 5  10 17 kg m  3 3  r 3  4 4 Or more picturesquely, the nuclear density is about 150 thousand metric tons per cubic mm like the mass of an ocean liner crammed into the head of a pin

Nuclear force • Attracts protons and neutrons together • Balances proton electrostatic repulsion • Short range, dies out very quickly beyond nuclear radius • Protons and neutrons in nucleus described by quantum mechanics in manner similar to electrons in an atom • e.g. shell structure, Pauli exclusion principle hold • alpha particle is like a completed K shell (2 protons, 2 neutrons, one each spin up, spin down) and so is stable

Nuclear force as a generic potential well Nucleon trapped In potential well L Nominal dimension

Classical energy 2 mv 2   m v  2 p 2 E  1  2 m 2 m Quantum mechanics: quantize momentum p  k n where k n   / nL Quantized energy for L  2 r 0 E n  p 2 2 m   2  2 n 2 2 8 mr 0  1.05  10  34  2  2  n 2 8  1.6  10  27   2  1.37  10  15  2  1.1  10  12 n 2 Joules  7 n 2 MeV Extremely small size of nucleus implies quantized energy levels are in MeV !!!

Coulomb Barrier • Potential well seen by protons and neutrons differs • Neutrons just see nuclear force (strong force) • Protons also see electrostatic repulsive force due to other protons • Nuclear force is short range, electrostatic force is long range

Coulomb Barrier, cont’d Potential seen by a neutron Neutron outside well feels no force, can wander in Neutron trapped In potential well L Well is due to attractive nuclear force from all other neutrons, protons (short range)

Coulomb Barrier, cont’d Proton outside well does not feel short range nuclear force Ridge (lip) due to repulsive but does feel repulsive electrostatic force is called Coulomb barrier force due to protons inside nucleus Proton trapped In potential well L Nominal dimension Well is due to attractive nuclear force from all other neutrons, protons (short range)

Magnitude of nuclear energy • If nuclear force balances proton repulsion, then stored energy U per pair of protons must be of the order of the electrostatic energy of two protons separated by r 0 e 2 4   0 r 0  1.78  10  13 J  1 MeV U  • Any substantial change in configuration of protons, neutrons in nucleus will generally involve releasing or absorbing energies of the order of MeV

Compare to energy of an electron orbiting in an atom • The distance is of the order of the Bohr radius so the order of magnitude of the energy for an electron orbiting an atom is e 2 U atom  4   0 r Bohr  30 eV • Chemical processes involve rearranging electron orbitals and so are an order of magnitude smaller, a few eV or less

Cross-section can also be used to characterize rate of a process • e. g. can define a cross-section for fission, or a cross-section for neutron capture • Cross-section is usually given in “barns” where 1 barn =10 -24 cm 2 • “like hitting a barn wall”

Reaction-rate cross section • Let R 0 be incident number of particles per second that can instigate a process in target particles having density n in a sheet of thickness d • Let R process be the rate of the process in question • Define the cross-section for the process as  process  R process 1 R 0 nd

Number of protons and neutrons as function of atomic mass • Protons repel each other • Nuclear force is “glue” between protons and neutrons that holds the nucleus together • Use “glue” analogy because glue is a short range force

Electrostatic potential energy In a sphere of charge, Gauss’s law shows that the charge behaves as if it is all at the center. The radial electric field of a sphere of radius r with Z charges is Ze E r  4   0 r 2 The repulsive radial force on each of these charges is Ze 2 F r  4   0 r 2 Here e  1. 6  10  19 is the charge on a proton and  0  8. 854  10  12 is the permittivity of vacuum

The work done per charge on bringing all the charges in from infinity to surface of a sphere of radius r is r U per charge   F r dr  r   Ze 2 4   0 r 2 dr  r Ze 2  4   0 r  Ze 2  4   0 r The work done on all the charges for doing this is U  Z 2 e 2 4   0 r This is the electrostatic potential energy of Z protons grouped together on surface of a sphere of radius r . If charges are uniformly distributed in the volume of the sphere, then find (HW) that U  3 Z 2 e 2 4   0 r 5

Protons on surface of a sphere Z 2 e 2 U  4   0 r Protons filling up volume of a sphere Z 2 e 2 U  3 5 4   0 r

U  3 Z 2 e 2 4   0 r 5 The nuclear radius scaled as r  r 0 A 1/3 and since Z  A /2 the electrostatic potential energy of nuclei scales with A as  A /2  2 e 2 Z 2 e 2 U  3 4   0 r  3 4   0 r 0 A 1/3  A 5/3 5 5 and so the electrostatic energy per nucleon scales as U A  A 2/3 Thus, proportionally more neutrons are required to hold together nuclei with large A

General structure of nuclide chart • Average nuclear force between neutrons and protons is about twice as much as force between two neutrons or two protons • Implies binding energy ought to be largest if there are equal numbers of neutrons and protons • But, when there are lots of protons their mutual electrostatic repulsion becomes important, so need extra neutrons • Large stable nuclides are neutron rich

Nuclide Chart Trends h c h i r c n i r o n t o o r r t P u e N Heavy elements are progressively more neutron rich Z=N

Light nucleii have approximately the same number of neutrons as protons Heavy nuclei have about 1. 5  the number of neutrons as protons Breaking up a heavy nucleus results in neutron-rich light nuclei

Thermal fission A low energy neutron becomes attached to a heavy nuclide having an odd value of A. The nuclide resonates like a jiggled water droplet. It develops a non-spherical shape. The long-range electrostatic repulsion force is only slightly reduced by the non-spherical shape. The short-range attractive nuclear force is greatly reduced by the non-spherical shape. The repulsive electrostatic force wins and the nucleus splits in two (fission). neutron Nucleus with odd A Nucleus with even A

+ + + + + + + + + Vibrating nucleus + + + + + + + + + + + + + + + + + +++++ +++++ +++++ +++++ +++++ +++++ Electrostatic repulsion +++++ +++++ +++++ +++++ +++++ +++++

Uranium fission example First neutron hits U-235 and excites it 236 U  235 U  n  92 92 where the asterisk means excited state (can vibrate) 144 Ba, 36 89 Kr and 3 neutrons Suppose the U-236 splits into 56 Using r  r 0 A 1/3 where r 0  1. 37  10  15 m the radii of the Barium and Krypton are r Ba  1. 37  10  15  144 1/3  7. 18  10  15 m r Kr  1. 37  10  15  89 1/3  6. 12  10  15 m