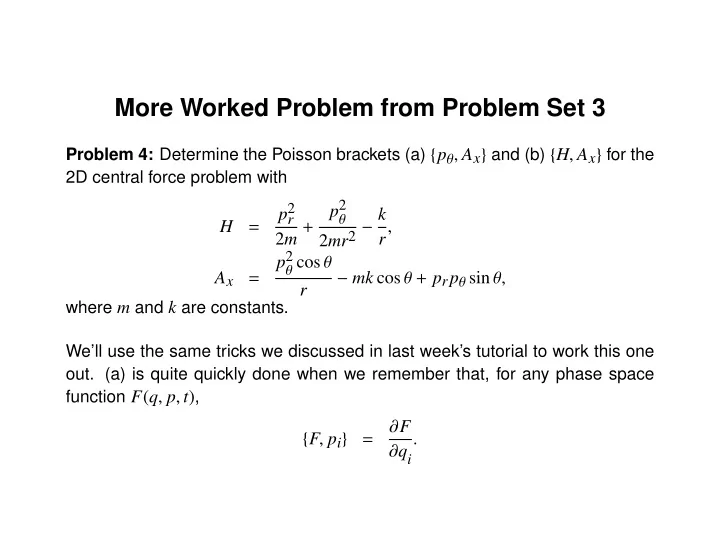
More Worked Problem from Problem Set 3 Problem 4: Determine the - PowerPoint PPT Presentation

More Worked Problem from Problem Set 3 Problem 4: Determine the Poisson brackets (a) { p θ , A x } and (b) { H , A x } for the 2D central force problem with p 2 p 2 2 mr 2 − k θ r r , H = 2 m + p 2 θ cos θ − mk cos θ + p r p θ sin θ, A x = r where m and k are constants. We’ll use the same tricks we discussed in last week’s tutorial to work this one out. (a) is quite quickly done when we remember that, for any phase space function F ( q , p , t ) , ∂ F { F , p i } . = ∂ q i

Thus, p 2   θ cos θ { p θ , A x } = − { A x , p θ } = − ∂ A x − ∂     − mk cos θ + p r p θ sin θ =       ∂θ ∂θ r     p 2 θ sin θ − mk sin θ − p r p θ cos θ = r It’s not stated in the problem, but this is denoted A y and is the y -component of the Runge-Lenz vector referred to in the Note. The other Poisson bracket we want is { H , A x } . This we’ll do using the full defi- nition, so ∂ H ∂ A x + ∂ H ∂ A x − ∂ H ∂ A x ∂ r − ∂ H ∂ A x { H , A x } = ∂ r ∂ p r ∂θ ∂ p θ ∂ p r ∂ p θ ∂θ

which is p 2   � 2 p θ cos θ � mr 3 + k  θ    { H , A x }  ( p θ sin θ ) + (0) + p r sin θ =  −       r 2 r   � p θ p 2 p 2 �   �   θ cos θ θ sin θ � p r         + mk sin θ + p r p θ cos θ −  −  −  −             r 2 mr 2 m r      0 = so we see that A x is a constant of the motion. And since p θ is conserved, then the quantity A y computed in (a) (and thus the full Runge-Lenz vector) is as well by Poisson’s theorem.

Problem 5: The rotation x → x ′ x cos α + y sin α = y → y ′ − x sin α + y cos α (1) = corresponds to a translation θ → θ ′ θ − α (2) = in the polar coordinate system. Prove this, by showing that x ′ and y ′ can be written as x ′ r cos θ ′ = y ′ r sin θ ′ =

This is quite straightforward: using x = r cos θ and y = r sin θ , we have x ′ ( r cos θ ) cos α + ( r sin θ ) sin α = r (cos θ cos α + sin θ sin α ) , = y ′ − ( r cos θ ) x sin α + ( r sin θ ) cos α = r (sin θ cos α − sin α cos θ ) . = Using the famous trig identities cos( A − B ) = cos A cos B + sin A sin B , sin( A − B ) = sin A cos B − sin B cos A , we see x ′ = r cos( θ − α ) = r cos θ ′ and y ′ = r sin( θ − α ) = r sin θ ′ . Thus, this may be rewritten as r cos θ → r cos θ ′ and r sin θ → r sin θ ′ and thus the transformation (1) is equivalent to the transformation r cos θ → r ′ cos θ ′ , r sin θ → r ′ sin θ ′ , r → r ′ = r , θ → θ ′ = θ − α ⇒ as desired.

Problem 6: Using that the Lagrangian for a spherically symmetric potential is m x 2 + ˙ � y 2 � L = ˙ − V ( r ) 2 m r 2 + r 2 ˙ � θ 2 � = ˙ − V ( r ) 2 Problem 5 was grand in and of itself, but it’s here – where we utilise Noether’s amazing theorem – that its significance really kicks in. Recall the definition: we assume that we have a transformation that depends on a continuous variable α , i.e. q i → q ′ i = q i ( α ) . If the Lagrangian does not change under this transfor- mation (a “symmetry” of the Lagrangian), then there is a conserved quantity – the Noether charge associated with this symmetry – given by ∂ q i ( α ) � ∂α . C p i = i

Now to apply it: (a) Compute the noether charge C xy related to the transformation (1). It’s easy to see this Lagrangian is symmetric under this transformation: � � � m x ′ ) 2 + (˙ L ′ � y ′ ) 2 � ( x ′ ) 2 + ( y ′ ) 2 = (˙ − V 2 m y sin α ) 2 + ( − ˙ � y cos α ) 2 � x cos α + ˙ x sin α + ˙ (˙ = 2 � � � ( x cos α + y sin α ) 2 + ( − x sin α + y cos α ) 2 − V � � � m x 2 + ˙ � y 2 � x 2 + y 2 = ˙ − V 2 which is L , so we can apply Noether’s theorem.

The rest of this problem is done in Brian’s notes (page 56) except with α re- placed by − α . If we take that result and switch α to − α , we see that C xy = p x y ( α ) − p y x ( α ) , which we immediately see is − ( � r × � p ) z = − L z . So this is yet another way of deriving AM conservation. (b) Compute the Noether charge C θ related to the transformation (2). Note that since θ ′ = θ − α , ˙ θ ′ = ˙ θ . Thus, under the transformation (2), we get m r ′ ) 2 + ( r ′ ) 2 (˙ L ′ � θ ′ ) 2 � − V ( r ′ ) = (˙ 2 m r 2 + r 2 ˙ � θ 2 � = ˙ − V ( r ) 2 = L so we have a symmetry.

The transformations we need are r ( α ) = r and θ ( α ) = θ − α , so ∂ r ( α ) ∂θ ( α ) C θ = p r + p θ ∂α ∂α = p r · 0 + p θ · ( − 1) − p θ . = So − p θ is constant, which we knew already from the fact that θ is a cyclic coordinate for this this Lagrangian. Surprise, surprise. (c) Show that C xy = C θ . We’ve shown time and time again that p θ = L z , so C xy = − L z and C θ = − p θ = − L z and so C xy and C θ are indeed the same constant.

Problem 8: For a particle with mass m , we know that the angular momentum xy ) in cartesian coordinates ( x , y , z ) and by L z = mr 2 ˙ is given by L z = m ( x ˙ θ y − ˙ in cylinder coordinates ( r , θ, z ) . Thus, (a) For a system of two particles with mass m 1 , m 2 , show that the z -component of the total angular momentum is given by MR 2 ˙ L tot Θ + µ r 2 ˙ θ, = L z 1 + L z 2 = z where ( R , Θ ) and ( r , θ ) are the centre-of-mass and relative coordinates, re- spectively, in cylinder coordinates; M is the total mass of the system, and µ = m 1 m 2 / M is the reduced mass.

This hearkens back to one of the optional problems on the first Problem Set: r 2 , � recall that the relation between � r 1 , � R and � r R = m 1 � r 1 + m 2 � r 2 � , � r = � r 1 − � r 2 , m 1 + m 2 � � � � m 2 m 1 r 1 = � r 2 = � � � r , � � r . R + R − m 1 + m 2 m 1 + m 2 What we do is pick two separate cylindrical coordinate systems for the CoM and relative position vectors, i.e ( R , Θ , Z ) and ( r , θ, z ) respectively. Then we see that the x - and y -coordinates of the two masses may be written as � � x = R cos Θ + µ m 2 r cos θ x 1 = X + m 1 + m 2 m 1 � � y = R sin Θ + µ m 2 r sin θ, y 1 = Y + m 1 + m 2 m 1

� � x = R cos Θ − µ m 1 r cos θ, x 2 = X − m 1 + m 2 m 2 � � y = R sin Θ − µ m 1 r sin θ. y 2 = Y − m 1 + m 2 m 2 From these we can now construct the individual z -components of the AM: L z 1 = m 1 ( x 1 ˙ y 1 − ˙ x 1 y 1 ) � � � �� R cos Θ + µ Θ cos Θ + µ � R sin Θ + R ˙ ˙ r sin θ + r ˙ r cos θ θ cos θ m 1 ˙ = m 1 m 1 � �� � � Θ sin Θ + µ R sin Θ + µ � R cos Θ − R ˙ ˙ r cos θ − r ˙ θ sin θ r sin θ − m 1 ˙ m 1 m 1 Θ + µ 2 m 1 R 2 ˙ r 2 ˙ rR − r ˙ θ + µ (˙ R )(sin θ cos Θ − cos θ sin Θ ) = m 1 + µ rR (˙ θ + ˙ Θ )(sin θ sin Θ + cos θ cos Θ ) .

A similarly tedious computation gives L z 2 = m 2 ( x 2 ˙ y 2 − ˙ x 2 y 2 ) Θ + µ 2 m 2 R 2 ˙ r 2 ˙ rR − r ˙ θ − µ (˙ R )(sin θ cos Θ − cos θ sin Θ ) = m 2 θ + ˙ − µ rR (˙ Θ )(sin θ sin Θ + cos θ cos Θ ) and we see lots of cancellations when we add the two: L tot = L z 1 + L z 2 z � 1 � + 1 ( m 1 + m 2 ) R 2 ˙ Θ + µ 2 r 2 ˙ θ. = m 1 m 2 Note that m − 1 1 + m − 1 = ( m 1 + m 2 ) / m 1 m 2 = 1 /µ , and so since m 1 + m 2 = M , we 2 find MR 2 ˙ L tot Θ + µ r 2 ˙ θ = z as desired!

(b) The Lagrangian for � r 1 , � r 2 can be written in cylinder coordinates in terms of centre-of-mass and relative coordinates as � ˙ + µ M R 2 + r 2 ˙ r 2 + r 2 ˙ Θ 2 � � θ 2 � − V ( � r 1 ,� L = ˙ r 2 ) 2 2 r 2 ) = V ( r ) , explain why both the relative angular momentum µ r 2 ˙ If V ( � r 1 ,� θ and the centre-of-mass angular momentum MR 2 ˙ Θ are separately conserved. This is quite easy, because we see that θ and Θ are cyclic coordinates for this Lagrangian, and thus p θ = ∂ L µ r 2 ˙ θ, = ∂ ˙ θ p Θ = ∂ L MR 2 ˙ = Θ ∂ ˙ Θ are both separately conserved quantities.