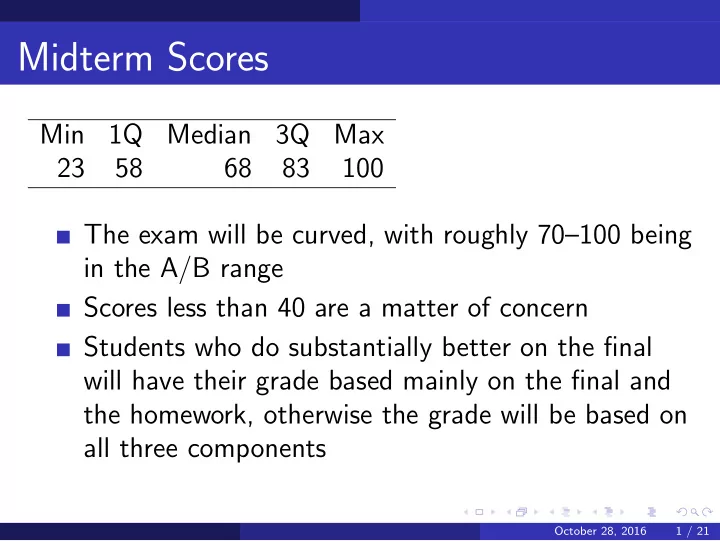
Midterm Scores Min 1Q Median 3Q Max 23 58 68 83 100 The - PowerPoint PPT Presentation

Midterm Scores Min 1Q Median 3Q Max 23 58 68 83 100 The exam will be curved, with roughly 70–100 being in the A/B range Scores less than 40 are a matter of concern Students who do substantially better on the final will have their grade based mainly on the final and the homework, otherwise the grade will be based on all three components October 28, 2016 1 / 21

Paths to Improvement Come to class Go to section Come to office hours Talk to me Read Lecture 1, slides 16–18, “How to Succeed in this Class” Keep up with the work. You can’t catch up on weeks of class in a couple of days of cramming October 28, 2016 2 / 21

Problem 1 One step in the manufacture of a medical device involves a fermentation process. A starter solution of engineered yeast cells has an estimated density of λ = 100,000 cells per ml (one milliliter = 1 thousandth of a liter). (a) Suppose that a sample of 1 µ l (one micro liter = 1 millionth of a liter) is taken of the starter solution and let X be the random variable defined as the number of yeast cells in the sample. Find the mean and the standard deviation of X . Find the coefficient of variation (CV) of X , defined as the ratio of the standard deviation to the mean. October 28, 2016 3 / 21

One µ l is 1/1000 of a ml, so the mean number of cells is cut by a factor of 1,000. Also, the distribution will be at least approximately Poisson, implying that the variance is equal to the mean. E ( X ) = V ( X ) = 100 SD ( X ) = 10 CV ( X ) = 10 / 100 = 0 . 1 October 28, 2016 4 / 21

(b) Suppose that the process needs that the CV of the sample number X be 0.05. How many microliters should the sample be to achieve this level? Suppose we sample k microliters and Y is the number of yeast cells E ( Y ) = V ( Y ) = 100 k √ SD ( Y ) = 10 k √ √ CV ( Y ) = 10 k / 100 k = 0 . 1 / k = 0 . 05 √ k = 2 k = 4 October 28, 2016 5 / 21

(c) If the sample volume is reduced to 0.1 µ l, what is the chance that there are no cells in the sample? The mean is reduces by an additional factor of 10, down to 10. The Poisson pdf is Pr ( X = x ) = e − 10 10 x / x ! so P ( X = 0) = e − 10 10 0 / 0! = e − 10 = 0 . 0000454 October 28, 2016 6 / 21

Problem 2 Batches of fibroblasts are treated to induce conversion to iPSC’s. A sample of 9 batches has a number of successfully converted cells as follows: { 3, 7, 5, 2, 4, 6, 8, 6, 4 } . (a) Find the mean, variance, and standard deviation of the number of converted cells. October 28, 2016 7 / 21

� x = 45 ¯ x = 45 / 9 = 5 x ) 2 = 30 � ( x − ¯ x 2 = 255 � x ) 2 = 255 − 9(5) 2 = 255 − 225 = 30 � ( x − ¯ s 2 = 30 / 8 = 3 . 75 √ s = 3 . 75 = 1 . 936 October 28, 2016 8 / 21

(b) Find the five-number summary (using the definitions from the book). What is the IQR? Ordered data: { 2 , 3 , 4 , 4 , 5 , 6 , 6 , 7 , 8 } . Ranks of quartiles are 2.5, 5, and 7.5 using f ( n + 1). So the five number summary is { 2 , 3 . 5 , 5 , 6 . 5 , 8 } . The IQR is 6 . 5 − 3 . 5 = 3 October 28, 2016 9 / 21

(c) Find the inner fences. Where are the ends of the whiskers in a boxplot? Lower inner fence = 3 . 5 − (1 . 5)(3) = − 1 Upper inner fence = 6 . 5 + (1 . 5)(3) = 11 The ends of the whiskers are at 2 and 8. October 28, 2016 10 / 21

Problem 3 A medical device company uses the following acceptance sampling procedure: Take a sample of size five from the (very large) shipment. Let X be the number of defects in the sample. If there are any defects in the sample ( X > 0), then reject the shipment; otherwise accept the shipment. (a) If the true probability is p = 0 . 1 that a random item in the shipment is defective, find the probability that the shipment is rejected. October 28, 2016 11 / 21

(a) If the true probability is p = 0 . 1 that a random item in the shipment is defective, find the probability that the shipment is rejected. Pr ( Rejected | p = 0 . 1) = Pr ( X > 0) = 1 − Pr ( X = 0) = 1 − (0 . 9) 5 = 0 . 4095 (b) If the true probability is p = 0 . 2 that a random item in the shipment is defective, find the probability that the shipment is rejected. Pr ( Rejected | p = 0 . 2) = Pr ( X > 0) = 1 − Pr ( X = 0) = 1 − (0 . 8) 5 = 0 . 6723 October 28, 2016 12 / 21

(c) Suppose that previous experience suggests that there is a 50% chance that a random shipment has p = 0 . 1 vs. p = 0 . 2. Find P ( p = 0 . 1 | Shipment Rejected ). ( Hint: first find P ( p = 0 . 1 ∩ Shipment Rejected ) and P ( p = 0 . 2 ∩ Shipment Rejected ), then P ( Shipment Rejected ), and finally P ( p = 0 . 1 | Shipment Rejected ) using the laws of probability.) October 28, 2016 13 / 21

Pr ( p = 0 . 1 ∩ Rejected ) = Pr ( Rejected | p = 0 . 1) Pr ( p = 0 . 1) = (0 . 4095)(0 . 5) = 0 . 2048 Pr ( p = 0 . 2 ∩ Rejected ) = Pr ( Rejected | p = 0 . 2) Pr ( p = 0 . 2) = (0 . 6723)(0 . 5) = 0 . 3362 Pr ( Rejected ) = 0 . 2048 + 0 . 3362 = 0 . 5409 Pr ( p = 0 . 1 | Rejected ) = Pr ( p = 0 . 1 ∩ Rejected ) / Pr ( Rejected ) = 0 . 2048 / 0 . 5409 = 0 . 3786 October 28, 2016 14 / 21

Problem 4 A piece of raw material for a medical device has an 80% chance of having no defects, a 15% chance of having 1 defect, and a 5% chance of having 2 defects. Let X be the random variable of the number of defects. (a) Find the mean, variance, and standard deviation of X . October 28, 2016 15 / 21

(a) Find the mean, variance, and standard deviation of X . x 2 P ( x ) P ( x ) xP ( x ) x 0 0.80 0 0 1 0.15 0.15 0.15 2 0.05 0.10 0.20 1.00 0.25 0.35 µ X = 0 . 25 = 0 . 35 − (0 . 25) 2 = 0 . 2875 σ 2 X σ X = 0 . 5362 October 28, 2016 16 / 21

(b) If each defect costs $5 to fix, let Y be the random variable of the cost of repair of the piece of raw material. Find the mean, variance, and standard deviation of Y . µ Y = (5)(0 . 25) = $1 . 25 σ 2 = (5) 2 (0 . 2875) = $7 . 19 Y σ Y = (5)(0 . 5362) = $2 . 68 October 28, 2016 17 / 21

(c) Suppose X i ( i = 1 , 2 , . . . , 20) are the numbers of defects in 20 pieces of raw material, assumed to be statistically independent. Find the mean, variance, and standard deviation of the total number of defects W in the 20 pieces. µ W = (20)(0 . 25) = 5 σ 2 = (20)(0 . 2875) = 5 . 75 W √ σ W = 5 . 75 = 2 . 398 October 28, 2016 18 / 21

Problem 5 Suppose that X is a uniform random variable, meaning that it has the pdf � 1 0 ≤ x ≤ 1 f ( x ) = 0 otherwise (a) Find the mean, variance, and standard deviation of X . October 28, 2016 19 / 21

(a) Find the mean, variance, and standard deviation of X . � 1 � 1 1 � � x dx = 1 2 x 2 µ X = xf ( x ) dx = = 0 . 5 � � 0 0 0 � 1 1 � x 2 dx = 1 3 x 3 � = 1 3 = 0 . 3333 � � 0 0 3 − 0 . 5 2 = 1 σ 2 1 = 12 = 0 . 0833 X √ σ X = 0 . 0833 = 0 . 289 October 28, 2016 20 / 21

(b) Find the mean, variance, and standard deviation of the average Y of X 1 , X 2 , . . . X 10 , where each of X i has the pdf as above, and they are statistically independent. µ Y = µ X = 0 . 5 σ 2 = σ 2 X / 10 = 0 . 00833 Y √ σ Y = σ X / 10 = 0 . 0913 October 28, 2016 21 / 21