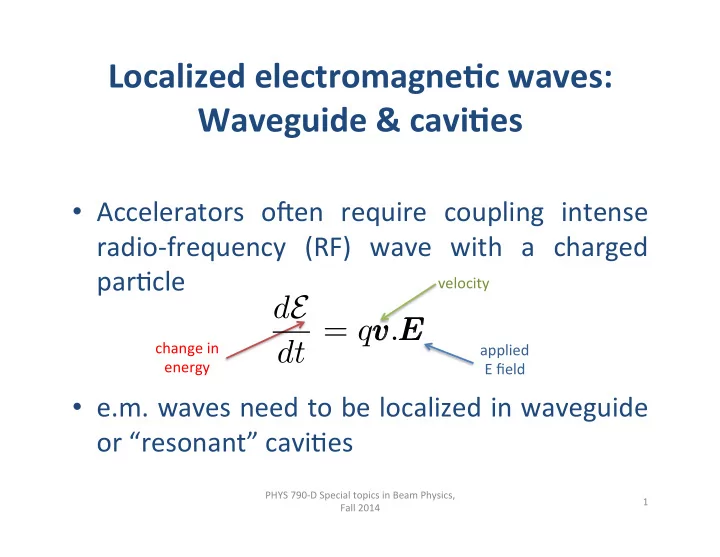
Localized electromagne0c waves: Waveguide & cavi0es - PowerPoint PPT Presentation

Localized ¡electromagne0c ¡waves: ¡ Waveguide ¡& ¡cavi0es ¡ ¡ • Accelerators ¡ o+en ¡ require ¡ coupling ¡ intense ¡ radio-­‑frequency ¡ (RF) ¡ wave ¡ with ¡ a ¡ charged ¡ par=cle ¡ velocity ¡ d E v E dt = qv v.E E change ¡in ¡ ¡ applied ¡ ¡ energy ¡ E ¡field ¡ • e.m. ¡waves ¡need ¡to ¡be ¡localized ¡in ¡waveguide ¡ or ¡“resonant” ¡cavi=es ¡ ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 1 ¡ Fall ¡2014 ¡

Example ¡of ¡a ¡plane ¡wave ¡(again) ¡ • For ¡a ¡plane ¡wave ¡in ¡ free ¡space : ¡ ¡ r, t ) = E 0 e i [ kz − ω t ] ˆ ˆ E ( r ˆ E r x E x x ¡ ¡ ¡ ¡ ¡this ¡wave ¡travels ¡along ¡the ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡direc=on. ¡ ¡ ¡ ˆ +ˆ ˆ z z z • change ¡in ¡ q ’s ¡energy ¡ Z + ∞ x x v = v ˆ x ˆ x x x ve i [ kz − ω t ] dt ' 0 ∆ E = qE 0 E E E −∞ • wave ¡and ¡ q ¡do ¡not ¡propagate ¡ v v k k k along ¡same ¡direc=on ¡ ˆ q z z z PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 2 ¡ Fall ¡2014 ¡

Boundary ¡condi0ons ¡ • at ¡a ¡perfect ¡conductors ¡surface: ¡ ˆ n n n n ˆ E n n × E E = 0 z ¡ S ¡ n ˆ B n n.B B = 0 ˆ ˆ n n n n n n • This ¡yields ¡to ¡the ¡mode ¡categoriza=on ¡ – transverse ¡magne=c ¡(TM): ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡everywhere ¡ ¡ B z = 0 ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡and ¡ ¡ E z | S = 0 – transverse ¡electric ¡(TE): ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡everywhere ¡ E z = 0 ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡and ¡ ∂ n B z | S = 0 PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 3 ¡ Fall ¡2014 ¡

case ¡of ¡parallel ¡plates ¡ ˆ x x x • consider ¡a ¡1-­‑D ¡waveguide ¡ a E E E • take ¡TE ¡mode: ¡ k k k B B B ¡ ¡ ¡ ¡ E y E E = E y ˆ y y 0 ˆ z z z ¡ ¡ ¡ ¡and ¡ ¡ ˆ y y y ¡ ¡ ¡ ¡ ¡ ¡ ¡ x, t ) = E 0 e i ω t [ e − i ( k z z + k x x ) − e − i ( k z z − k x x ) ] E y ( x x ¡ = − 2 iE 0 e i ( ω t − k z z ) sin( k x x ) PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 4 ¡ Fall ¡2014 ¡

case ¡of ¡parallel ¡plates: ¡boundaries ¡ ˆ x x x • E ¡vanishes ¡at ¡the ¡plates ¡ a E E E E y ( x = 0) = E y ( x = a ) = 0 k k k B B B 0 ˆ z z z • so ¡that ¡the ¡ x ¡wavevector ¡ ˆ y y y take ¡discrete ¡values ¡ • Can ¡also ¡derive ¡the ¡B ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ k x = m π field ¡from ¡ ¡ B E = � dB B ¡ a E r r r ⇥ E dt PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 5 ¡ Fall ¡2014 ¡

modes ¡ [ ¡picture ¡taken ¡from ¡L. ¡Daniel ¡(MIT)] ¡ • mode ¡are ¡characterized ¡ ¡ E B by ¡m: ¡ – m=1 ¡ � TE1 ¡ – m=2 ¡ � TE3 ¡ • note ¡that ¡ ¡ k = ω p c = ( k 2 z + k 2 x ) • so ¡ ¡ ⌘ 2 ⇣ m π ⌘ 2 ¡ ⇣ ω k 2 z = − c a PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 6 ¡ Fall ¡2014 ¡

dispersion ¡rela0ons ¡ need ¡to ¡intersect ¡with ¡ ¡ light ¡line ¡for ¡coupling ¡ ¡ • propaga=ng ¡modes ¡require ¡ k 2 z > 0 to ¡charged ¡par=cle ¡ • for ¡a ¡given ¡m ¡mode, ¡ ¡ only ¡frequency ¡above ¡ ω the ¡cutoff ¡ v φ ≡ ω /k z ω = k z c ω c ≡ cm π a ¡ ¡ ¡ ¡propagates. ¡ ω 2 • phase ¡velocity ¡always ¡ ω 1 v g ≡ d ω /dk z supraluminal… ¡ ¡ k z PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 7 ¡ Fall ¡2014 ¡

2-­‑D ¡waveguides ¡ • can ¡extend ¡the ¡ ¡ same ¡formalism ¡ ¡ for ¡a ¡rectangular ¡ ¡ waveguide ¡with ¡ ¡ 4 ¡sides ¡ ¡ • modes ¡are ¡characterized ¡by ¡2 ¡indices ¡ • cutoff ¡frequency ¡is ¡ r⇣ m π ⌘ 2 ⇣ n π ⌘ 2 ω c ≡ c + a b PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 8 ¡ Fall ¡2014 ¡

3-­‑D ¡boxes: ¡resonant ¡cavi0es ¡ • if ¡we ¡add ¡two ¡extra ¡wall ¡the ¡waveguide ¡is ¡ closed ¡and ¡referred ¡to ¡as ¡a ¡resonant ¡cavity ¡ • there ¡are ¡no ¡propaga=ng ¡modes ¡and ¡standing ¡ waves ¡are ¡established ¡ • the ¡mode ¡that ¡can ¡be ¡excited ¡are ¡eigenmode ¡ characterized ¡by ¡an ¡eigenfrequency ¡ ¡ r⇣ m π ⌘ 2 ⇣ n π ⌘ 2 ⇣ p π ⌘ 2 ω c ≡ c + + a b L length ¡of ¡the ¡cavity ¡ ¡ in ¡z ¡direc=on ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 9 ¡ Fall ¡2014 ¡

cylindrical-­‑symmetric ¡cavi0es ¡ • boundary ¡condi=ons ¡ on ¡cylindrical ¡surface ¡ ¡ Courtesy ¡of ¡H. ¡Padamsee, ¡Fermilab ¡ � ¡Bessel ¡func=on ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 10 ¡ Fall ¡2014 ¡

TM ¡modes ¡ • c ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 11 ¡ Fall ¡2014 ¡

TE ¡modes ¡ • c ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 12 ¡ Fall ¡2014 ¡

Quality ¡factor ¡ • figure ¡of ¡merit ¡for ¡any ¡oscillator ¡ Stored ¡energy ¡ In ¡the ¡system ¡ Quality ¡ ¡ Power ¡dissipated ¡ ¡ (or ¡ Q-­‑ ) ¡factor ¡ per ¡cycle ¡ • P ¡comes ¡from ¡ “ imperfec=on ” ¡in ¡the ¡system ¡ – For ¡copper ¡(normal-­‑conduc=ng) ¡cavi=es ¡this ¡ “ imperfec=on ” ¡ ¡ is ¡Ohmic ¡loss ¡ Q=10 6-­‑7 ¡ – For ¡superconduc=ng ¡cavi=es ¡(no ¡Joule ¡hea=ng) ¡these ¡ imperfec=ons ¡come ¡from ¡aperture ¡use ¡to ¡couple ¡the ¡ power ¡to ¡the ¡cavity ¡along ¡with ¡other ¡needed ¡instrument ¡ Q=10 10 ¡ – Non-­‑ ∞ ¡Q ¡ → ¡the ¡cavity ¡resonate ¡for ¡a ¡finite ¡<me ¡ ¡ τ ∼ Q/ ω PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 13 ¡ Fall ¡2014 ¡

Quality ¡factor ¡ • Q ¡is ¡also ¡representa=ve ¡of ¡=me ¡ ¡ – needed ¡to ¡fill ¡the ¡cavity ¡ – And ¡the ¡field ¡decay ¡=me ¡(a+er ¡excita=on ¡power ¡is ¡ turned ¡off) ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 14 ¡ Fall ¡2014 ¡

Resonant ¡cavi0es ¡-­‑-­‑ ¡introductory ¡remarks ¡II ¡ Example ¡of ¡cavi=es ¡ ¡ • accelera<ng ¡cavi<es ¡for ¡the ¡ ¡ Interna<onal ¡Linear ¡Collider ¡ Heavy ¡ions ¡accelera<ng ¡cavi<es ¡ ¡ Photonic ¡band-­‑gap ¡type ¡ ¡ accelera<ng ¡cavity ¡ ¡ The ¡academic ¡model ¡we ¡consider ¡ ¡ • (new ¡concept) ¡ is ¡the ¡case ¡of ¡a ¡ “ pillbox ” ¡cavity ¡ ¡ – ¡note ¡it ¡has ¡no ¡prac=cal ¡applica=on ¡ ¡ since ¡we ¡will ¡assumed ¡the ¡ ¡ cavity ¡is ¡closed ¡(no ¡aperture)… ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ Pillbox ¡w ¡apertures ¡