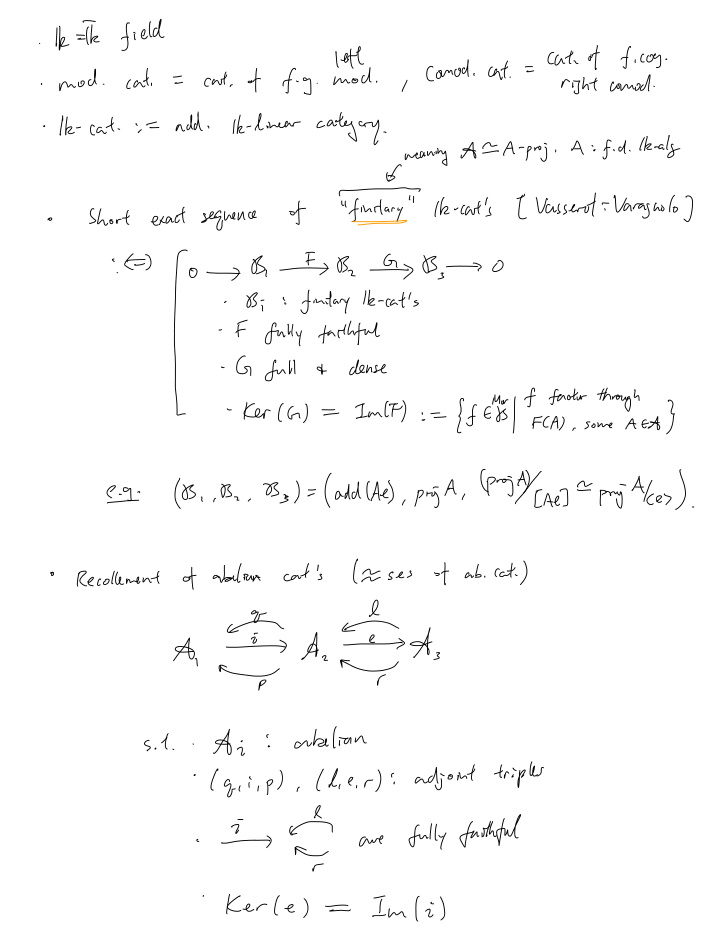



lk1k field cat of f cog Htt comod cat cat of f g mod cat mod right comod add 1h linear category 1k cat a A f d.lk alg AEA proj Ican.my 1k cat's Vasserotivaragaolo of Short exact sequence G B B B O o i findary 1k cat's Bi F fully faithful Gf dense t ggeqt.FI IItaeA Imt Ker h add Ae prg A.TT B B B pmjAkes EAe3 Recollement of abelian cat's Fuses of ab.cat l g c c A A A 3 2 7 I y r p abelian Ai s t adjoint triples L e r q i p Is fully faithful are Ker Im i e
Vitoria see for Psaroudakis Fact example A fad only mod A If A equivalent to then is recollement Ae Ae Ake man moat standard µ e dake Ake T Hamal Homme eti where in A idem et EA e Fat Indian K cat Rego i e g l Ake q pngA pnj Ae modele mont c page Akey nod Comal mod dualiu we will I categarify Upgrade j Mj pro finitely many obj t Han's are finitary calls Mtemiett Thyle e HE ftp.III e nice 2 category ztrepresentation of of e f7nte.ota.afegjd.cat s.es comaldDE corrode I C o 7 Mj E Mj C mj D I comodelE F coalgebm object in the abelianisation of the morphism 1 off's e algebra co
K mod E when Classical setting is Note Th horizontal compo compo of linear maps vertical compo description of Dd E relative to C Intrinsic there is Unlike correspondence 1k co algebra setting no Note idempotent and injective C comodules between ALGEBRA CO ALGEBRA From tansy la c endomorphism ab eAeT HaelQ it e g D ftp.msn.subcoaiaota Hai Yi a Q E D Soc 0 To To Amon I comod C also start from the other side We can E ii 102 I ice 4D YD I to Ae Y's.it'S Hm_ QQ ete
Remarks The functors are E C HELQ.CI 3 e Heem LD 3 Heel A cHEl cnn.detunCQ corroded corrode D D Q ED Hutton iQ Wh Hom e 27cal 1M 2 representation over es w i internal Hom 7 b bifunctural no nom M FN EHomeci.pl tnENiMJ F Homme Non trivial e.g of 2 cat nice NOT su e.g tensor category single obj case Cat of e g Elias Williamson bonodules Su Soergel e.g 2 art of finite truncation of quantum gp categoritoed upper low half of Khorana Lauda Rougnier
Recommend
More recommend