From Steklov to Neumann via homogenization Concentration of density - PowerPoint PPT Presentation

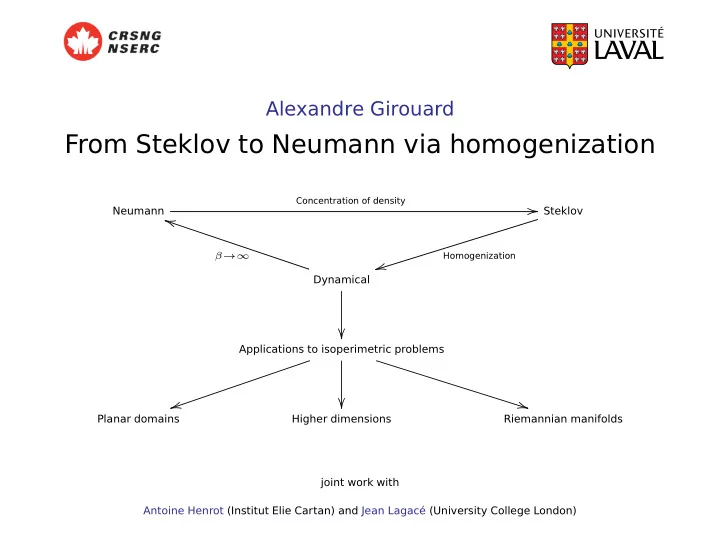
� � � � � � Alexandre Girouard From Steklov to Neumann via homogenization Concentration of density � Steklov Neumann β →∞ Homogenization Dynamical Applications to isoperimetric problems Planar domains Higher dimensions Riemannian manifolds joint work with Antoine Henrot (Institut Elie Cartan) and Jean Lagacé (University College London)

1. Neumann, Steklov and dynamical problems Let Ω ⊂ R d be open, bounded with smooth ∂ Ω Neumann Steklov � � − ∆ u = µ u in Ω ∆ u = 0 in Ω ∂ ν u = 0 on ∂ Ω ∂ ν u = σ u on ∂ Ω 0 = µ 0 ≤ µ 1 ≤ µ 2 ≤ · · · ր + ∞ 0 = σ 0 ≤ σ 1 ≤ σ 2 ≤ · · · ր + ∞ 2 / 23

1. Neumann, Steklov and dynamical problems Let Ω ⊂ R d be open, bounded with smooth ∂ Ω Neumann Steklov � � − ∆ u = µ u in Ω ∆ u = 0 in Ω ∂ ν u = 0 on ∂ Ω ∂ ν u = σ u on ∂ Ω 0 = µ 0 ≤ µ 1 ≤ µ 2 ≤ · · · ր + ∞ 0 = σ 0 ≤ σ 1 ≤ σ 2 ≤ · · · ր + ∞ Dynamical with parameter β ≥ 0 � − ∆ U = β Σ U in Ω ∂ ν U = Σ U on ∂ Ω 0 = Σ 0 ,β ≤ Σ 1 ,β ≤ Σ 2 ,β ≤ · · · ր ∞ Reference: François – von Below (2005) 2 / 23

Mass concentration along ∂ Ω Let w : Ω − → R be a positive density Consider the non-homogeneous Neumann problem � − ∆ u = µ wu in Ω ∂ ν u = 0 on ∂ Ω If the density w concentrates on ∂ Ω , then µ k (Ω , w ) → σ k (Ω) References: Bandle 1980 Arrieta – Jiménez-Casas – Rodríguez-Bernal 2008 Lamberti – Provenzano 2014 3 / 23

� � ⋆ − ⇀ d H ∂ Ω w dx � Steklov Neumann Dynamical 4 / 23

2. Homogenization theory 5 / 23

Let Ω ⊂ R d be open, bounded with smooth ∂ Ω Given ε > 0 and k ∈ Z d � � d − ε 2 , ε B ε T ε k = ε k + k = B ( ε k , r ε ) 2 I ε = { k ∈ Z d : B ε k ⊂ Ω } � Ω ε = Ω \ T ε k k ∈ I ε 6 / 23

� Ω ε = Ω \ T ε T ε = B ( ε k , r ε ) where k ∈ I ε 7 / 23

Girouard – Henrot – Lagacé 2019 Ω ε = Ω \ T ε T ε = ∪ k ∈ I ε B ( ε k , r ε ) where A d := | ∂ B ( 0 , 1 ) | 8 / 23

Girouard – Henrot – Lagacé 2019 Ω ε = Ω \ T ε T ε = ∪ k ∈ I ε B ( ε k , r ε ) where A d := | ∂ B ( 0 , 1 ) | Dynamical problem with parameter β ≥ 0 � − ∆ U = β Σ U in Ω ∂ ν U = Σ U on ∂ Ω Main homogenization theorem  ε → 0 | ∂ T ε | − − − → β | Ω |  ∼ β ε d = r d − 1 ⇒ ε A d ε → 0 σ k (Ω ε )  − − − → Σ k ,β Reference: arXiv:1906.09638 8 / 23

Trichotomy Analogous to the crushed ice problem � Ω ε = Ω \ T ε T ε = where B ( ε k , r ε ) k ∈ I ε Critical regime If r d − 1 ∼ A − 1 d βε d , then | ∂ T ε | → β | Ω | and σ k (Ω ε ) → Σ k ,β ε Small holes, β = 0 Thawing If r d − 1 = o ( ε d ) , then | ∂ T ε | → 0 and σ k (Ω ε ) → σ k (Ω) ε Large holes Freezing /ε d = + ∞ , then | ∂ T ε | → ∞ and σ k (Ω ε ) → 0 ε → 0 r d − 1 If lim ε 9 / 23

� � Girouard – Henrot – Lagacé 2019 ⋆ − ⇀ d H ∂ Ω w dx � Steklov Neumann homogenization Dynamical 10 / 23

� � Girouard – Henrot – Lagacé 2019 ⋆ − ⇀ d H ∂ Ω w dx � Steklov Neumann β →∞ homogenization Dynamical Theorem β →∞ β Σ k ,β = µ k lim Reference: arXiv:1906.09638 10 / 23

Corollary for planar domains r ε ∼ β 2 πε 2 A 2 = | ∂ D | = 2 π | ∂ Ω ε | = | ∂ Ω | + | ∂ T ε | ε → 0 − − − → | ∂ Ω | + β | Ω | 11 / 23

Corollary for planar domains r ε ∼ β 2 πε 2 A 2 = | ∂ D | = 2 π | ∂ Ω ε | = | ∂ Ω | + | ∂ T ε | ε → 0 − − − → | ∂ Ω | + β | Ω | Area-Normalized Neumann β →∞ ε → 0 σ k (Ω ε ) | ∂ Ω ε | − − − → Σ k ,β ( | ∂ Ω | + β | Ω | ) − − − − → µ k (Ω) | Ω | Perimeter-Normalized Steklov 11 / 23

3. Applications to isoperimetric problems If Ω ⊂ R 2 is simply-connected then. . . Szeg˝ o (1954) Weinstock (1954) µ 1 (Ω) | Ω | ≤ µ 1 ( D ) × π ∼ = 3 . 39 π σ 1 (Ω) | ∂ Ω | ≤ σ 1 ( D ) × 2 π = 2 π with equality if and only if Ω is a disk 12 / 23

3. Applications to isoperimetric problems If Ω ⊂ R 2 is simply-connected then. . . Szeg˝ o (1954) Weinstock (1954) µ 1 (Ω) | Ω | ≤ µ 1 ( D ) × π ∼ = 3 . 39 π σ 1 (Ω) | ∂ Ω | ≤ σ 1 ( D ) × 2 π = 2 π with equality if and only if Ω is a disk If Ω ⊂ R 2 is simply-connected then. . . Weinberger (1956) Kokarev (2014) µ 1 (Ω) | Ω | ≤ µ 1 ( D ) × π σ 1 (Ω) | ∂ Ω | < 8 π 12 / 23

3. Applications to isoperimetric problems If Ω ⊂ R 2 is simply-connected then. . . Szeg˝ o (1954) Weinstock (1954) µ 1 (Ω) | Ω | ≤ µ 1 ( D ) × π ∼ = 3 . 39 π σ 1 (Ω) | ∂ Ω | ≤ σ 1 ( D ) × 2 π = 2 π with equality if and only if Ω is a disk If Ω ⊂ R 2 is simply-connected then. . . Weinberger (1956) Kokarev (2014) µ 1 (Ω) | Ω | ≤ µ 1 ( D ) × π ?? σ 1 (Ω) | ∂ Ω | < 2 π ?? 12 / 23

Let Ω ε := B ( 0 , 1 ) \ B ( 0 , ε ) Dittmar (2004) σ 1 (Ω ε ) = σ 1 ( D ) + o ( ε ) = 1 + o ( ε ) This implies σ 1 (Ω ε ) | ∂ Ω ε | = 2 π + 2 πε + o ( ε ) > 2 π For ε ∼ = 0 . 2, we have σ 1 (Ω ε ) | ∂ Ω ε | ∼ = 2 . 17 π 13 / 23

How large can σ 1 (Ω) | ∂ Ω | be for Ω ⊂ R 2 ? 2 . 17 π < sup { σ 1 (Ω) | ∂ Ω | : Ω ⊂ R 2 } ≤ 8 π 14 / 23

How large can σ 1 (Ω) | ∂ Ω | be for Ω ⊂ R 2 ? 2 . 17 π < sup { σ 1 (Ω) | ∂ Ω | : Ω ⊂ R 2 } ≤ 8 π Homogenization of Ω = D Perimeter-Normalized Steklov ε → 0 β →∞ σ 1 (Ω ε ) | ∂ Ω ε | − → Σ 1 ,β ( | ∂ Ω | + β | Ω | ) − → µ 1 (Ω) | Ω | = µ 1 ( D ) | D | Area-Normalized Neumann Corollary 3 . 39 π ≈ µ 1 ( D ) π ≤ sup { σ 1 (Ω) | ∂ Ω | : Ω ⊂ R 2 } ≤ 8 π 14 / 23

How large can σ k (Ω) | ∂ Ω | be for Ω ⊂ R 2 ? Conjecture 1 There is no isoperimetric maximizer for σ 1 15 / 23

How large can σ k (Ω) | ∂ Ω | be for Ω ⊂ R 2 ? Conjecture 1 There is no isoperimetric maximizer for σ 1 Conjecture 2 sup { σ 1 (Ω) | ∂ Ω |} = µ 1 ( D ) × π ∼ = 3 . 39 π 15 / 23

How large can σ k (Ω) | ∂ Ω | be for Ω ⊂ R 2 ? Conjecture 1 There is no isoperimetric maximizer for σ 1 Conjecture 2 sup { σ 1 (Ω) | ∂ Ω |} = µ 1 ( D ) × π ∼ = 3 . 39 π Conjecture 3 sup { σ k (Ω) | ∂ Ω |} = sup { µ k (Ω) | Ω |} 15 / 23

Isoperimetric bounds: Steklov − → Neumann Corollary Let Ω ⊂ R 2 be open, bounded with smooth ∂ Ω Then µ 1 (Ω) | Ω | ≤ 8 π Proof. It follows from the above that Kokarev < 8 π � �� � σ 1 (Ω ε ) | ∂ Ω ε | µ 1 (Ω) | Ω | = lim β →∞ lim ε → 0 This inequality is of course not new The moral is new Bounds on σ k which depends only on the perimeter can be used to prove bounds on µ k 16 / 23

Isoperimetry in higher dimensions: Ω ⊂ R d Colbois – El Soufi – Girouard (2011) There exists a constant C = C ( d ) such that σ k (Ω) | ∂ Ω | ≤ C | Ω | 1 − 2 d k 2 / d (1) Applying to Ω ε ⊂ Ω and taking ε → 0 gives Σ k ,β ( | ∂ Ω | + β | Ω | ) ≤ C | Ω | 1 − 2 d k 2 / d 17 / 23

Isoperimetry in higher dimensions: Ω ⊂ R d Colbois – El Soufi – Girouard (2011) There exists a constant C = C ( d ) such that σ k (Ω) | ∂ Ω | ≤ C | Ω | 1 − 2 d k 2 / d (1) Applying to Ω ε ⊂ Ω and taking ε → 0 gives Σ k ,β ( | ∂ Ω | + β | Ω | ) ≤ C | Ω | 1 − 2 d k 2 / d Corollary Corollary 2 / d is the optimal exponent in (1) Kröger (1992) µ k (Ω) | Ω | 2 / d ≤ Ck 2 / d Any smaller exponent would contradict Weyl’s law for µ k 17 / 23

4. Homogenization on a closed Riemannian manifold M Joint work with Jean Lagacé (University College London) M : closed Riemannian manifold, β ∈ C ∞ ( M ) positive − ∆ f = βλ f 0 = λ 0 ≤ λ 1 ( M , β ) ≤ λ 2 ( M , β ) ≤ · · · ր ∞ . Theorem There is a sequence Ω ε ⊂ M of domains such that ⋆ ε → 0 d H ∂ Ω ε − ⇀ β dx σ k (Ω ε ) − − − ⇀ λ k ( M , β ) and Corollary If M is a surface, there is Ω ε ⊂ M such that ε → 0 σ k (Ω ε ) | ∂ Ω ε | − − − ⇀ λ k ( M ) A ( M ) . Reference: arXiv: coming next week. . . 18 / 23

5. Proofs Variational characterizations Eigenfunctions are the critical points of the Dirichlet energy: � H 1 (Ω) ∋ u �− |∇ u | 2 → Ω Under the following constraints Dynamical Steklov � � � u 2 = 1 . u 2 + 2 πβ u 2 = 1 . ∂ Ω Ω ∂ Ω 19 / 23

5. Proofs Variational characterizations Eigenfunctions are the critical points of the Dirichlet energy: � H 1 (Ω) ∋ u �− |∇ u | 2 → Ω Under the following constraints Dynamical Steklov � � � u 2 = 1 . u 2 + 2 πβ u 2 = 1 . ∂ Ω Ω ∂ Ω ( U , Σ) is a dynamical eigenpair ( u , σ ) is a Steklov eigenpair ⇐ ⇒ ⇐ ⇒ ∀ v ∈ C ∞ (Ω) � � � � � ∇ U ·∇ v = Σ( 2 πβ Uv + Uv ) ∇ u · ∇ v = σ uv Ω Ω ∂ Ω Ω ∂ Ω 19 / 23