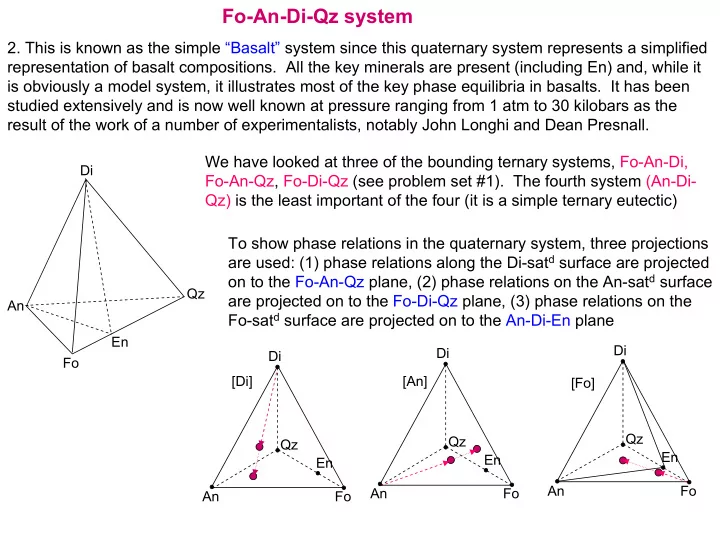
Fo-An-Di-Qz system 2. This is known as the simple Basalt system - PowerPoint PPT Presentation

Fo-An-Di-Qz system 2. This is known as the simple “Basalt” system since this quaternary system represents a simplified representation of basalt compositions. All the key minerals are present (including En) and, while it is obviously a model system, it illustrates most of the key phase equilibria in basalts. It has been studied extensively and is now well known at pressure ranging from 1 atm to 30 kilobars as the result of the work of a number of experimentalists, notably John Longhi and Dean Presnall. We have looked at three of the bounding ternary systems, Fo-An-Di, Di Fo-An-Qz, Fo-Di-Qz (see problem set #1). The fourth system (An-Di- Qz) is the least important of the four (it is a simple ternary eutectic) To show phase relations in the quaternary system, three projections are used: (1) phase relations along the Di-sat d surface are projected on to the Fo-An-Qz plane, (2) phase relations on the An-sat d surface Qz are projected on to the Fo-Di-Qz plane, (3) phase relations on the An Fo-sat d surface are projected on to the An-Di-En plane En Di Di Di Fo [Di] [An] [Fo] Qz Qz Qz En En En An Fo An Fo An Fo

Fo-An-Di-SiO 2 (“Basalt”) system at P = 1 atm: Perspective representation Di P: isobaric invariant point (peritectic): L P (An-Fo-Di-En) Heavy lines: isobaric, univariant equilibria (inside tetrahedron) Reaction at P is: Fo + L P ↔ An + En + Di Light lines: isobaric, univariant Di Q: isobaric invariant point (eutectic): L Q (An-En-Di-Qz) equilibria on surface (ternary Reaction at Q is: L Q ↔ An + En + Di + Qz systems) i h P - 1240ºC a-P: L(An,Di,Fo) Pig f d-P: L(An,Fo,En) Q - 1211ºC g S-P: L(Fo,En,Di) a R - 1310ºC P-Q: L(An,En,Di) S S - 1322ºC R R-Q: L(En,Di,Qz) S-R: L(Di,En,Pig) Q i-S: L(Di,Fo,Pig) P f-S: L(En,Fo,Pig) An Qz En g-R: L(En,Pig,Qz) b An SiO 2 e d sp c Note: (1) Spinel compositions lie outside the tetrahedron. En, Pig, and Di show limited solid Fo solution. All other minerals have fixed composition En (2) Thermal maximum along a-P and b-c (3) All compositions in wt. % Fo After: Presnall et al. (1979) J. Pet., 20, 3-35

Wo Fo-Di-An-Qz system at P = 1 atm The results obtained in this 4- [An]: Projection of An-sat d surface component system are very [An] important because they provide [Fo]: Projection of Fo-sat d surface an essential framework to [Di]: Projection of Di-sat d surface understand experiments carried a out using natural rocks as Di starting material in experiments. P: 1240ºC b What major components are Q: 1211ºC Di+An m missing in this simple system? m: Maximum on field boundary P(1240) Fo+An Q(1211) Qz En+An Qz+An Sp+An Wo c d e Qz Fo En [Di] [Fo] Di+Qz Di R g Q(1211) Pig+Di i En+Di En i Di+Fo Pig + Fo a P(1240) S f S(1320) m An+Di An+Fo Fo+Di m P(1240) En+Fo Sp+Fo a An Fo d An En After: Longhi (1987) Am J. Sci.

En (Mg 2 Si 2 O 6 ) - Di (CaMgSi 2 O 6 ) at P = 20 kb (2 GPa) 1800 At pressures above ~2-3 kb, L L+En SS the pyroxene (En-Di) join L+Di SS appears to be binary. At lower a En SS b pressures, Fo is the liquidus En 1600 L+Pig SS Pig SS + phase. Pig T(ºC) Note the lower stability limit Di SS Pig+Di SS c of pigeonite. 1400 En SS + Di SS Note: 3 isobaric equilibria represented by the reactions: a : En ss + L ↔ Pig ss 1200 20 40 60 80 Di En b : Pig ss + L ↔ Di ss Wt. % c : Pig ↔ En ss + Di ss Two-pyroxene geothermometer: Widely used in natural assemblages containing orthopyroxene and clinopyroxene Example: Cpx with composition Di 70 En 30 coexisting with Opx of composition En 96 Di 4 would represent a pyroxene pair formed at 1350ºC. Applications of this geothermometer to natural assemblages is subject to a number of limitations. Think of what some of these limitations might be?