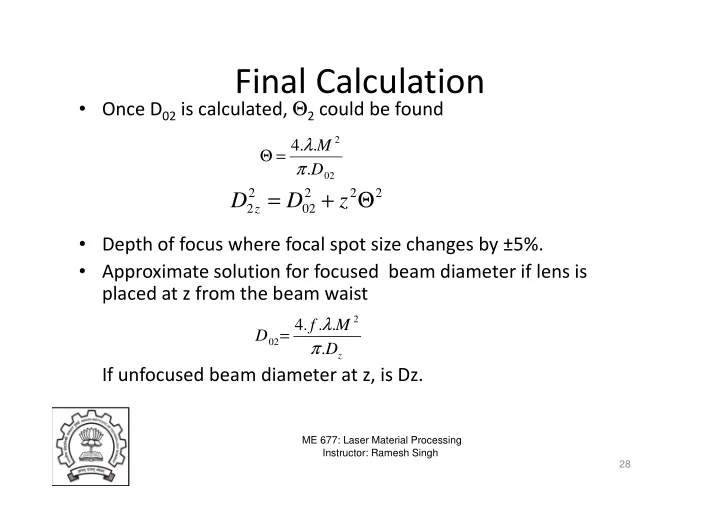
Final Calculation Once D 02 is calculated, 2 could be found 2 4 - PowerPoint PPT Presentation

Final Calculation • Once D 02 is calculated, Θ 2 could be found 2 λ 4 . . M Θ = π . D 02 2 2 2 2 = + Θ D z D z 2 02 • Depth of focus where focal spot size changes by ±5%. • Depth of focus where focal spot size changes by ±5%. • Approximate solution for focused beam diameter if lens is placed at z from the beam waist 2 λ 4 . f . . M = D 02 π . D z If unfocused beam diameter at z, is Dz. ME 677: Laser Material Processing Instructor: Ramesh Singh 28

Aberrations • Spherical Aberration • Thermal Distortion • Astigmatism • Damage Damage ME 677: Laser Material Processing Instructor: Ramesh Singh 29

Spherical Aberration • There are two reasons why a lens will not focus to a theoretical point – Diffraction limited problem – Spherical lens is not a perfect shape. – Spherical lens is not a perfect shape. • Most lenses are made with a spherical shape since this can be accurately manufactured economically • The alignment of the beam is not so critical as with a perfect aspheric shape ME 677: Laser Material Processing Instructor: Ramesh Singh 30

Thermal Distortion • High power laser beams are absorbed by lenses/optics – Selection of right optics ZnSe with CO2 – The power distribution in TEM00 causes more – The power distribution in TEM00 causes more severe gradients than Donut • Shape change of lens • Varies the refractive index, specially in ZnSe ME 677: Laser Material Processing Instructor: Ramesh Singh 31

Astigmatism and Damage • Due to optical misalignment • Damage – Due to dirt accumulation and burning on lens surface surface ME 677: Laser Material Processing Instructor: Ramesh Singh 32

Summary • Absorption • Beam Modes • Propagation • Focusing Focusing • Aberrations ME 677: Laser Material Processing Instructor: Ramesh Singh 33

Analytical Modeling of Laser Moving Sources Moving Sources

Contains: • Heat flow equation • Analytic model in one dimensional heat flow • Heat source modeling – Point heat source – Line heat source – Plane heat source – Surface heat source • Finite difference formulation • Finite elements

Heat flow equation For developing basic heat flow equation, consider the differential element . Heat balance in element is given by, Heat in – Heat out + Heat generated = Heat accumulated Heat flow through differential Heat in and out rates depends on conduction element and convection. ∂ T 2 ∇ − ρ − ρ ∇ = − k T C C U T H p p ∂ t

Analytic model in one dimensional heat flow: If the heat flow in only one direction and there is no convection or heat generation, the basic equation becomes where α = diffusivity, t = time. It is assumed that there is constant thermal properties, with no radiant heat loss or melting, then the BC ‘s are The solution is,

Heat source modelling: Introduction: • Why modeling? 1. Semi-quantitative understanding of the process mechanism for the design of experiments. 2. Parametric understanding for control purpose. E.g. statistical charts. 3. Detailed understanding to analyse the precise process mechanisms for the purpose of prediction, process improvement . for the purpose of prediction, process improvement . Types of heat sources: Point heat source. Line heat source. Plane heat source. (e.g. circular , rectangular)

1.Instantaneous point heat source: The differential equation for the conduction of heat in a stationary medium assuming no convection or radiation, is This is satisfied by the solution for infinite body, 2 2 2 δ − + − + − q ( x x ') ( y y ') ( z z ') ( ) = − dT ' x y z t , , , exp[ ] 3 − 4 ( a t t ') ρ π − C (4 a t ( t ')) 2 − semi inf inite 2 2 2 δ − + − + − 2 q ( x x ') ( y y ') ( z z ') ( ) = − dT ' x y z t , , , exp[ ] 3 − 4 ( a t t ') ρ π − 2 C (4 a t ( t ')) δ q = instantaneous heat generated, C = sp. heat capacity, α = diffusivity, ρ = Density, t = time, K = thermal conductivity. gives the temperature increment at position (x, y, z) and time t due to an instantaneous heat source δ q applied at position (x’, y’, z’) and time t’.