FASTENER AS FAIL-SAFE DISBOND/DELAMINATION ARREST FOR LAMINATED - PDF document

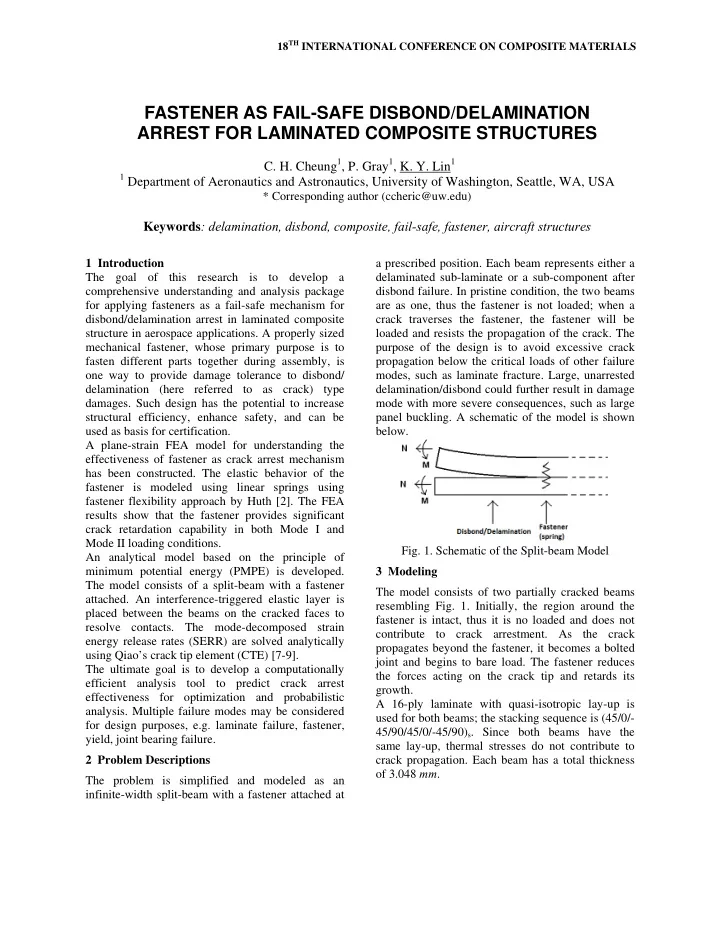
18 TH INTERNATIONAL CONFERENCE ON COMPOSITE MATERIALS FASTENER AS FAIL-SAFE DISBOND/DELAMINATION ARREST FOR LAMINATED COMPOSITE STRUCTURES C. H. Cheung 1 , P. Gray 1 , K. Y. Lin 1 1 Department of Aeronautics and Astronautics, University of Washington, Seattle, WA, USA * Corresponding author (ccheric@uw.edu) Keywords : delamination, disbond, composite, fail-safe, fastener, aircraft structures 1 Introduction a prescribed position. Each beam represents either a The goal of this research is to develop a delaminated sub-laminate or a sub-component after disbond failure. In pristine condition, the two beams comprehensive understanding and analysis package for applying fasteners as a fail-safe mechanism for are as one, thus the fastener is not loaded; when a disbond/delamination arrest in laminated composite crack traverses the fastener, the fastener will be structure in aerospace applications. A properly sized loaded and resists the propagation of the crack. The mechanical fastener, whose primary purpose is to purpose of the design is to avoid excessive crack fasten different parts together during assembly, is propagation below the critical loads of other failure one way to provide damage tolerance to disbond/ modes, such as laminate fracture. Large, unarrested delamination (here referred to as crack) type delamination/disbond could further result in damage damages. Such design has the potential to increase mode with more severe consequences, such as large structural efficiency, enhance safety, and can be panel buckling. A schematic of the model is shown used as basis for certification. below. A plane-strain FEA model for understanding the effectiveness of fastener as crack arrest mechanism has been constructed. The elastic behavior of the fastener is modeled using linear springs using fastener flexibility approach by Huth [2]. The FEA results show that the fastener provides significant crack retardation capability in both Mode I and Mode II loading conditions. Fig. 1. Schematic of the Split-beam Model An analytical model based on the principle of minimum potential energy (PMPE) is developed. 3 Modeling The model consists of a split-beam with a fastener The model consists of two partially cracked beams attached. An interference-triggered elastic layer is resembling Fig. 1. Initially, the region around the placed between the beams on the cracked faces to fastener is intact, thus it is no loaded and does not resolve contacts. The mode-decomposed strain contribute to crack arrestment. As the crack energy release rates (SERR) are solved analytically propagates beyond the fastener, it becomes a bolted using Qiao’s crack tip element (CTE) [7-9]. joint and begins to bare load. The fastener reduces The ultimate goal is to develop a computationally the forces acting on the crack tip and retards its efficient analysis tool to predict crack arrest growth. effectiveness for optimization and probabilistic A 16-ply laminate with quasi-isotropic lay-up is analysis. Multiple failure modes may be considered used for both beams; the stacking sequence is (45/0/- for design purposes, e.g. laminate failure, fastener, 45/90/45/0/-45/90) s . Since both beams have the yield, joint bearing failure. same lay-up, thermal stresses do not contribute to crack propagation. Each beam has a total thickness 2 Problem Descriptions of 3.048 mm . The problem is simplified and modeled as an infinite-width split-beam with a fastener attached at

AS4/3501-6 laminar material properties are used. crack tip. The crack tip does not need to be modeled Ply thickness = 0.1905 mm; E 1 = 127.5 GPa ; E 2 = elastically since the CTE already considers crack tip E3 = 11.3 GPa ; G 12 = G 13 = 6.0 GPa ; G 23 = 3.6 rotation and shear deformation. A set of springs GPa ; ν 12 = ν 13 = 0.3; ν 23 = 0.4; G IC = 0.2627 N / mm ; attached to the free end of the beams represent the G IIC = 1.226 N/mm ; mixed-mode fracture parameter fastener. Loads are applied to the free end of the used in the B-K law is η = 1.75, shown in Eq. (1). beams, assuming that the far field loads reaches the  (1) fastener location unaltered.   G      G G G G II   A layer of “infinite stiffness” contact springs is equiv IC IIC IC  G G   I II placed between the beams. The contact springs are only activated when interference is detected; The fastener is made of Titanium, with E = 114 interference must be resolved iteratively. The GPa . The fastener interacts with the two beams via stiffness of the contact springs is selected such that the fastener flexibility model [2], shown in Eq. (2). convergence can be obtained without generating For single-lap bolted graphite/epoxy joints, the numerical errors related to machine size numbers. constants are a = 2/3, b = 4.2 and n = 1. The System equilibrium is solved using PMPE. resulting joint stiffness is 1/C . Trigonometric series are used as shape functions for a the beams. The energy contribution by the contact    ( 2 )  t t  b 1 1 1 1     C 1 2     spring is shown in Eq. (3), where w1 and w2 are the  d  n t E nt E t E nt E 2  2 2  1 1 2 2 1 3 2 3 shape functions of the split beams. Force and It is assumed that disbond/delamination in laminated moment equilibrium provides input for determining composites propagates in a self-similar fashion. the SERRs using Qiao’s CTE. Thus, the crack tip always remains at the prescribed N ( 3 ) 1      2 U k w w interface between the two beams regardless of load | EL n  n  2 1 2 L   conditions.  n 1  N  3.1 FEM Model in Abaqus Although contact is resolved iteratively, each step is a linear elastic analysis, thus retaining the The fastened split-beam is modeled in Abaqus using computational efficiency of the method. Competing a combination of a plane-strain elements and spring failure modes, such as surface strain failure, fastener elements that which describes the equivalent elastic failure and joint failure, can easily be integrated into behavior of the fastener joint. Strain energy release this method to provide a comprehensive design tool. rate used to calculate crack propagation is evaluated using Virtual Crack Closure Technique (VCCT). 4 Results and Discussions Crack face friction, fastener preload, thermal 4.1 FEM Results stresses can be optional included. Each ply is represented by one element, thus each Fig.2 shows a load vs. crack length curve for a load element has a thickness of 0.1905 mm . Element case with an opening moment applied to only one of length is 1.5 times the thickness, which provides the beams. This asymmetric load case yields mixed- converged VCCT and displacement results. mode, though primarily Mode I, SERR components Initially, the intact region is modeled by tying the at the crack tip. The fastener is located at crack nodes of the two beams at the interface with location zero. displacement constraints. The FEA solver iterates The horizontal portions of the curves imply that the for a load magnitude that yields exactly the SERRs crack propagation is unstable, which is catastrophic needed to propagate the crack. Then, the tie in nature. The case without the fastener shows that constraint at the node at the crack tip is released, the crack propagation is totally unstable with no opening the crack. The next node along the interface opportunity of arrest. becomes the new crack tip. In the case with a fastener, when the crack propagates pass fastener, it is slowed by the arresting 3.2 Analytical Model effect of the fastener, as shown by the rising load The analytical model includes two separate beams curve. The load required to propagate the crack just attached to a fixed boundary, which represents the 2 mm beyond the fastener is double the initial