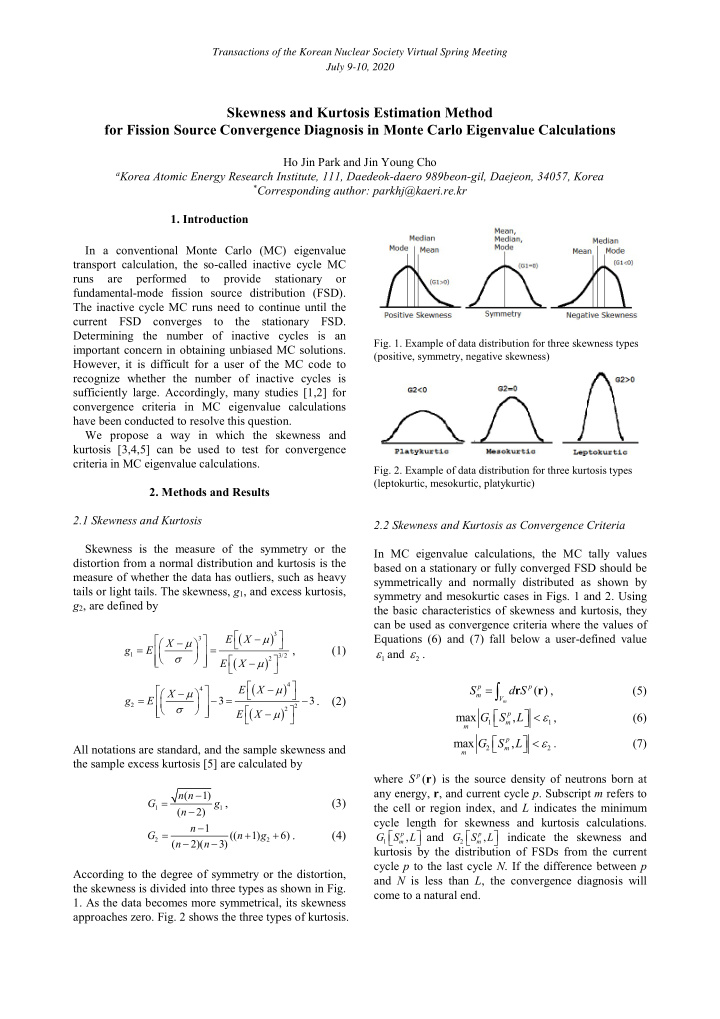



Transactions of the Korean Nuclear Society Virtual Spring Meeting July 9-10, 2020 Skewness and Kurtosis Estimation Method for Fission Source Convergence Diagnosis in Monte Carlo Eigenvalue Calculations Ho Jin Park and Jin Young Cho a Korea Atomic Energy Research Institute, 111, Daedeok-daero 989beon-gil, Daejeon, 34057, Korea * Corresponding author: parkhj@kaeri.re.kr 1. Introduction In a conventional Monte Carlo (MC) eigenvalue transport calculation, the so-called inactive cycle MC runs are performed to provide stationary or fundamental-mode fission source distribution (FSD). The inactive cycle MC runs need to continue until the current FSD converges to the stationary FSD. Determining the number of inactive cycles is an Fig. 1. Example of data distribution for three skewness types important concern in obtaining unbiased MC solutions. (positive, symmetry, negative skewness) However, it is difficult for a user of the MC code to recognize whether the number of inactive cycles is sufficiently large. Accordingly, many studies [1,2] for convergence criteria in MC eigenvalue calculations have been conducted to resolve this question. We propose a way in which the skewness and kurtosis [3,4,5] can be used to test for convergence criteria in MC eigenvalue calculations. Fig. 2. Example of data distribution for three kurtosis types (leptokurtic, mesokurtic, platykurtic) 2. Methods and Results 2.1 Skewness and Kurtosis 2.2 Skewness and Kurtosis as Convergence Criteria Skewness is the measure of the symmetry or the In MC eigenvalue calculations, the MC tally values distortion from a normal distribution and kurtosis is the based on a stationary or fully converged FSD should be measure of whether the data has outliers, such as heavy symmetrically and normally distributed as shown by tails or light tails. The skewness, g 1 , and excess kurtosis, symmetry and mesokurtic cases in Figs. 1 and 2. Using g 2 , are defined by the basic characteristics of skewness and kurtosis, they can be used as convergence criteria where the values of 3 Equations (6) and (7) fall below a user-defined value E X 3 X g E , (1) and . 1 3/2 2 1 2 E X 4 E X p p S d S r ( ) r , (5) 4 X m . (2) g E 3 3 V m 2 2 2 E X p max G S , L , (6) 1 m 1 m p max G S , L . (7) All notations are standard, and the sample skewness and 2 m 2 m the sample excess kurtosis [5] are calculated by p where S ( ) r is the source density of neutrons born at any energy, r , and current cycle p . Subscript m refers to n n ( 1) , (3) G g the cell or region index, and L indicates the minimum 1 1 ( n 2) cycle length for skewness and kurtosis calculations. n 1 . (4) G (( n 1) g 6) G S p , L and G S p , L indicate the skewness and 2 2 ( n 2)( n 3) 1 m 2 m kurtosis by the distribution of FSDs from the current cycle p to the last cycle N . If the difference between p According to the degree of symmetry or the distortion, and N is less than L , the convergence diagnosis will the skewness is divided into three types as shown in Fig. come to a natural end. 1. As the data becomes more symmetrical, its skewness approaches zero. Fig. 2 shows the three types of kurtosis.
Transactions of the Korean Nuclear Society Virtual Spring Meeting July 9-10, 2020 Moreover, the skewness and kurtosis estimation method Figs. 3 and 4 plot the trends of the cycle-wise can be used for judging whether MC-tally values are skewness and kurtosis, respectively. As the cycle fully-converged or not. proceeds, the skewness and kurtosis are closer to 0.0. Table II shows the results of convergence cycles by each method. For comparison, the convergence cycle by G Q , (8) 1 m 1 the Ueki’s posterior source convergence diagnosis [1] G Q . (9) and Shim’s on-the-fly stopping criterion (Type A & B) 2 m 2 [2] were calculated. where Q means any MC tally of cell m . and G Q m 1 m 5 G Q indicate the skewness and kurtosis by the 2 m 4 cycle-wise MC tallies from the 1 st cycle to the last cycle N . The modules for these methods were implemented 3 Cycle-wise Skewness into the McCARD MC code [6]. 2 1 0.5 2.3 Slab Test Problem 0 -0.5 To examine the effectiveness of the convergence -1 criteria for skewness, simple slab problems were -2 considered. We took the slab problem from Ref. [7] and -3 its cross sections are shown in Table I. The 10cm 1D CEL1 CEL2 CEL3 CEL4 CEL5 -4 slab was divided equally into ten cells. All cells had the CEL6 CEL7 CEL8 CEL9 CEL10 same cross section and the leftmost (cell 1) and -5 0 200 400 600 800 rightmost (cell 10) boundary surfaces of the slab had CYCLE reflective boundary conditions. The dominance ratio Fig. 3. Cycle-wise cumulative skewness of slab problem for the slab problem was about 0.92. (biased case) Table I: Slab Problem Description 16 Parameter Value CEL1 CEL2 CEL3 CEL4 Total cross section( ) 1.0 12 CEL5 CEL6 t CEL7 CEL8 Scattering cross section( ) 0.6 Cycle-wise Kurtosis s ,0 CLE9 CEL10 Production cross section ( v ) 0.48 8 f Width (cm) 10 Number of cell (#) 10 4 Uniform case Initial Fission Source (FS) 0.5 /Biased case 0 -0.5 In the slab problem, all McCARD calculations were performed using 1,000 cycles ( N ) with 100,000 neutron -4 histories per cycle, and no skipped cycle. The mean and 0 100 200 300 400 500 standard deviation for skewness and kurtosis were CYCLE Fig. 4. Cycle-wise cumulative kurtosis of the slab problem calculated from 59 replicas with different random (biased case) number sequences. In this study, 0.5 was used as the , because ± 0.5 is generally convergence criterion 1 In the ‘uniform’ case, the number of inactive cycles considered as the acceptable range of skewness for determined by the Ueki’s method was 3, while those by normal distribution [8]. The other convergence the Shim’s types A and B stopping criteria with the criterion and the minimum cycle length L were 2 default option were 45 and 50. The convergence cycles arbitrarily set as 0.5 and 100, respectively. To examine of the uniform case by skewness and kurtosis estimation the change of the convergence cycle length due to the method were 5 whereas those in the biased case were 46 initial FSD, we considered uniform and biased cases. and 50. Overall, we found that the convergence cycle by The initial fission sources for the ‘uniform’ case were = =0.5) agreed well with those by the new method ( uniformly sampled from the whole slab whereas those 1 2 the Ueki’s posterior source convergence diagnosis. for the ‘biased’ case were only sampled in ‘cell 1’ at the Table III shows the maximum skewness or kurtosis left boundary of the slab. among the results by Equations (8) and (9) for each cell. By the skewness and kurtosis estimation method, the
Recommend
More recommend