e
play

E 0 k i ( r k r r t ) r = r = c | r E E 0 e k | B 0 i ( r k r r - PowerPoint PPT Presentation

Oct 09, 2023 •169 likes •464 views

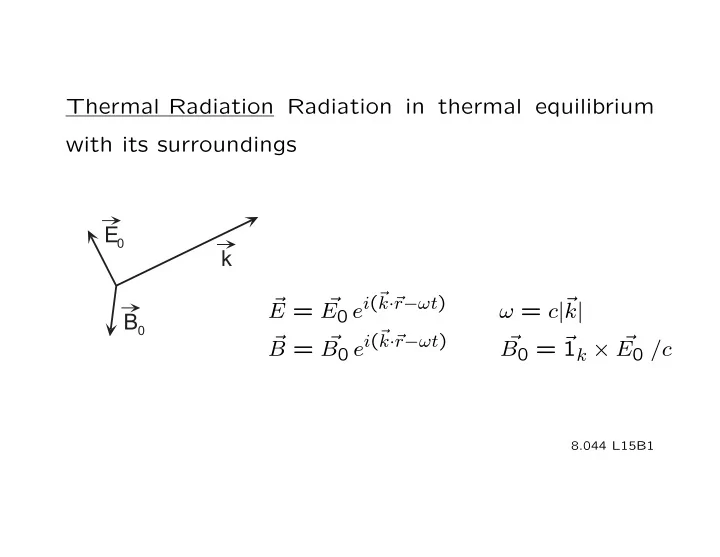
Thermal Radiation Radiation in thermal equilibrium with its surroundings E 0 k i ( r k r r t ) r = r = c | r E E 0 e k | B 0 i ( r k r r t ) r r r r r B = B 0 e B 0 = 1 k E 0 /c 8.044 L 15 B1 - 0 | 2 1 Time

  1. Thermal Radiation Radiation in thermal equilibrium with its surroundings E 0 k i ( r k · r r − �t ) r = r � = c | r E E 0 e k | B 0 i ( r k · r r − �t ) r r r r r B = B 0 e B 0 = 1 k × E 0 /c 8.044 L 15 B1

  2. - 0 | 2 1 Time average energy density u = E 0 | E 2 - j E = ( cu ) - Time average energy flux 1 k Time average pressure ( ⇒ to - k ) P = u Thermal radiation has a continuous distribution of frequencies. u( ν ,T) Peaks near hν = 3 k B T ( h/k B � 5 × 10 − 11 K-sec) ν 8.044 L 15 B2

  3. Spectral Region ν (Hz) T (K) Thermal Rad. 10 6 1 . 7 × 10 − 5 Radio 10 10 Microwave 0 . 17 cosmic background 10 13 1 . 7 × 10 2 Infrared room temp. 2 × 10 15 8 . 5 × 10 3 Visible sun’s surface 1 10 16 1 . 7 × 10 5 Ultraviolet 10 18 1 . 7 × 10 7 X ray black holes 10 21 1 . 7 × 10 10 γ ray 8.044 L15B3

  4. ENERGY ABSORBED ABSORPTIVITY α ( ν ,T) ENERGY INCIDENT ISOTROPIC ENERGY EMITTED EMISSIVE POWER e ( ν ,T) AREA ISOTROPIC 8.044 L15B4

  5. THERMAL RADIATION: PROPERTIES 2 ENERGY FLUXES, IN AND OUT OF CAVITY B T B CAVITY A T A CAVITY B FILTER: FREQUENCY OR POLARIZATION ASSUME T A = T B AND THERMAL EQUILIBRIUM 8.044 L15B5

  6. CONCLUSIONS: • u ( ν, T ) is independent of shape and wall material • u ( ν, T ) is isotropic • u ( ν, T ) is unpolarized 8.044 L 15 B6

  7. CONSIDER AN OBJECT IN THE CAVITY, IN THERMAL EQUILIBRIUM COMPUTE THE ENERGY FLUX T dA c ∆ t θ n ∆ A 8.044 L15B7

  8. ∆ E = ( E in cylinder) p ( θ, φ ) dθ dφ sin θ 1 = ( u ∆ A cos θ c ∆ t ) dθ dφ 2 2 π π/ 2 cos θ sin θ 2 π 1 = c u ∆ A ∆ t dθ dφ 0 2 0 2 π 1 1 / 4 1 ⇒ energy flux onto dA = 4 c u ( ν, T ) 8.044 L 15 B8

  9. Momentum Flux r = u r Plane wave momentum density 1 k p c | ∆ p | = 2 | p ⇒ | since p ⇒ in = r − r p ⇒ out 8.044 L 15 B9

  10. 2 cos θ | ∆ p | ν = ( E in cylinder) p ( θ, φ ) dθ dφ c 2 π 1 π/ 2 cos 2 θ sin θ dθ = u ( ν, T ) ∆ A ∆ t dφ 0 0 2 π 1 / 3 1 1 = 3 u ( ν, T ) ∆ A ∆ t � u ( ν, T ) dν ⇒ P ( T ) = 1 3 0 8.044 L 15 B10

  11. Apply detailed balance to the object in the cavity. E out = E in 1 c u ( ν, T )) dA e dA = α ( 4 e ( ν, T ) 1 ⇒ = 4 c u ( ν, T ) α ( ν, T ) This ratio has a universal form for all materials. The result is known as KIRCHOFF’S LAW. 8.044 L 15 B11

  12. Black Body Radiation If α ≡ 1 ≡ “Black” Then e ( ν, T ) = 1 4 c u ( ν, T ) OVEN Measure e ( ν, T ) CAVITY AT and obtain u ( ν, T ) T 8.044 L 15 B12

  13. Thermodynamic Approach ∞ u ( T ) ≡ u ( ν, T ) dν 0 Then E ( T, V ) = u ( T ) V 1 P ( T, V ) = 3 u ( T ) 8.044 L 15 B 13

  14. This is enough to allow us to find u ( T ). dE = TdS − PdV ∂E ∂S ∂P � � � � � � = T − P = T − P ∂V ∂V ∂T T T V 1 Tu � ( T ) − 1 = 3 u ( T ) 3 also = u ( T ) u � ( T ) = 4 u ( T ) � T u ( T ) = AT 4 8.044 L 15 B 14

  15. Emissive Power of a Black ( α = 1) Body 4 c u ( T ) = 1 AcT 4 e ( ν, T ) = 1 4 c u ( ν, T ) ⇒ e ( T ) = 1 4 4 e ( T ) ∞ σT This is known as the STEFAN-BOLTZMANN LAW. σ = 56 . 7 × 10 − 9 watts/ m 2 K 4 8.044 L 15 B 15

  16. Statistical Mechanical Approach H ? Single normal mode (plane standing wave) in a rectangular conducting cavity. E x z 0 L r r, t ) = E ( t ) sin( nπz/L ) r E ( r 1 x 0 , 0 ,n,r 1 x r, t ) = ( nπc 2 /L ) − 1 E r ˙ ( t ) cos( nπz/L ) r B ( r 1 y 0 , 0 ,n,r 1 y 8.044 L 15 B 16

  17. 1 - · E - + 1 - · B - Energy density = 1 E 0 E 0 B [ no - r or t average ] µ 2 2   H = V  1 E 0 E 2 ( t ) + 1 1 ( nπc 2 /L ) − 2 E ˙ 2 ( t )  2 2 2 µ 0 V E 0 E 2 ( t ) + ( nπc/L ) − 2 E ˙ 2 ( t ) = 2 2 � Each mode corresponds to a harmonic oscillator. 8.044 L 15 B 17

  18. Count the modes. i�t r E n x ,n y ,n z = | E | r j sin( n x πx/L ) sin( n y πy/L ) sin( n z πz/L ) e The unit polarization vector E j has 2 possible o rthog- onal directions and n i = 1 , 2 , 3 · · · . � 2 E � 2 E πc � � 2 = − c � 2 E = 0 2 E 2 2 2 ( n + n + n ) � x y z �t 2 L 8.044 L 15 B 18

  19. If the radian frequency < ω n z L � 2 2 2 R R = n + n + n = ω x y z πc # modes (freq. < ω ) n y 1 4 = 2 × 8 × 3 πR 3 GRID SPACING 3 1 UNIT n x π L ω 3 = 3 πc 8.044 L 15 B 19

  20. � 3 d # L V � ω 2 ω 2 D ( ω ) = = π = π 2 c 3 dω πc D( ω ) ω 8.044 L 15 B 20

  21. Classical Statistical Mechanics D ( � ) k B T 3 � 2 < E ( � ) > = k B T � u ( �, T ) = < E ( � ) > = π 2 c V ∞ u ( T ) = u ( �, T ) d� = ∞ 0 CLASSICAL u( ω , T) MEASURED ω 8.044 L 15 B2 1

  22. Quantum Statistical Mechanics ¯ h� < E ( � ) > = + ¯ h�/ 2 ¯ h�/kT − 1 e � 3 D ( � ) ¯ h u ( �, T ) = < E ( � ) > = + z. p. term π 2 c 3 ¯ h�/kT − 1 V e du ( �, T ) To find the location of the maximum, set = 0 . d� The maximum occurs at ¯ h�/kT ≈ 2 . 82. 8.044 L 15 B 22

  24. − ¯ hω/ 2 kT (1 − e − ¯ hω/kT ) − 1 Z = Z i Z i = e states i The first factor in the expression for Z i comes from the zero-point energy. F ( V, T ) = − kT ln Z = − kT ln Z i � states i � � = − kT D ( ω ) [ln Z i ] dω 0 8.044 L 15 B 23

  25. � � � − ¯ hω/kT ) F ( V, T ) = − kT D ( ω ) − ln(1 − e dω + · · · 0 kT V � ω 2 ln(1 − e − ¯ hω/kT ) dω = π 2 c 3 0 V � 2 ln(1 − e ( kT ) 4 − x ) dx = x h 3 π 2 c 3 ¯ 0 v- _ − π 4 45 π 2 1 ( kT ) 4 V = − h 3 45 c 3 ¯ 8.044 L 15 B 24

  26. π 2 ∂F 1 � � ( kT ) 4 P = − = h ) 3 ∂V T 45 ( c ¯ π 2 4 ∂F � � k 4 T 3 V S = − = h ) 3 ∂T V 45 ( c ¯ π 2 1 4 1 � � ( kT ) 4 V E = F + TS = + ( · · · ) = − h ) 3 45 45 15 ( c ¯ Note: P = 1 3 E/V = 1 3 u ( T ) independent of V . 8.044 L 15 B 25

  27. NOTE: THE ADIABATIC PATH IS T 3 V=CONSTANT T ADIABATIC T/T 0 = (V/V 0 )- 1 / 3 V 8.044 L15B26

  28. MIT OpenCourseWare http://ocw.mit.edu 8.044 Statistical Physics I Spring 2013 For information about citing these materials or our Terms of Use, visit: http://ocw.mit.edu/terms.

Recommend

More recommend