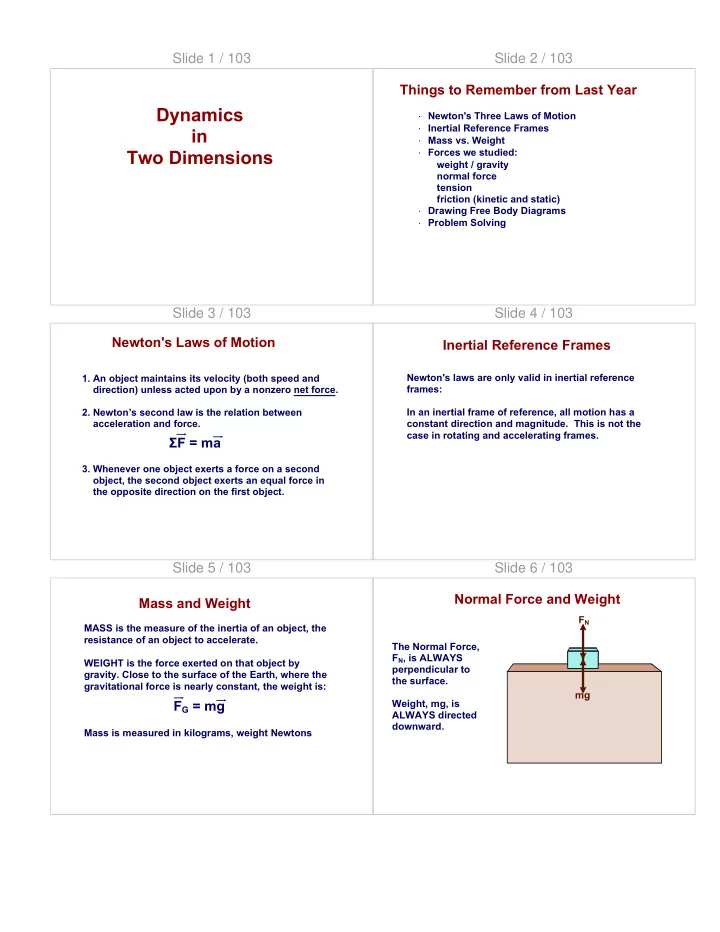

Slide 1 / 103 Slide 2 / 103 Things to Remember from Last Year Dynamics · Newton's Three Laws of Motion · Inertial Reference Frames in · Mass vs. Weight · Forces we studied: Two Dimensions weight / gravity normal force tension friction (kinetic and static) · Drawing Free Body Diagrams · Problem Solving Slide 3 / 103 Slide 4 / 103 Newton's Laws of Motion Inertial Reference Frames 1. An object maintains its velocity (both speed and Newton's laws are only valid in inertial reference frames: direction) unless acted upon by a nonzero net force. 2. Newton’s second law is the relation between In an inertial frame of reference, all motion has a acceleration and force. constant direction and magnitude. This is not the case in rotating and accelerating frames. ΣF = ma 3. Whenever one object exerts a force on a second object, the second object exerts an equal force in the opposite direction on the first object. Slide 5 / 103 Slide 6 / 103 Normal Force and Weight Mass and Weight F N MASS is the measure of the inertia of an object, the resistance of an object to accelerate. The Normal Force, F N , is ALWAYS WEIGHT is the force exerted on that object by perpendicular to gravity. Close to the surface of the Earth, where the the surface. gravitational force is nearly constant, the weight is: mg F G = mg Weight, mg, is ALWAYS directed downward. Mass is measured in kilograms, weight Newtons
Slide 7 / 103 Slide 8 / 103 Kinetic Friction Static Friction Friction forces are v Static friction is ALWAYS parallel to always equal and the surface exerting f K f S F APP opposite the Net them. Applied Force acting on the object Kinetic friction is always directed (not including friction). opposite to direction the object Its magnitude is: is sliding and has magnitude: f S ≤ μ S F N f K = μ k F N Slide 9 / 103 Slide 10 / 103 Tension Force When a cord or rope pulls on an object, it is said to be under Two Dimensions tension, and the force it exerts is called a tension force, F T . F T a mg Slide 11 / 103 Slide 12 / 103 Resolving Forces Resolving Forces Consider a child pulling a wagon down the street. The wagon has a handle that is not vertical, not horizontal, but at an angle. This means the child is Since forces are vectors, they may have both a pulling UP and OVER at the same time. horizontal and vertical influence on an object. In order to solve problems using forces acting at an angle, we must find the horizontal (x) and vertical (y) components of the forces using trigonometry (right triangles/SohCahToa).
Slide 13 / 103 Slide 14 / 103 Resolving Forces A free body diagram would include this, and all y (vertical) component other forces, as seen below. # F N x (horizontal) component F app F f To find the net force on the object, we consider each component separately. Let's assume the force the child pulled with was 50 Newtons at 30 o mg F = 50 N y (vertical) component F N F y # x (horizontal) component F f F app F x mg Slide 15 / 103 Slide 16 / 103 F = 50 N y (vertical) component F = 50 N F y y (vertical) component F y 30 o 30 o x (horizontal) component x (horizontal) component F x = 43.3 N F x The horizontal (x) component of the force is equal to 43.3 N. We can include this on a free body diagram: We can use COSINE = Adjacent / Hypotenuse to find F x F N F N F f F f F x F x mg mg But if we do that, we lose the vertical (y) component of the original force... so we must find that next: So F x = 43.3 N Slide 17 / 103 Slide 18 / 103 F = 50 N y (vertical) component F = 50 N F y y (vertical) component = 25 N F y 30 o 30 o x (horizontal) component x (horizontal) component F x = 43.3 N F x The vertical (y) component of the force is equal to 25 N. We can now add this to complete the free body diagram: We can use SINE = Opposite/ Hypotenuse to find F y F y F y F N F N F f F f F x F x mg mg Notice that our original force F app is no longer shown... it So F y = 25 N can be replaced by the x and y components!
Slide 19 / 103 Slide 20 / 103 Resolving forces practice: Resolving forces practice: Resolve each of the forces into x and y components, and then show the components on a free body diagram. Show your work on a separate page! Resolve each of the forces into x and y components, and then 1. 2. show the components on a free body diagram. 100N 500N Ex. 45 o 15 o 20N 40 o 4. 3. 250N 22 o -25 o 330N Slide 21 / 103 Slide 22 / 103 Resolving forces Homework: Force and friction acting on an object Resolve each of the forces into x and y components, and then show the components on a free body diagram. Show your work on a separate page! 1. Previously, we solved 2. problems with multiple 80N forces, but they were 600N either parallel or 40 o 35 o perpendicular. For instance, draw the free body diagram of 4. the case where a box 3. is being pulled along a 1500N surface, with friction, 12 o -60 o at constant speed. 24N Slide 23 / 103 Slide 24 / 103 Force and friction acting on an object Force and friction acting on an object F N y - axis x - axis F N Now find the ΣF = ma ΣF = ma F APP acceleration given f F APP - f k = ma that the applied F N - mg = 0 F APP f force is 20N, the F APP - μ k F N = ma F N = mg box has a mass of mg F APP - μ k mg = ma F N = (3.0kg)(10m/s 2 ) 3.0kg, and the F APP = 20N coefficient of kinetic a = (F APP - μ k mg)/m mg F N = 30N m = 3.0kg friction is 0.20. a = (20N - (0.20)(30N))/3.0kg μ k = 0.20 a = (20N - 6.0N)/3.0kg a = (14N)/3.0kg a = 4.7 m/s 2
Slide 25 / 103 Slide 26 / 103 Forces at angles acting on an object Forces at angles acting on an object The next, critical, step is to choose axes. F N Now we'll solve F APP Previously, we always problems where the used vertical and f forces act at an angle horizontal axes, since so that it is not parallel one axis lined up with or perpendicular with the forces...and the one another. acceleration. mg First we do a free body Now, we must choose axes so that all the acceleration is diagram, just as we did along one axis, and there is no acceleration along the other. previously. You always have to ask, "In which direction could this object accelerate?" Then make one axis along that direction, and the other perpendicular to that. What's the answer in this case? Slide 27 / 103 Slide 28 / 103 Forces at angles acting on an object Forces at angles acting on an object y y This time vertical and Now we have to break horizontal axes still any forces that don't F N F N X X F APP F APP work...since we assume line up with our axes the box will slide along into components that f f the surface. do. However, if this In this case, F APP , must assumption is wrong, mg be broken into mg we'll get answers that don't make sense, and F x and F y we'll have to reconsider our choice. Slide 29 / 103 Slide 30 / 103 Forces at angles acting on an object Force and friction acting on an object y y Let's use our work Once we do that, F N X to find the F N X we can now acceleration if the F y F y proceed as we did f f applied force is 20N previously, just F F at 37 o above x x using each horizontal, the box component has a mass of mg appropriately. mg 3.0kg, and the coefficient of kinetic friction is 0.20.
Slide 31 / 103 Slide 32 / 103 Normal Force and Friction Force and friction acting on an object Friction was reduced F APP = 20N at 37 o x - axis y - axis because the Normal F N m = 3.0kg F APPy Force was reduced; the ΣF = ma ΣF = ma μ k = 0.20 box's weight, mg, was F x - f k = ma F N + F y - mg = 0 supported by the y- mg y component of the force F N F x - μ k F N = ma F N = mg - F y plus the Normal X Fcos # - μ k mg = ma F N = mg - Fsin # Force...so the Normal F APPy f Just looking at the y-axis F N = (3.0kg)(10m/s 2 ) Force was a = (Fcos # - μ k F N )/m F APPx - (20N)(sin37 o ) lowered...lowering a = (20N cos37 o ΣF = ma F y friction. mg F N = 30N - 12N - (0.20)(18N))/3.0kg F N + F y - mg = 0 F N F N = 18N a = (16N - 3.6N)/3.0kg F N = mg - F y Note that F N is lower mg a = (12.4N)/3.0kg F N = mg - Fsin # due to the force helping a = 4.1 m/s 2 to support the object Slide 33 / 103 Slide 34 / 103 Normal Force and Friction Normal Force and Friction y F N What would happen What would happen X with both the Normal with both the Normal Force and Friction in Force and Friction in f the case that the object the case that the object is being pushed along is being pushed along F APP the floor by a downward the floor by a downward angled force. angled force? mg Slide 35 / 103 Slide 36 / 103 Normal Force and Friction 1 The normal force on the box is: y F N In this case the pushing X force is also pushing mg A the box into the surface, F f x F app increasing the Normal θ B mg sin# Force as well as friction. F y C mg cos# mg Just looking at the y-axis D mg + F sin# F N E mg - F sin# ΣF = ma F N - F y - mg = 0 mg F N = mg + F y F N = mg + Fsin # F y http://njc.tl/6v
Recommend
More recommend