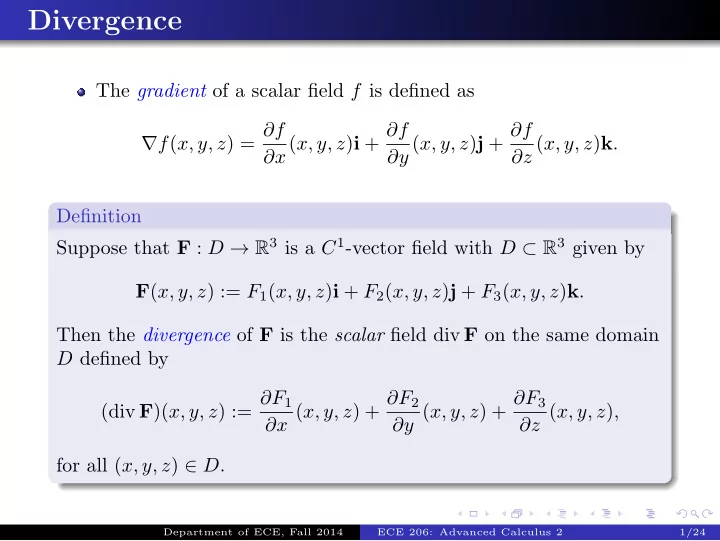
Divergence The gradient of a scalar field f is defined as f ( x, y, - PowerPoint PPT Presentation

Divergence The gradient of a scalar field f is defined as ∇ f ( x, y, z ) = ∂f ∂x ( x, y, z ) i + ∂f ∂y ( x, y, z ) j + ∂f ∂z ( x, y, z ) k . Definition Suppose that F : D → R 3 is a C 1 -vector field with D ⊂ R 3 given by F ( x, y, z ) := F 1 ( x, y, z ) i + F 2 ( x, y, z ) j + F 3 ( x, y, z ) k . Then the divergence of F is the scalar field div F on the same domain D defined by (div F )( x, y, z ) := ∂F 1 ∂x ( x, y, z ) + ∂F 2 ∂y ( x, y, z ) + ∂F 3 ∂z ( x, y, z ) , for all ( x, y, z ) ∈ D . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 1/24

Divergence (cont.) We can define the symbolic vector ∇ as ∇ := ∂ ∂x i + ∂ ∂y j + ∂ ∂z k . Using this vector, one can define div F = ∇ · F . Thus, ∇ · F is an alternative notation for div F (often preferred in more modern books). Remark: A vector field F : D → R 3 , with D ⊂ R 3 , is called sole- noidal ( incompressible ), if ∇ · F = 0. We will see later that the magnetic field B is always solenoidal. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 2/24

Curl Defintion Suppose that F : D → R 3 is a C 1 -vector field with D ⊂ R 3 given by F ( x, y, z ) := F 1 ( x, y, z ) i + F 2 ( x, y, z ) j + F 3 ( x, y, z ) k . Then the curl of F is the vector field curl F on the same domain D defined by � ∂F 3 ∂y ( x, y, z ) − ∂F 2 � (curl F )( x, y, z ) := ∂z ( x, y, z ) i + � ∂F 1 ∂z ( x, y, z ) − ∂F 3 � ∂x ( x, y, z ) j + � ∂F 2 ∂x ( x, y, z ) − ∂F 1 � ∂y ( x, y, z ) k . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 3/24

Curl (cont.) An alternative way to define curl F would be as � � i j k � � ∂ ∂ ∂ � � curl F = ∇ × F = . � � ∂x ∂y ∂z � � F 1 F 2 F 3 � � (Physical interpretation: http://mathinsight.org/curl idea .) Theorem Suppose F : D → R 3 is a C 1 -vector field with D = R 3 . Then the fol- lowing are equivalent 1 F is a conservative vector field; 2 � Γ F ( r ) · d r = 0 for every closed curve Γ in R 3 ; 3 ( ∇ × F )( x, y, z ) = 0 for all ( x, y, z ) ∈ R 3 . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 4/24

Curl (cont.) Remark A vector field F : R 3 → R 3 is called irrotational if ( ∇ × F )( x, y, z ) = 0 for all ( x, y, z ) ∈ R 3 . Thus, F is conservative iff it is irrotational. Theorem Suppose that f : D → R is a C 2 -scalar field with D ⊂ R 3 . Then the vector field ∇ f is irrotational, that is ∀ ( x, y, z ) ∈ R 3 . ( ∇ × ( ∇ f ))( x, y, z ) = 0 , Theorem Suppose that G : D → R 3 is a C 2 -vector field with D ⊂ R 3 . Then the vector field ∇ × G is solenoidal, that is ∀ ( x, y, z ) ∈ R 3 . ( ∇ · ( ∇ × G ))( x, y, z ) = 0 , Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 5/24

Laplacian Definition Suppose that f : D → R is a C 2 -scalar field with D ⊂ R 3 . Then the Laplacian of the scalar field f is another scalar field denoted by ∆ f and defined on the same domain D by ∆ f ( x, y, z ) = ∂ 2 f ∂x 2 ( x, y, z )+ ∂ 2 f ∂y 2 ( x, y, z )+ ∂ 2 f ∂z 2 ( x, y, z ) , ∀ ( x, y, z ) ∈ R 3 . Definition Suppose that F : D → R 3 is a C 2 -vector field with domain D ⊂ R 3 given by F ( x, y, z ) := F 1 ( x, y, z ) i + F 2 ( x, y, z ) j + F 3 ( x, y, z ) k , ∀ ( x, y, z ) ∈ D. The Laplacian of the vector field F is another vector field ∆ F on the same domain D defined by ∆ F ( x, y, z ) := ∆ F 1 ( x, y, z ) i + ∆ F 2 ( x, y, z ) j + ∆ F 3 ( x, y, z ) k . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 6/24

Laplacian (cont.) Theorem Suppose that f : D → R is a C 2 -scalar field with D ⊂ R 3 . Then the Laplacian of f is the divergence of the gradient of f , that is ∆ f ( x, y, z ) := ∇ · ( ∇ f )( x, y, z ) , ∀ ( x, y, z ) ∈ D. Remark Suppose that f : D → R is a C 2 -scalar field such that its gradient is solenoidal. Then, ∆ f ( x, y, z ) := 0 , ∀ ( x, y, z ) ∈ D. The above relation is a particular case of Laplace’s equation . Any f that satisfies the relation is called a solution of Laplace’s equation. Laplace’s equation is ubiquitous throughout mathematical phy- sics and engineering. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 7/24

Time derivative Let F ( x, y, z, t ) be a time-dependant vector field defined on some domain D ⊂ R 3 . Then, it can be shown that ∂t [( ∇ · F )( x, y, z, t )] = ∇ · ∂ F ( x, y, z, t ) ∂ . ∂t Moreover, ∂t [( ∇ × F )( x, y, z, t )] = ∇ × ∂ F ( x, y, z, t ) ∂ . ∂t Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 8/24

Useful identities ∇ ( cf )( x, y, z, t ) = c ( ∇ f )( x, y, z, t ) ∇ ( f + g )( x, y, z, t ) = ( ∇ f )( x, y, z, t ) + ( ∇ g )( x, y, z, t ) ∇ ( fg )( x, y, z, t ) = g ( x, y, z, t )( ∇ f )( x, y, z, t )+ f ( x, y, z, t )( ∇ g )( x, y, z, t ) ∇ ( f/g )( x, y, z, t ) = g ( x, y, z, t )( ∇ f )( x, y, z, t ) − f ( x, y, z, t )( ∇ g )( x, y, z, t ) , g 2 ( x, y, z, t ) for all ( x, y, z, t ) such that g ( x, y, z, t ) � = 0. ∆( fg )( x, y, z, t ) = g ( x, y, z, t )(∆ f )( x, y, z, t ) + f ( x, y, z, t )(∆ g )( x, y, z, t )+ + 2( ∇ f · ∇ g )( x, y, z, t ) Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 9/24

Useful identities (cont.) ∇ · ( c F )( x, y, z, t ) = c ( ∇ · F )( x, y, z, t ) ∇ · ( F + G )( x, y, z, t ) = ( ∇ · F )( x, y, z, t ) + ( ∇ · G )( x, y, z, t ) ∇ × ( c F )( x, y, z, t ) = c ( ∇ × F )( x, y, z, t ) ∇ × ( F + G )( x, y, z, t ) = ( ∇ × F )( x, y, z, t ) + ( ∇ × G )( x, y, z, t ) ∇ × ( ∇ × F )( x, y, z, t ) = ∇ ( ∇ · F )( x, y, z, t ) − ∆ F ( x, y, z, t ) ∇ · ( F × G )( x, y, z, t ) = G ( x, y, z, t ) · ( ∇ × F )( x, y, z, t ) − − F ( x, y, z, t ) · ( ∇ × G )( x, y, z, t ) Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 10/24

Closed surfaces Definition The surface S is called closed when it is the boundary of some region in R 3 , or equivalently completely contains some region in R 3 . Some examples: 1 Close surface: sphere in R 3 . 2 Open surface: x − y plane. 3 Finite open surface: a disk of radius r < ∞ in R 3 . We are going to assume that our finite open surface S always has a boundary Γ which is closed curve. We always give Γ that particular direction which is such that S enclosed by Γ is on your left when you traverse Γ in this direction. Such boundary curve Γ is called positively oriented . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 11/24

Theorem of Stokes Stokes’ theorem Suppose that S is a finite open surface in R 3 with closed positively oriented boundary curve Γ, and F : R 3 → R 3 is a C 1 -vector field. Then � � F · d r = ( ∇ × F ) · d A . Γ S Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 12/24

Remark Suppose that S 1 and S 2 are two finite open surfaces in R 3 having a common closed positively oriented boundary curve Γ. Then, � � F · d r = ( ∇ × F ) · d A . Γ S 1 and � � F · d r = ( ∇ × F ) · d A . Γ S 2 . Consequently, we obtain � � ( ∇ × F ) · d A = ( ∇ × F ) · d A . S 1 S 2 . This result can be used to replace evaluation of a difficult surface integral with the evaluation of an easier surface integral. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 13/24

Remark (cont.) Suppose we have F : R 3 → R 3 . Let ( x, y, z ) ∈ R 3 be some point and let n be a unit vector pas- sing through ( x, y, z ). Let S ρ be a flat disc of radius ρ with centre at ( x, y, z ) and normal direction n . Finally, let Γ ρ be the closed positively oriented boundary of S . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 14/24

Remark (cont.) From Stokes’ theorem, we have � � F · d r = ( ∇ × F ) · d A . S ρ Γ ρ When ρ is very small, we have � ( ∇ × F ) · d A ≈ ( ∇ × F )( x, y, z ) · n area { S ρ } . S ρ Consequently, we can write 1 � ( ∇ × F )( x, y, z ) · n ≈ F · d r , area { S ρ } Γ ρ and thus 1 � ( ∇ × F )( x, y, z ) · n = lim F · d r . area { S ρ } ρ → 0 Γ ρ Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 15/24

Remark (cont.) Suppose that F is an electric field. We have seen that the “turning” of F around Γ ρ represented by the circulation is actually the electromotive force. In this case, ( ∇ × F )( x, y, z ) · n is the limit of electromotive force per unit area enclosed by Γ ρ as ρ → 0. This interpretation of ∇ × F , when F is an electric field, is very useful in electromagnetism. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 16/24

Outward vs. inward orientation We say that a finite closed surface has outward orientation when the unit vector normal to the surface points out of the enclosed region at every point on the surface. Analogously, a finite closed surface is said to have inward orien- tation when the unit vector normal to the surface points into the enclosed region at every point on the surface Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 17/24

Divergence theorem of Gauss-Ostrogradskii Divergence theorem of Gauss-Ostrogradskii Suppose that S is a closed surface with outward orientation which encloses a finite region Ω ⊂ R 3 , and F : R 3 → R 3 is a C 1 -vector field. Then � � ( ∇ · F ) dV = F · d A . Ω S Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 18/24