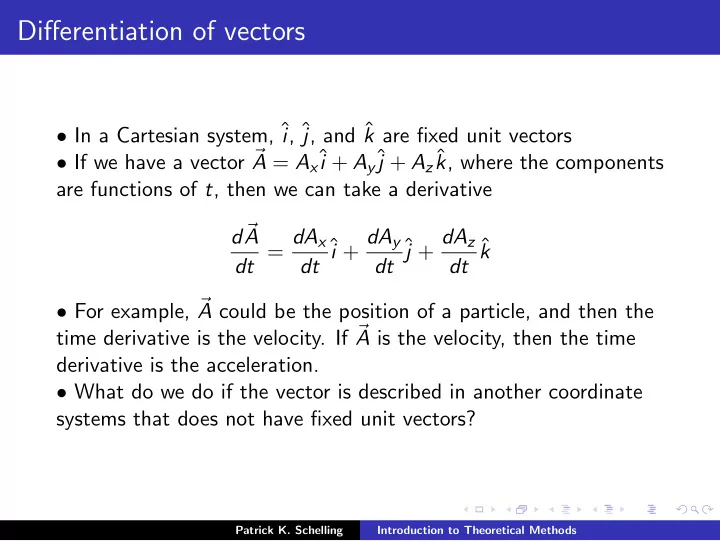
Differentiation of vectors In a Cartesian system, i , j , and k - PowerPoint PPT Presentation

Differentiation of vectors • In a Cartesian system, ˆ i , ˆ j , and ˆ k are fixed unit vectors • If we have a vector � A = A x ˆ i + A y ˆ j + A z ˆ k , where the components are functions of t , then we can take a derivative d � i + dA y dt = dA x A j + dA z ˆ ˆ ˆ k dt dt dt • For example, � A could be the position of a particle, and then the time derivative is the velocity. If � A is the velocity, then the time derivative is the acceleration. • What do we do if the vector is described in another coordinate systems that does not have fixed unit vectors? Patrick K. Schelling Introduction to Theoretical Methods

Differentiation of vectors in a polar system • We can express � A in the xy-plane using unit vectors in a polar system � A = A x ˆ i + A y ˆ j = A r ˆ e r + A θ ˆ e θ • The ˆ i and ˆ j unit vectors have a fixed direction, but ˆ e r and ˆ e θ do not Patrick K. Schelling Introduction to Theoretical Methods

Unit vectors in polar coordinate system expressed in terms of Cartesian system • We can easily see that, e r = cos θ ˆ i + sin θ ˆ ˆ j e θ = − sin θ ˆ i + cos θ ˆ ˆ j • Then computing the time derivatives is straightforward and easy, d ˆ dt = − sin θ d θ e r i + cos θ d θ j = d θ ˆ ˆ dt ˆ e θ dt dt d ˆ dt = − cos θ d θ e θ i − sin θ d θ j = − d θ ˆ ˆ dt ˆ e r dt dt Patrick K. Schelling Introduction to Theoretical Methods

Differentiation of vectors in polar coordinates continued • Now that we have the differentiation of the unit vectors, we can differentiate � A = A r ˆ e r + A θ ˆ e θ d � dt = dA r A e r + dA θ d θ d θ dt ˆ dt ˆ dt ˆ e r + A r dt ˆ e θ − A θ e θ Patrick K. Schelling Introduction to Theoretical Methods

Example: Section 4, problem 8 • Example: In a polar coordinate system, the position vector of a particle is � r = r ˆ e r . Find expressions for the velocity and acceleration. v = d � r • For the velocity, we see that � dt , and then A r = r and A θ = 0, hence v = d � dt = dr r e r + r d θ dt ˆ dt ˆ � e θ a = d 2 � dt 2 , and now A r = dr r • For the acceleration, we see � dt and A θ = r d θ dt , hence � � 2 � a = d 2 � d 2 r + r d 2 θ � d θ � � dr � � d θ � � r � dt 2 = dt 2 − r e r + ˆ 2 ˆ e θ dt 2 dt dt dt Patrick K. Schelling Introduction to Theoretical Methods

Fields • Any physical quantity that varies in space can be taken as a field • Example, the electric field � E ( x , y , z ) is defined in terms of the � electrostatic force on a charge q , � F E = q • In the case of electric field or magnetic field, there is a direction and so we call these vector fields • In the case of electrostatic potential, or gravitational potential, or even temperature or density, we have a scalar field since there is no direction just a magnitude • To describe how these fields behave and the observed phenomena, we need different kinds of differentials of them, which can be called operators • Examples of operators include gradient, divergence, curl, and Laplacian, but there are others Patrick K. Schelling Introduction to Theoretical Methods

Directional derivative and the gradient • Begin by considering a scalar field , for example temperature T ( x , y , z ), or electrostatic potential φ ( x , y , z ) at each point in space • We can pick a direction (not necessarily along the Cartesian coordinate axes!) and determine how φ ( x , y , z ) changes with a small displacement along that direction j + z ˆ r = x ˆ i + y ˆ • Consider a vector � k u = u x ˆ i + u y ˆ j + u z ˆ • Get the direction from a unit vector � k . (Since it is a unit vector. u 2 x + u 2 y + u 2 z = 1) Patrick K. Schelling Introduction to Theoretical Methods

Total differential of a scalar field • Make a displacement of length ds along the � u direction, then j + u z ds ˆ j + dz ˆ � u = u x ds ˆ i + u y ds ˆ k = dx ˆ i + dy ˆ ds = ds � k • We then see the components of the displacement are dx = u x ds , dy = u y ds , and dz = u z ds • Then we can find d φ d φ = ∂φ ∂ x dx + ∂φ ∂ y dy + ∂φ ∂ z dz = ∂φ ∂ x u x ds + ∂φ ∂ y u y ds + ∂φ ∂ z u z ds • The partial derivatives are evaluated at the components of the r = x ˆ i + y ˆ j + z ˆ vector � k Patrick K. Schelling Introduction to Theoretical Methods

Gradient operator • In our calculation of d φ along the vector � ds , we see that it can be described as the scalar product � ∂φ � i + ∂φ j + ∂φ � � ˆ ˆ ˆ u x ds ˆ i + u y ds ˆ j + u z ds ˆ d φ = k · k ∂ x ∂ y ∂ z • We take d φ = ∇ φ · � ds = ds ∇ φ · � u and hence define the gradient operator (in a Cartesian system) ∇ φ = grad φ = ∂φ i + ∂φ j + ∂φ ˆ � ˆ ˆ k ∂ x ∂ y ∂ z • We then define the directional derivative as d φ ds = � ∇ φ · � u Patrick K. Schelling Introduction to Theoretical Methods