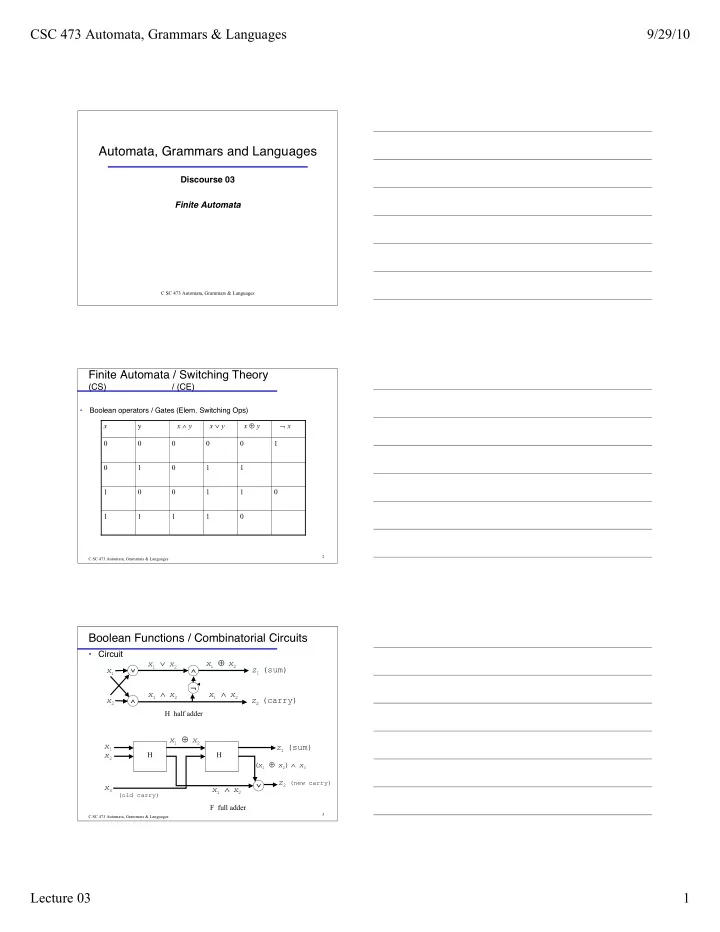

CSC 473 Automata, Grammars & Languages 9/29/10 Automata, Grammars and Languages Discourse 03 Finite Automata C SC 473 Automata, Grammars & Languages Finite Automata / Switching Theory (CS) / (CE) • Boolean operators / Gates (Elem. Switching Ops) x ∧ y x ∨ y x ⊕ y ¬ x x y 0 0 0 0 0 1 0 1 0 1 1 1 0 0 1 1 0 1 1 1 1 0 2 C SC 473 Automata, Grammars & Languages Boolean Functions / Combinatorial Circuits • Circuit � � x x x x 1 2 1 2 x ∨ ∧ z 1 (sum) 1 ¬ � � x x x x 1 2 1 2 x ∧ z 2 (carry) 2 H half adder � x x 1 2 x z 1 (sum) 1 x H H 2 � � ( x x ) x 1 2 3 z 2 (new carry) ∨ x � x x 3 1 2 (old carry) F full adder 3 C SC 473 Automata, Grammars & Languages Lecture 03 1
CSC 473 Automata, Grammars & Languages 9/29/10 Boolean Functions / Comb. Circuits (cont ʼ d) • Table representing F x 1 x 2 x 3 z 1 z 2 0 0 0 0 0 0 0 1 1 0 0 1 0 1 0 0 1 1 0 1 1 0 0 1 0 1 0 1 0 1 1 1 0 0 1 1 1 1 1 1 4 C SC 473 Automata, Grammars & Languages Boolean Functions / Comb. Circuits (cont ʼ d) • Equations representing F = = � � z g x ( ) x x x 1 1 1 2 3 = = � � � � z g x ( ) (( x x ) x ) ( x x ) 2 2 1 2 3 1 2 • General scheme ( n inputs, m outputs) = z g x ( ) = = … … ( , z z , , z ) g x ( , x , , x ) 1 2 m 1 2 n ( g x ( , x , … , x ), … , g x ( , x , … , x )) 1 1 2 n m 1 2 n 5 C SC 473 Automata, Grammars & Languages Finite Automata / Sequential Circuits • Add “memory” elements = delay elements y i + y i ( ) ( 1 ) combinatorial f x z circuit = � � … z i ( ) f x i ( ( ), ( x i 1 ), ( x i 2 ), ) • Finite # of delay elements possible ⇒ ∃ d = � � … z i ( ) f x i ( ( ), ( x i 1 ), x i ( d )) 6 C SC 473 Automata, Grammars & Languages Lecture 03 2
CSC 473 Automata, Grammars & Languages 9/29/10 Finite Automata / Sequential Circuits • Ex: sequential adder: add 2 binary numbers; low x ( 0 ) order bits received first 1001 1 x ( 0 ) 0101 2 1110 • (a) sequential net (circuit): x i ( ) 1 full x i ( ) z i ( ) 2 adder F 1 carry y i + ( 1 ) 1 y i ( ) 1 7 C SC 473 Automata, Grammars & Languages Finite Automata / Sequential Circuits • (b) Next-State & Output Equations: + = � � � � y i ( 1 ) (( ( ) x i x i ( )) y i ( )) ( ( ) x i x i ( )) 1 1 2 1 1 2 = � � z i ( ) x i ( ) x i ( ) y i ( ) 1 1 2 1 = = • (c) Transition Table: state space { 0 ,1 } { , q q } 0 1 � input alphabet ={(00),(01),(10),(11)} � output alphabet ={0,1} next state/output table x ( 00 ) ( 01 ) ( 10 ) ( 11 ) q q q 0 / 0 q 0 / 1 q 0 / 1 q 1 / 0 0 q q 0 / 1 q 1 / 0 q 1 / 0 q 1 / 1 1 8 C SC 473 Automata, Grammars & Languages Finite Automata / Sequential Circuits • (d) State Diagram: ( 01 )/ 0 ( 00 )/ 0 ( 11 )/ 0 ( 11 )/ 1 q q ( 01 )/ 1 1 0 ( 00 )/ 1 ( 10 )/ 1 ( 10 )/ 0 9 C SC 473 Automata, Grammars & Languages Lecture 03 3
CSC 473 Automata, Grammars & Languages 9/29/10 Finite Automata / Sequential Circuits • (e) Finite-State Transducer (Mealy Machine) = � � � M ( , , , , Q q ) A 5-tuple where 0 = Q { q , q } finite set of states 0 1 q start state 0 � = {( 00 ),( 01 ),( 10 ),( 11 )} input alphabet � = { 0 ,1 } output alphabet � � � � � � : Q Q transition/output function � = � = ( ,( q 00 )) ( ,0 q ) ( ,( q 01 )) ( ,1 q ) 0 0 0 0 � = � = ( ,( q 10 )) ( ,1 q ) ( ,( q 11 )) ( ,0 q ) 0 0 0 1 � = ( ,( q 01 )) ( ,0 q ) etc . 1 1 10 C SC 473 Automata, Grammars & Languages General Sequential Network B = { 0 ,1 } δ is a z x boolean 1 1 functio � + n s � � : B n x z m n + � m s B state space = s y B 1 … � y s � = + ( ( ), ( )) y i x i ( ( y i 1 ), ( )) z i � = � ( , q a ) ( , q b ) 11 C SC 473 Automata, Grammars & Languages Three Types of Automata � � a i a � a a a � c c c c n 3 2 1 n 3 2 1 M time transducer a � a a a n 3 2 1 Yes(1) M No(0) recognizer (acceptor) c � c c c n 3 2 1 M Enumerator (generator) 12 C SC 473 Automata, Grammars & Languages Lecture 03 4
CSC 473 Automata, Grammars & Languages 9/29/10 Machines that Recognize • Detection of an “event”, i.e., a pattern in input • Recognition of just those words in some language L • Definition of a language • Ex: detect abab –all non-overlapping occurrences b b a a b a b a b a 13 C SC 473 Automata, Grammars & Languages Ex: C Comments /* … */ � � � = a {,/} • Filter in the lexical scanner empty (transducer) aa : notation � / : / / : a � � � : : � � in : out : � � � : / : � � : a � : a a : / � / : • Recognizer / a � / � � / a 14 C SC 473 Automata, Grammars & Languages Finite Automaton (Finite State Machine, FSA) • Defn 1.5: A (deterministic) finite automaton is a 5-tuple = � � M ( , , , Q q , F ) 0 Q is a finite set, the states • � is a finite set, the alphabet • � � � � : Q Q is the transition function • � q Q is the start state • 0 � F Q is the set of accepting (final) states • = = � � Q { , q q , q } , F • Ex: M ( , , , Q q ) 0 0 1 2 0 � = = { , } a b F { } q 1 a � = � = ( , ) q a q ( , ) q b q q q 0 1 0 2 1 0 b � = � = ( , ) q a q ( , ) q b q 1 2 1 0 M a b � = � = 0 ( , ) q a q ( , ) q b q 2 2 2 2 q 2 a b , 15 C SC 473 Automata, Grammars & Languages Lecture 03 5
CSC 473 Automata, Grammars & Languages 9/29/10 How FA Compute = � � , F • FA is a finite structure—like M ( , , , Q q ) 0 a program—fixed and static • Need to define the behavior of M on input w Sequence of configurations Like trace of a program on given data Dynamic and input-dependent = M w ababa • Ex: start on input Look at sequence 0 of “moves” determined by the transition function: ( q 0 , ababa ) � ( q 1 , baba ) � ( q 0 , aba ) � q 1 , � ) ( q 1 , ba ) � ( q 0 , a ) ( � � ( q 0 , ababa ) � � ( q 1 , � ) Since in accepting state when input exhausted, w is recognized by M 0 16 C SC 473 Automata, Grammars & Languages www.jflap.org JFLAP is a package of graphical tools which can be used as an aid in learning the basic concepts of Formal Languages and Automata Theory. C SC 473 Automata, Grammars & Languages Info on JFLAP • Website http://www.jflap.org/ Downloads Tutorial • Lectura (linux) install cd /usr/local/jflap java -jar JFLAP.jar • X11 forwarding (graphics) ssh -X lectura 18 C SC 473 Automata, Grammars & Languages Lecture 03 6
CSC 473 Automata, Grammars & Languages 9/29/10 How FA Compute (cont ʼ d) = � � , F • Given a FA M ( , , , Q q ) 0 � � � Q • Defn: configuration of M is an element of ! • Defn: yields in one step (or moves) relation between configurations is defined by ( � q , w ) � � ( q , a ) = � ( q , aw ) � q � � � � � � � a , w , , q q Q where q , � ) ( Notes: is a function, since δ is. Is undefined. � � � � • Defn: yields is the relation Means “moves in zero or more steps to” q , w ) � � ( ( q , w ) • Defn: A string w is recognized (accepted) by M ⇔ q 0 , w ) � � ( ( � f � F )( f , � ) 19 C SC 473 Automata, Grammars & Languages How FA Compute (cont ʼ d) • Defn: The language recognized (accepted) by M is ( M ) = { L w : M accepts w } = { w :( � f � F )( � � ( f , � ) q 0 , w ) } • Defn 1.16: A language S is regular iff there is some FA � � = that recognizes it, i.e., ( M )[ is a FA M S L M ( )] q 0 , a ) � � ( M q 1 , � ) • Ex: In FA ( 0 � � ( q 1 , � ) ( q 0 , aba ) � i a ) � � ( q 1 , � ) ( q 0 , ( ab ) � L ( M 0 ) = {( i a : i � 0 ab ) } 20 C SC 473 Automata, Grammars & Languages Example: i = make change for i – 30 ¢ & vend coffee • Coin checker for 30¢ coffee. Σ = { n,d,q } d n 0 d 50 5 n q q 10 q 45 n d q 15 40 q n 20 q 35 n d 25 30 n d d 21 C SC 473 Automata, Grammars & Languages Lecture 03 7
Recommend
More recommend