CG Configuration = + C C C Assumption: r o is large. 1 1 D - PowerPoint PPT Presentation

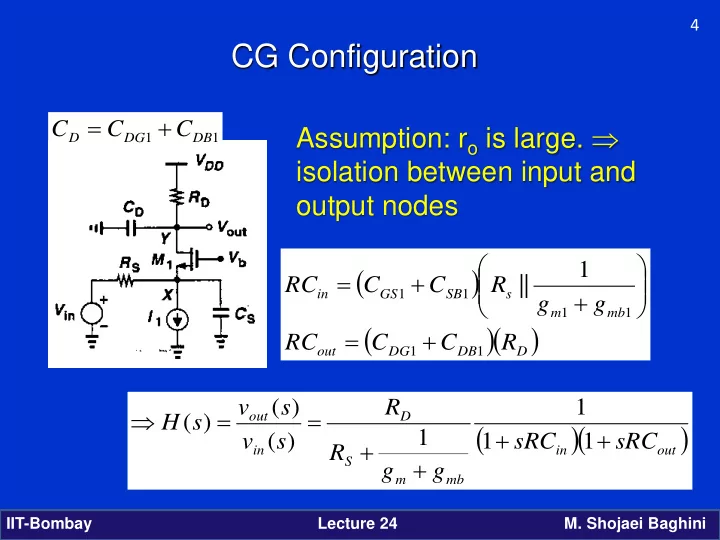
4 CG Configuration = + C C C Assumption: r o is large. ⇒ 1 1 D DG DB isolation between input and output nodes   1 ( )   = + || RC C C R   + 1 1 in GS SB s   g g 1 1 m mb ( )( ) = + RC C C R 1 1 out DG DB D ( ) 1 v s R ⇒ = = ( ) out D H s ( )( ) + + 1 ( ) 1 1 v s sRC sRC + R in in out + S g g m mb IIT-Bombay Lecture 24 M. Shojaei Baghini

5 Precise H(s): CG Configuration (If r o is not negligible but R L is negligible) ( ) + + ( ) 1 v s g g r = out m mb o [ ] ( ( ) ) + + + + + + 2 ( ) 1 v s R r C C s r R g g r R C C R s in s o L in o s m mb o s L in s IIT-Bombay Lecture 24 M. Shojaei Baghini

6 CG Configuration (If r o is not negligible) R L + 1 Z r = ≈ L o || Z ( ) + + in 1 sC g g r in m mb o   1 1 Z   + L ||   ( ) + +   sC g g g g r in m mb m mb o Z = in v v Pole estimation at the input , + s M in 1 R Z s in IIT-Bombay Lecture 24 M. Shojaei Baghini

7 Interesting Approximation for the Second Pole of CG Configuration ( ) + + ( ) 1 v s g g r = out m mb o [ ] ( ( ) ) + + + + + + 2 ( ) 1 v s R r C C s r R g g r R C C R s in s o L in o s m mb o s L in s ( ) R r C C C τ ≈ + ⇒ τ ≈ = s o L in in : If g g r R C ( ) + + 1 2 m mb o s L g g r R C g g m mb o s L m mb Simple isolated time constant at the input (pole assigned to the input node) and τ 1 >> τ 2 IIT-Bombay Lecture 24 M. Shojaei Baghini

8 Frequency Response of Cascode Amplifier Very difficult to use direct calculation! We use property of CG configuration, given in the previous slide. IIT-Bombay Lecture 24 M. Shojaei Baghini

9 Approximation in the Frequency Response of Cascode Amplifier ( ) τ ≈ || R R C , out out D out eq = + + C C C C , 2 2 out eq BD GD L − ( ) v s g ≈ = − ⇒ 1 X m A + 0 ( ) v s g g 2 2 A m mb   g   = + + 1 m 1 C C C   + , 1 1 in eq GS GD   g g 2 2 m mb ⇒ τ ≈ • What about the pole R C , in s in eq at node X? • Worst case estimation IIT-Bombay Lecture 24 M. Shojaei Baghini

4 Related to the assignment (approximations and validity of time constant method) IIT-Bombay Lecture 25 M. Shojaei Baghini

5 DM Frequency Response of Differential Amplifier Differential-mode half circuit IIT-Bombay Lecture 25 M. Shojaei Baghini

6 CM Frequency Response of Differential Amplifier • Impact of mismatch 1 2 1 2 • Tradeoff between voltage headroom and CM frequency response IIT-Bombay Lecture 25 M. Shojaei Baghini