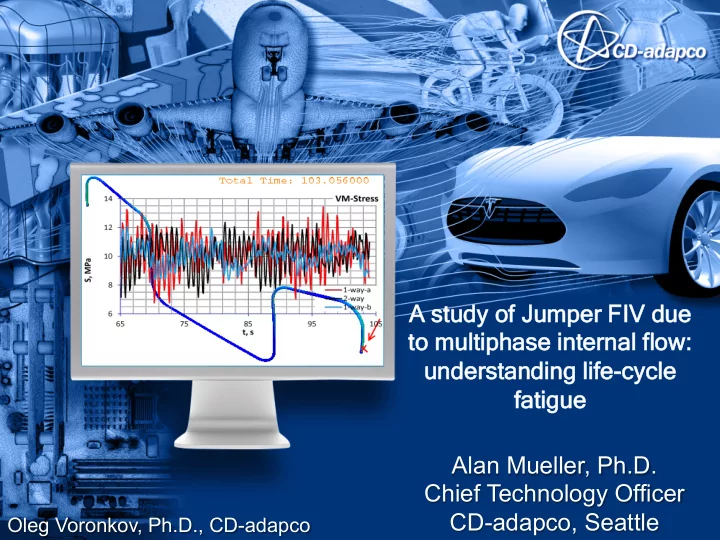

A A st study y of Ju Jump mper r FIV V due to mu mult ltip iphase se in intern rnal l flo low: underst rstandin ing lif life-cycle -cycle fatig igue Alan Mueller, Ph.D. Chief Technology Officer CD-adapco, Seattle Oleg Voronkov, Ph.D., CD-adapco
Case d description [2] deformable jumper Main structural dimensions [1]: in Mixture on inlet out endings top half - air bottom half - water Case set-up: 1) water-air (50/50 % volume) mixture flow inside jumper; 2) the deformable jumper is clamped on its ends; 3) outside water is accounted as added mass and added damping. [1] L. Chica. Fluid Structure Interaction Analysis of Two-Phase Flow in an M-shaped Jumper. University of Houston, College of [1] Technology, Mechanical Engineering Technology. Star Global Conference 2012. January 28, 2012. [2] Tie-in and structures. Brochure. Aker Solutions, 2010. [2]
Appli lied p phys ysics & & c cha haracteristic p parame meters Fluid side (STAR-CCM+): Structural side (Abaqus): Step options: Models: Implicit, 2-order time; Segregated flow, 2-order time; Non-Linear Geometry (not a Eulerian Multiphase; requirement) VOF; URANS k-omega SST turbulence; Geometric parameters: Gravity: [0,0,-1g]. Circular pipe; Flow parameters: D out = 10.75’’ (0.273 m); V in = 3.048 m/s; D in = 8.25’’ (0.210 m). water (incompressible): Mechanical characteristics: µ = 0.001 Pa · s; Steel: ρ = 1000 kg/m 3 ; E = 205 GPa; Re(D in , V in ) = 6.4e+5; ν = 0.29; air (ideal gas, isothermal): ρ = 7800 kg/m 3 . µ = 2e-5 Pa · s; ρ (P = 0) = 1.2 kg/m 3 ; Multi-phase internal flow leads to Re(Din, Vin) = 3.8e+4; range of forcing frequencies not ρ air / ρ water = 1.2e-3; found in single phase flows µ air / µ water = 2e-2.
Possible le c couple led s solu lution a n approache hes 1-way coupled 2-way coupled Ø transient forces from the fluid solution are Ø forces from the fluid side are transmitted to the structural solution; transmitted to the structural side and displacements of the structure Ø don’t account for vibration of the structure in are passed back to the fluid side; the fluid solution; Ø requires implicit coupling for Ø requires application of added mass and stability; damping for internal vibrations; Ø added mass and damping from internal flow is applied in a natural manner as a reaction for movement Damping is Damping is of the structure; two applied as applied as variants mass stiffness proportional proportional Ø computationally more expensive; Ø commonly used practice; Ø potentially more accurate; Ø needs minimum computational effort; Ø how large is the difference in effort & accuracy from the 1-way Ø how to estimate the reasonable amount of the coupled? added damping due to multiphase flow
Abaqus S Stand ndalo lone ne A Ana nalys lysis: S : Stiffne ness Static a ana nalys lysis t to e evalu luate s stiffne ness – 21K shell elements (S4), 126K DoF BCs: clamped ends
Abaqus S Stand ndalo lone ne A Ana nalys lysis: S : Stiffne ness in X direction 2 forces (500 N each) applied def. x1000 in corresponding direction in Y direction X Y Z St., N/mm 575 56.5 489 def. x100 in Z direction Considerably weaker in Y (cross) direction def. x1000
Abaqus S Stand ndalo lone ne A Ana nalys lysis: N : Natural M l Modes Eigenvalu lue a ana nalys lysis t to e evalu luate mo mode s sha hapes a and nd f fund ndame ment ntal f l frequenc ncies Accounted mass: 1) structural mass (M s ); 2) mass of internal mixture (M im ): in assumption of uniform 50/50% air/water vol. fraction; 3) added mass of surrounding water: M e /M w = m* + Ca, m* = (M s + M im )/M w , Ca ≈ 1 [3], [4], M w – mass of displaced water; M e – effective structural mass mode #3 – Y mode #2 – Z mode #1 – Y [3] J.P. Pontaza, B. Abuali, G.W. Brown, F.J. Smith. Flow-Induced Vibrations of Subsea Piping: A Screening Approach Based on Numerical Simulation. Shell International Exploration and Production Inc., Shell U.K. Limited. SPE 166661. 2013. [4] J.P. Pontaza, R.G. Menon. Flow-Induced Vibrations of Subsea Jumpers due to Internal Multi-Phase Flow. Shell Projects & Technology. OMAE2011-50062.
Structural mo l mode s sha hapes & & na natural f l frequenc ncies Natural frequencies: Mode 1 2 3 4 5 6 7 8 … f, Hz 0.59 1.30 1.70 1.77 2.06 2.07 2.82 6.07 Considered in dynamic simulations mode #4 – X, Z mode #5 – Y mode #7 – Y mode #6 – X, Z
STAR-C -CCM+ V VOF S Stand ndalo lone ne Structure i is r rigid 4.2M polyhedral cells; VOF Generalized Cylinder Mesh – Considered Eulerian Multiphase, but not as stable) Spatial r l resolu lution s n sufficient nt t to r reasona nably c ly capture w water/air i int nterface s surface Y+ ne near 1 1 t to r resolv lve w wall e ll effects Time me s step li limi mitation : f n : free s surface mo moves le less t tha han 1 n 1 c cell i ll in 1 n 1 t time me s step – Δ t f ≈ T 7 /50 (small fraction of highest mode considered) – Larger times step possible but leads to significant numerical diffusion Pressure Outlet Wall no slip Velocity Inlet
Flu luid S Stand ndalo lone ne: V : Volu lume me F Fraction a n and nd P Pressure Do Domi mina nate F Frequenc ncy 0 y 0.0 .09 H Hz < << M Mode 1 1 F Frequenc ncy 0 y 0.5 .59 H Hz Pressure
1-way coupled Flu luid – Same setup as in fluid standalone Δ t f ≈ T 7 /50 St Structure – Structure resolution as in structure standalone – Same added mass for internal and external flow as in Eigenmode analysis – Time step chosen to resolve well 7 th mode Δ t=T 7 /60 – External fluid added damping – Internal fluid added damping • To c correct f for r rigid a assumption i n in f n flu luid mo model l Expli licit C Coupli ling ng – Fluid loads to structure onc nce p per f flu luid t time me s step Simu mula lation o n over 1 10 f fund ndame ment ntal f l flu luid f forcing ng p periods
2-way coupled Flu luid – Same conditions as in 1-way except fluid time step same as solid time step: Δ t f =T 7 /60 St Structure – Same as 1-way but no added mass and damping for internal flow Im Impli licit C Coupli ling ng – Data Exchange onc nce p per i iteration i n in a n a t time me s step • Fluid loads sent to structure • Structure displacements sent to fluid – Fluid and structure use identical time steps – Move to next time step when both structure and fluid residuals converge Simu mula lation o n over 1 10 f fund ndame ment ntal f l flu luid f forcing ng p periods Under the stated conditions, the primary difference between 1-way and 2-way coupling: 2-way coupling requires more iterations within a time step
One ne-Way C y Coupli ling ng : Int : Interna nal Da l Damping ng Cho hoice o of Da Damping ng – Mass Proportional C= α *M – Stiffness Proportional C= β *K Size Size α , β to g give d damping ng i in M n Mode 1 1 as me measured i in 2 n 2-w -way c y coupli ling ng – ln(D) = 0.09 => ξ = ln(D)/sqrt[4 · pi 2 + ln(D) 2 ] Stiffness proportional: 2 ξ = βω Mass proportional: 2 ξ = α / ω Mode 1 2 3 4 5 6 7 0.59 1.30 1.70 1.77 2.06 2.07 2.82 f, Hz α =0.106Hz ξ : 1.43e-2 6.50e-3 4.97e-3 4.77e-3 4.10e-3 4.08e-3 3.00e-3 β =7.71e-3s ξ : 1.43e-2 3.16e-2 4.13e-2 4.30e-2 5.00e-2 5.03e-2 6.85e-2
Water v volu lume me f fraction ( n (section a n averaged) 2 6 1 5 ji jo 4 3 Dominant (slug) frequencies, Hz # 1 2 3 4 5 6 f, Hz 0.089 0.18 0.28 0.37 0.46 0.54
Water v volu lume me f fraction: e n: evolu lution o n of s slu lugs bend before 2 nd lift: 1 st lift: formation of long slugs short slugs (1 … 3)D in long bend after 2 nd lift: long slugs ~(12 … 15)D in
Water v volu lume me f fraction 1-way coupled 2-way coupled cs #4 cs #4 cs #5 cs #5
12 2 Pipe v vibrations ns 10 4 Ux FFT (Ux), [25 s, tmax] 8 6 Fluid force
12 2 Pipe v vibrations ns 10 4 Uz FFT (Uz), [25 s, tmax] 8 6
12 2 Pipe v vibrations ns 10 4 Uy FFT (Uy), [25 s, tmax] 8 6
Pipe v vibrations ns 12 11 3 2 10 4 1 13 8 7 6 5 9 Dominant frequencies, Hz Mode - 1 2 3 4 5, 6 7 U (x200) U1-x 0.089 - 1.32 - 1.7-1.8 2.04 - U2-y 0.089 0.56 - 1.6-1.7 - 2.06 2.77 U3-z 0.089 - 1.32 - - 2.04 -
Stress s signa nal Def.: U (x200); Field: VM stress σ max (tension), max. amplitude Input for fatigue life calculation
Fatigue li life e estima mate Rain flow counting results S-N curve [5] 1 repetition = ~79 s Applied modifications: thickness effect; stress gradient (bending); surface roughness. Pa Palmg lmgre ren-Min -Miner r ru rule le to co comp mpute the dama mage and lif life est stima imate [5] Guide for the fatigue assessment of offshore structures. American Bureau of Shipping, Houston, TX, USA. November 2010.
Calc lcula lation e n efforts STAR-CCM+ v.9.02.002: 52 cpus: 82k cells per cpu; ABAQUS v.6.13-1: 4 cpu: 31.5k dof per cpu; Total effort: 18400 steps; 2-way coupled: 13 days (61.5 sec/step); 1-way coupled: 6.5 days (30.6 sec/step).
Recommend
More recommend