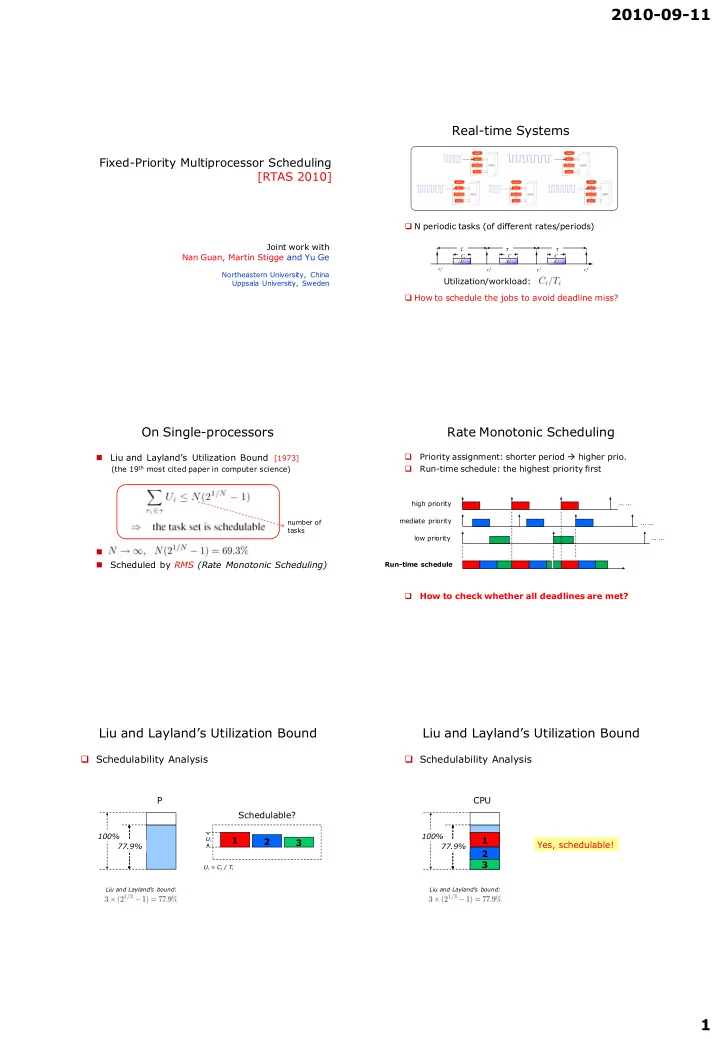
2010-09-11 Real-time Systems Fixed-Priority Multiprocessor - PDF document

2010-09-11 Real-time Systems Fixed-Priority Multiprocessor Scheduling [RTAS 2010]  N periodic tasks (of different rates/periods) Joint work with T T T i i i Nan Guan, Martin Stigge and Yu Ge C i C C J i1 J i2 J i3 i i r i1 r i2 r i3 r i4 Northeastern University, China Utilization/workload: Uppsala University, Sweden  How to schedule the jobs to avoid deadline miss? On Single-processors Rate Monotonic Scheduling Liu and Layland’s Utilization Bound [1973] Priority assignment: shorter period  higher prio.   (the 19 th most cited paper in computer science) Run-time schedule: the highest priority first  high priority … … mediate priority number of … … tasks … … low priority   Scheduled by RMS (Rate Monotonic Scheduling) Run-time schedule How to check whether all deadlines are met?  Liu and Layland’s Utilization Bound Liu and Layland’s Utilization Bound  Schedulability Analysis  Schedulability Analysis P CPU Schedulable? 100% 100% U i 1 1 2 3 Yes, schedulable! 77.9% 77.9% 2 3 U i = C i / T i Liu and Layland’s bound: Liu and Layland’s bound: 1

2010-09-11 Multiprocessor (multicore) Scheduling Open Problem (since 1973)  Significantly more difficult:  Find a multiprocessor scheduling algorithm that can achieve Liu and Layland ’ s utilization bound  Timing anomalies ?  Hard to identify the worst-case scenario Bin-packing/NP-hard problems  number of Multiple resources e.g. caches, bandwidth  processors  … … Multiprocessor Scheduling Best Known Results (before 2010) Partitioned Scheduling Global Scheduling Partitioned Scheduling with Task Splitting new task 4 waiting queue 2 3 4 2 2 2 2 2 3 2 5 5 8 9 5 3 7 1 6 7 1 6 4 8 1 6 cpu 2 cpu 3 cpu 2 cpu 3 cpu 2 cpu 3 cpu 1 cpu 1 cpu 1 Best Known Results (before 2010) Best Known Results Our New Result % 80 Liu and Layland ’ s RTAS 2010 Utilization Bound 70 RTSS 2010_submitted 60 69.3 50 40 65 66 30 50 50 50 [ RTCSA’ 06] 20 38 [ TPDS’ 05] [ ECRTS’ 03] [ RTSS’ 04] [ OPODIS’ 08] 10 Fixed Dynamic Fixed Dynamic Fixed Dynamic Priority Priority Priority Priority Priority Priority Task Splitting Global Partitioned Lehoczky et al. CMU ECRTS 2009 Multiprocessor Scheduling 2

2010-09-11 Multiprocessor Scheduling Multiprocessor Scheduling Global Scheduling Global Scheduling Would fixed-priority scheduling Would fixed-priority scheduling e.g. “RMS” work? e.g. “RMS” work? new task new task 4 4 Unfortunately “RMS” suffers waiting queue waiting queue from the Dhall’s anomali 2 5 2 5 Utilization may be “0%” 8 8 1 6 1 6 cpu 2 cpu 3 cpu 2 cpu 3 cpu 1 cpu 1 Dhall’s anomali Dhall’s anomali Task 1 CPU1 1 1 1 1 Task 2 CPU2 2 2 2 3 3 2 Deadline miss Task 3 3 ε 1 1+ ε ε 1 1+ ε 0 0 Schedule the 3 tasks on 2 CPUs using “RMS Dhall’s anomali Multiprocessor Scheduling (M+1 tasks and M processors) Partitioned Scheduling 1/( ε +1) ε /1 ε /1 ε /1 #M+1 2 3 4 #1 #2 … … #M 5 8 9 P 1 P 2 P M 1 6 7 M* ε + 1/(1+ ε ) U 0 M … … cpu 1 cpu 2 cpu 3 3

2010-09-11 Multiprocessor Scheduling Partitioned Scheduling  The Partitioning Problem is similar to Bin-packing Problem (NP-hard) Partitioned Scheduling Resource utilization may  Limited Resource Usage, 50% necessary condition to be limited to 50% guarantee schedulability 2 3 4 #1 #2 … … #M #M+1 50%+ ε 5 8 9 1 6 7 P 1 P 2 P M … … cpu 2 cpu 3 cpu 1 Partitioned Scheduling Partitioned Scheduling  The Partitioning Problem is similar to  The Partitioning Problem is similar to Bin-packing Problem (NP-hard) Bin-packing Problem (NP-hard)  Limited Resource Usage  Limited Resource Usage necessary condition to necessary condition to guarantee schedulability guarantee schedulability #M+1,1 … … #M+1 50%+ ε … … 50%+ ε #M+1,2 P 1 P 2 P M P 1 P 2 P M … … … … #1 #2 #M #1 #2 #M Partitioned Scheduling Multiprocessor Scheduling  The Partitioning Problem is similar to Bin-packing Problem (NP-hard) Partitioned Scheduling with Task Splitting  Limited Resource Usage necessary condition to guarantee schedulability 2 2 2 2 2 3 … … 50%+ ε 5 3 7 1 6 4 P 1 P 2 P M #M+1,1 #M+1,2 … … cpu 1 cpu 2 cpu 3 #1 #2 #M 4

2010-09-11 Partitioned Scheduling Bin-Packing with Item Splitting  Partitioning  Resource can be “fully” (better) utilized 2 1 1 1 3 6 4 5 9 7 8 P1 P2 P3 Bin1 Bin2 Bin3 8 1 1 1 6 5 2 7 4 3 8 2 1 2 Previous Algorithms Lakshmanan ’s Algorithm [ECRTS’09] [Kato et al. IPDPS’08] [Kato et al. RTAS’09] [ Lakshmanan et al. ECRTS’09] Sort the tasks in some order e.g. utilization or priority order  Sort all tasks in decreasing order of utilization   Select a processor, and assign as many tasks as possible P1 8 8 highest util. 7 7 6 6 5 5 4 4 3 3 2 2 1 1 lowest util. Lakshmanan ’s Algorithm [ECRTS’09] Lakshmanan ’s Algorithm [ECRTS’09]  Pick up one processor, and assign as many  Pick up one processor, and assign as many tasks as possible tasks as possible P1 P1 8 highest util. highest util. 7 7 6 6 8 5 5 4 4 3 3 2 2 1 1 lowest util. lowest util. 5

2010-09-11 Lakshmanan ’s Algorithm [ECRTS’09] Lakshmanan ’s Algorithm [ECRTS’09]  Pick up one processor, and assign as many  Pick up one processor, and assign as many tasks as possible tasks as possible P1 P1 highest util. highest util. 6 1 7 7 6 8 8 6 2 5 5 4 4 3 3 2 2 1 1 lowest util. lowest util. Lakshmanan ’s Algorithm [ECRTS’09] Lakshmanan ’s Algorithm [ECRTS’09]  Pick up one processor, and assign as many  Pick up one processor, and assign as many tasks as possible tasks as possible P1 P2 P1 P2 highest util. highest util. 6 1 6 1 7 7 8 8 6 2 6 2 5 5 4 4 3 3 2 2 1 1 lowest util. lowest util. Lakshmanan ’s Algorithm [ECRTS’09] Lakshmanan ’s Algorithm [ECRTS’09]  Pick up one processor, and assign as many  Pick up one processor, and assign as many tasks as possible tasks as possible P1 P2 P1 P2 highest util. highest util. 6 1 6 1 7 7 4 5 5 8 8 6 2 6 2 4 3 3 2 2 1 1 lowest util. lowest util. 6

2010-09-11 Lakshmanan ’s Algorithm [ECRTS’09] Lakshmanan ’s Algorithm [ECRTS’09]  Pick up one processor, and assign as many  Pick up one processor, and assign as many tasks as possible tasks as possible P1 P2 P1 P2 highest util. highest util. 2 1 6 1 6 1 3 3 7 7 4 4 5 5 8 8 6 2 6 2 2 2 2 1 1 lowest util. lowest util. Lakshmanan ’s Algorithm [ECRTS’09] Lakshmanan ’s Algorithm [ECRTS’09]  Pick up one processor, and assign as many  Pick up one processor, and assign as many tasks as possible tasks as possible P1 P2 P3 P1 P2 P3 highest util. highest util. 2 1 2 1 6 1 6 1 3 3 7 7 4 4 1 5 5 8 8 2 2 2 2 6 2 6 2 1 lowest util. lowest util. Lakshmanan ’s Algorithm [ECRTS’09] Lakshmanan ’s Algorithm [ECRTS’09]  Pick up one processor, and assign as many  Pick up one processor, and assign as many tasks as possible tasks as possible P1 P2 P3 P1 P2 P3 highest util. highest util. 2 1 2 1 6 1 6 1 3 3 7 7 4 4 1 1 5 5 8 8 2 2 2 2 6 2 6 2 key feature: Utilization Bound: “depth - first” partitioning with decreasing utilization order 65% lowest util. lowest util. 7

2010-09-11 Our Algorithm Our Algorithm  Sort all tasks in increasing priority order [RTAS10] 7 lowest priority “width - first” partitioning 6 with increasing priority order 5 4 3 2 1 highest priority Our Algorithm Our Algorithm  Select the processor on which the assigned  Select the processor on which the assigned utilization is the lowest utilization is the lowest 7 lowest priority lowest priority 6 6 5 5 P1 P2 P3 P1 P2 P3 4 4 3 3 7 2 2 1 1 highest priority highest priority Our Algorithm Our Algorithm  Select the processor on which the assigned  Select the processor on which the assigned utilization is the lowest utilization is the lowest lowest priority lowest priority 5 P1 P2 P3 P1 P2 P3 4 4 3 3 7 7 5 6 6 2 2 1 1 highest priority highest priority 8

2010-09-11 Our Algorithm Our Algorithm  Select the processor on which the assigned  Select the processor on which the assigned utilization is the lowest utilization is the lowest lowest priority lowest priority P1 P2 P3 P1 P2 P3 3 3 4 4 7 7 5 5 6 6 2 2 1 1 highest priority highest priority Our Algorithm Our Algorithm  Select the processor on which the assigned  Select the processor on which the assigned utilization is the lowest utilization is the lowest lowest priority lowest priority P1 P2 P3 P1 P2 P3 2 1 2 1 2 2 3 3 4 4 7 7 5 5 6 6 2 2 1 1 highest priority highest priority Our Algorithm Our Algorithm  Select the processor on which the assigned  Select the processor on which the assigned utilization is the lowest utilization is the lowest lowest priority P1 P2 P3 P1 P2 P3 1 2 1 1 1 1 2 1 2 1 2 2 3 2 2 3 4 4 7 7 5 5 6 6 highest priority 1 2 9