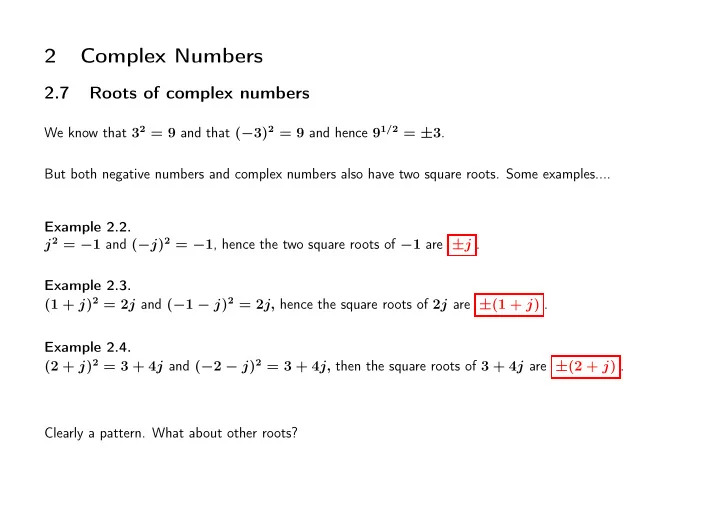
2 Complex Numbers 2.7 Roots of complex numbers We know that 3 2 = - PowerPoint PPT Presentation

2 Complex Numbers 2.7 Roots of complex numbers We know that 3 2 = 9 and that ( − 3) 2 = 9 and hence 9 1 / 2 = ± 3 . But both negative numbers and complex numbers also have two square roots. Some examples.... Example 2.2. j 2 = − 1 and ( − j ) 2 = − 1 , hence the two square roots of − 1 are ± j . Example 2.3. (1 + j ) 2 = 2 j and ( − 1 − j ) 2 = 2 j, hence the square roots of 2 j are ± (1 + j ) . Example 2.4. (2 + j ) 2 = 3 + 4 j and ( − 2 − j ) 2 = 3 + 4 j, then the square roots of 3 + 4 j are ± (2 + j ) . Clearly a pattern. What about other roots?

How about? Example 2.5. (1 + j ) 4 = − 4 , ( − 1 − j ) 4 = − 4 , (1 − j ) 4 = − 4 ( − 1 + j ) 4 = − 4 . and Hence − 4 has four fourth roots: ( − 4) 1 / 4 = ± 1 ± j where all four possible combinations of plus/minus may be taken. Example 2.6. (1 + 2 j ) 4 = − 7 − 24 j, ( − 1 − 2 j ) 4 = − 7 − 24 j, (2 − j ) 4 = − 7 − 24 j ( − 2 + j ) 4 = − 7 − 24 j. and Therefore the four fourth roots of ( − 7 − 24 j ) are ± (1 + 2 j ) and ± (2 − j ) . Presumably this works too for 8 th , 16 th roots etc....

Example 2.7. √ √ ( − 2) 3 = − 8 , 3 j ) 3 = − 8 3 j ) 3 = − 8 . (1 + and (1 − So we have three third roots of − 8 , namely √ √ − 2 , 1 + 3 j and 1 − 3 j . Example 2.8. If one were to find the sixth powers of √ √ √ √ 2 , − 2 , 1 + 3 j , 1 − 3 j , − 1 + 3 j , − 1 − 3 j , then each would yield 64 . So 64 has six sixth roots. So this suggests a pattern: there are n different n th roots for a complex number. However, that is not the only pattern.

Im Im Im • 1 + j − 1 + j • 1 + j • • 2 + j √ √ √ 2 5 2 Re Re Re • − 2 − j • • • 1 − j − 1 − j − 1 − j Example 2.3. (2 j ) 1 / 2 Example 2.4. (3 + 4 j ) 1 / 2 Example 2.5. ( − 4) 1 / 4 Im Im Im √ √ √ • 1 + 2 j − 1 + 3 j • 1 + 3 j • 1 + 3 j • 2 − j • √ − 2 − 2 5 2 • 2 • • Re Re Re • 2 − j • • • • √ √ √ − 1 − 2 j 1 − 3 j 1 − 3 j − 1 − 3 j Example 2.6. ( − 7 − 24 j ) 1 / 4 Example 2.7. ( − 8) 1 / 3 Example 2.8. 64 1 / 6 Figure 2.5. Displaying the roots corresponding to Examples 2.3 to 2.8.

Im − 1 + j • 1 + j • √ 2 Re • • 1 − j − 1 − j Example 2.5. ( − 4) 1 / 4 In all cases the circle is split into equal segments. The general method (illustrated, but working backwards....) The four roots in Ex. 2.5 are, √ √ √ √ 2 e jπ/ 4 , 2 e 3 jπ/ 4 , 2 e 5 jπ/ 4 2 e 7 jπ/ 4 . and and their 4 th powers are 4 e jπ , 4 e 3 jπ , 4 e 5 jπ 4 e 7 jπ , and These are all − 4 , i.e. the same place in the complex plane, but separated by 2 π : 4 e (1+2 n ) πj , n = 0 , 1 , 2 , 3 ,

Im • 1 + j √ 2 Re • − 1 − j Example 2.3. (2 j ) 1 / 2 Example 2.9. Find the square roots of 2 j . [N.b. slightly different from the printed notes.] 2 j = 2 e ( π/ 2) j 2 j = 2 e ( π/ 2) j , 2 e (5 π/ 2) j , Let or as 2 j = 2 e ( π/ 2+2 nπ ) j , or more generally as where n = 0 , 1 . √ √ � 2 e ( π/ 4) j 2 e (5 π/ 4) j . Now take the square roots: 2 j = or For this particular example we can write the Cartesian form without the use of a calculator! √ √ √ 2 j � � � � cos 1 4 π + j sin 1 cos 5 4 π + j sin 5 = 2 4 π or 2 4 π = 1 + j, − 1 − j.

Example 2.10. Find the fifth roots of (3 + 4 j ) . In polar form we have = 5 e θj , 5 e ( θ +2 π ) j , 5 e ( θ +4 π ) j , 5 e ( θ +6 π ) j , 5 e ( θ +8 π ) j z = 5 e ( θ +2 nπ ) j for n = 0 , 1 , 2 , 3 , 4 . Here tan θ = 4 3 with θ lying in the first quadrant (i.e. θ = 0 . 927295 ). The five fifth roots are z 1 / 5 = 5 1 / 5 e ( θ +2 nπ ) j/ 5 for n = 0 , 1 , 2 , 3 , 4 . If we were to plot these in the Complex Plane then we would see that the five points are equally distributed around a circle of radius 5 1 / 5 centred on the origin.

Example 2.11. Find z = (4 + 3 j ) 2 / 3 . This is a 2 / 3 rd power. There are two ways of doing this one. We could square first and then take the cube root, or just take the above approach. First way: Let (4 + 3 j ) 2 � 1 / 3 � = (7 + 24 j ) 1 / 3 . z = In complex exponential form we have 7 + 24 j = 25 e ( θ +2 nπ ) j for n = 0 , 1 , 2 , where tan θ = 24 7 and hence θ = 1 . 287002 (first quadrant). Therefore z = 25 1 / 3 e ( θ +2 nπ ) j/ 3 for n = 0 , 1 , 2 and where θ = 1 . 287002 .

Second way: Let z = (4 + 3 j ) 2 / 3 . In polar form we have 4 + 3 j = 5 e ( φ +2 mπ ) j for m = 0 , 1 , 2 , where tan φ = 3 4 and hence φ = 0 . 643501 . Therefore z = 5 2 / 3 e 2( φ +2 mπ ) j/ 3 for m = 0 , 1 , 2 and where φ = 0 . 643501 .

z = 25 1 / 3 e ( θ +2 nπ ) j/ 3 for n = 0 , 1 , 2 and where θ = 1 . 287002 . z = 5 2 / 3 e 2( φ +2 mπ ) j/ 3 for m = 0 , 1 , 2 and where φ = 0 . 643501 . The following figure shows where the roots are as a consequence of these two analyses. The locations of the roots are identical, but manner in which they are counted is different. Im m = 0 n = 1 n = 0 • m = 2 • 25 1 / 3 Re • n = 2 m = 1 Example 2.11 Arguments: 0 , 2 π/ 3 , 4 π/ 3 . Arguments: 0 , 4 π/ 3 , 8 π/ 3 .

2.8 Relationship with the hyperbolic functions. The functions cos θ and sin θ are known as either trigonometric or circular functions, while cosh θ and sinh θ are the hyperbolic functions. Given that e θj = cos θ + j sin θ e − θj = cos θ − j sin θ, and we may add and subtract these to obtain the relations, � e θj + e − θj � � e θj − e − θj � cos θ = 1 1 and sin θ = . 2 2 j These are reminiscent of � e θ + e − θ � � e θ − e − θ � cosh θ = 1 sinh θ = 1 and , 2 2 and explains why there is so much similarity between the circular and hyperbolic functions, particularly when dealing with calculus and differential equations, even though the circular and the hyperbolic functions look so different from one another.