1
play

1 Linear momentum transfer Angular momentum transfer Linear - PDF document

Jun 11, 2023 •381 likes •480 views

F|N|F spin valves: non-collinear Spin torques and spin pumping I Gerrit Bauer , ESM Part I.2 L sd L Current-induced magnetization reversal Current-induced magnetization reversal Electrical currents can controllably reverse the

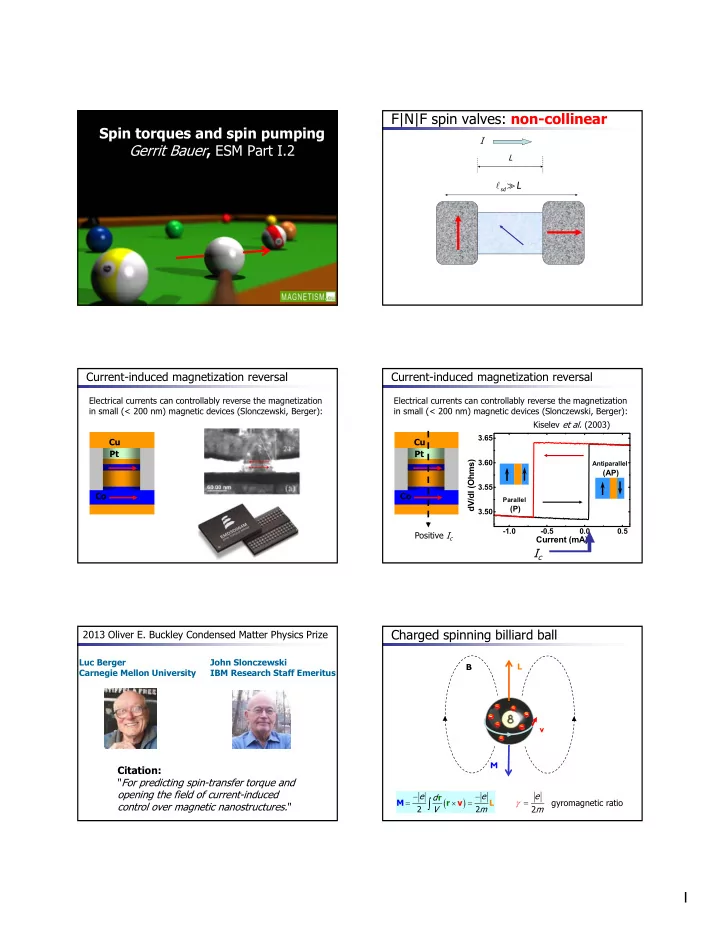
  1. F|N|F spin valves: non-collinear Spin torques and spin pumping I Gerrit Bauer , ESM Part I.2 L sd    L Current-induced magnetization reversal Current-induced magnetization reversal Electrical currents can controllably reverse the magnetization Electrical currents can controllably reverse the magnetization in small (< 200 nm) magnetic devices (Slonczewski, Berger): in small (< 200 nm) magnetic devices (Slonczewski, Berger): Kiselev et al. (2003) 3.65 Cu Cu Pt Pt 3.60 dV/dI (Ohms) Antiparallel (AP) 3.55 Co Co Parallel (P) 3.50 -1.0 -0.5 0.0 0.5 Positive I c Current (mA) I c Charged spinning billiard ball 2013 Oliver E. Buckley Condensed Matter Physics Prize Luc Berger John Slonczewski L B Carnegie Mellon University IBM Research Staff Emeritus v M Citation: " For predicting spin-transfer torque and opening the field of current-induced e   e e   e e d d r r r r       M M     v v   L L   gyromagnetic ratio control over magnetic nanostructures. " 2 2 V V 2 2 m m 2 m 1

  2. Linear momentum transfer Angular momentum transfer Linear momentum transfer Angular momentum transfer Angular momentum transfer 2

  3. Angular momentum transfer Angular momentum pump Angular momentum pump Angular momentum addition Angular momentum addition Angular momentum addition 3

  4. Rotation in quantum mechanics Rotation in quantum mechanics  f  f     ˆ Rotation of a state  by an angle    ˆ R d  f  f x  dx y ,  dy  f  dx  dy   R   z z  x  y n around an axis with unit vector n : R      i    ˆ  f   y  x f d   1  L d  f ˆ   ˆ Rotation operator: R   exp i  n L  /      z  x  y      n    ˆ y R   e i n σ   /2 ˆ Electron spin: L  s  σ n Finite rotation:   lim nd  2 n  n    With n  cos ,sin ,0      z n i   ˆ   ˆ R   lim 1  d L  x n  ( , x y ,0)    1     z z    n        ,  R ,    0     log 1  x  x x   x  dx  x  yd  generates all possible spin states.   n y   y  dy  y  xd   lim log 1  x  lim nx  y Example: n  n  i     1 i i   ˆ        L ˆ ˆ ˆ ˆ n log R   li m nd  L   L  R   e z   R ,     x x z z z z 2 2 0      n  Spins on the Bloch sphere Slonczewski torque in half metals N ormal HMF m 0 1 m    1  0 1      /2              i   /2 / 2 R ,   e  e  1 0      y     m 2 m !  1 0 2       m  0 longitudinal spin current      transverse spin current = torque cos  sin   1 0 2 2          R ,2        /   2 0 1 2        =   / 2 sin cos    2 2   m Spin-up state in x -direction is obtained by rotation about y -axis with   .  1   1  / 2    / 2   x 1 2   m    Ne V        V     2 2         s  x  1,0,0 Check: x 2 N = number of incoming channels Spin-mixing conductance Exchange-field torque e N 2  Precession by spins in evanescent states     ˆ ˆ  m  x  z V  V  =  e h    ˆ x 2 e 2 E 2 e 2 m  F G  1  N ˆ Sharvin y ˆ z h h   k    k incoming wave vectors m ˆ x         m  x ˆ  V  V z ˆ R e G  y ˆ I m G Scattering theory: e      ˆ z e e 2 2 E E e N e N 2 2 Spin mixing conductance:       * *     F F G G   1 1   r r   r r            k k   k k   h h     h h     k k 4

  5. Switching by spin transfer Switching by spin transfer Ohm’s and Kirchhoff’s Laws Spin currents N spin accumulation in N V I I V N I c V F s c ,3  2 s spin accumulation in F  c ,1  2 m s V 1 V 2 V 3 V F magnetization direction s N F  V charge conservation I , I , I transverse c s ,  s ,   spin currents I   0 c j , i collinear/longitudinal j Charge current:     I  2Re G m  V N  m  2Im G V N  m   conductance I  G V  V G  s ,  s s   c , 1  2 1  2 1 2 1 2 in-plane out-of-plane     I (damping) (effective field) s ,  2 e e e 2 2 e e 2 2   2 2     G G g g t t Landauer formula nm nm h h h h nm nm Pauli matrix notation Spin currents    1 0 0 1 0  i 1 0          spin accumulation in N V N ˆ ˆ 1, σ  , , , V N           F s V s spin accumulation in F  0 1 1 0 i 0 0  1           m s F V magnetization direction s  X X  N F ˆ ˆ X     X 1  X σ ˆ   * c s X X     I , I , I G G   transverse ˆ 0 s ,  s ,  G     ˆ ˆ  V N  V N 1  V N  σ ˆ spin currents G * G   c s   collinear/longitudinal ˆ ˆ V F  V F 1  V F m σ  ˆ c s s=  ,  spin-dependent Landauer   2 2   2 2 ˆ ˆ g g   t t s s   N N   r r s s I  I 1  I  σ ˆ conductances for charge and s s nm nm nm nm c s ˆ N nm nm nm nm V ˆ F collinear spin current V         complex spin-mixing conductance * * ˆ g g     N N r r   r r   I N F nm nm nm nm for transverse spin current nm nm (torque + exchange field) 5

  6. Angular magnetoresistance Circuit theory (Brataas et al ., 2000)  Py Re G   0.5 10  15   1 m  2 ˆ ˆ (12 nm) I  I  1 2 3 2 Cu Py (6 nm) AF AF m m s 1 3 2 Py (1.5 nm) Cu ˆ ˆ ˆ ˆ G G G G Py 1 2 3 4 ˆ ˆ ˆ AF  V V V V 0 1 2 3 ˆ ˆ V  V 1  V m  σ ˆ spin & charge conservation: 1 c ,1 s ,1 1 ˆ ˆ ˆ ˆ ˆ V  V 1  V s  σ I  I  0 Exp: S. Urazhdin et al . (2005) 2 c ,2 s ,2 2 1  2 3  2 Contents Spin accumulation-driven magnetization reversal V Spin pumping Kimura et al . (2005) Spin vs. charge pumping Spin torque and spin pumping Pumping (Büttiker, Brouwer): Current flows without applied bias, but due to time-dependent modulation of Onsager reciprocals (Brataas et al ., 2011) scattering matrix. Spin currents cause magnetization motion Magnetization motion causes spin currents (spin transfer torque, Slonczewski, 1996). (spin pumping, Tserkovnyak, 2002). charge and mass pumping LLG equation spin transfer torque H J s   M   L L  H   M  mm ms      J L L V      sm ss s s spin pumping   spin pumping spin conductance     e V s s       L Μ  L T  Μ g  spin mixing conductance  sm ms 6

Recommend

More recommend